Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-29T13:32:12.735Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent plane Couette flow at moderately high Reynolds number

Published online by Cambridge University Press:  17 June 2014

V. Avsarkisov ,
S. Hoyas ,
M. Oberlack  and
J. P. García-Galache
V. Avsarkisov
Affiliation:
Chair of Fluid Dynamics, TU Darmstadt, Otto-Berndt-Strasse 2, 64287 Darmstadt, Germany
S. Hoyas*
Affiliation:
CMT Motores Térmicos, Universitat Politècnica de València, València, Spain
M. Oberlack
Affiliation:
Chair of Fluid Dynamics, TU Darmstadt, Otto-Berndt-Strasse 2, 64287 Darmstadt, Germany Center of Smart Interfaces, TU Darmstadt, Alarich-Weiss-Strasse 10, 64287 Darmstadt, Germany GS Computational Engineering, TU Darmstadt, Dolivostrasse 15, 64293 Darmstadt, Germany
J. P. García-Galache
Affiliation:
CMT Motores Térmicos, Universitat Politècnica de València, València, Spain
*
†Email address for correspondence: serhocal@mot.upv.es
Get access Rights & Permissions [Opens in a new window]

Abstract

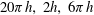A new set of numerical simulations of turbulent plane Couette flow in a large box of dimension ($\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}20\pi h,\, 2h,\, 6\pi h$) at Reynolds number 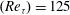$(\mathit{Re}_{\tau }) =125$, 180, 250 and 550 is described and compared with simulations at lower Reynolds numbers, Poiseuille flows and experiments. The simulations present a logarithmic near-wall layer and are used to verify and revise previously known results. It is confirmed that the fluctuation intensities in the streamwise and spanwise directions do not scale well in wall units. The scaling failure occurs both near to and away from the wall. On the contrary, the wall-normal intensity scales in inner units in the near-wall region and in outer units in the core region. The spectral ridge found by Hoyas & Jiménez (Phys. Fluids, vol. 18, 2003, 011702) for the turbulent Poiseuille flow can also be seen in the present flow. Away from the wall, very large-scale motions are found spanning through all the length of the channel. The statistics of these simulations can be downloaded from the webpage of the Chair of Fluid Dynamics.

JFM classification

Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulence theory Turbulent Flows: Turbulent Flows
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 751 , 25 July 2014 , R1
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

del Álamo, J. C. & Jiménez, J. 2003 Spectra of the very large anisotropic scales in turbulent channels. Phys. Fluids 15 (6), L41–L44.Google Scholar
Bech, K., Tillmark, N., Alfredsson, P. & Andersson, H. 1995 An investigation of turbulent plane Couette flow at low Reynolds numbers. J. Fluid Mech. 286, 291–325.Google Scholar
Bernardini, M., Pirozzoli, S. & Orlandi, P. 2013 The effect of large-scale turbulent structures on particle dispersion in wall-bounded flows. Intl J. Multiphase Flow 51, 55–64.Google Scholar
Busse, F. H. 1970 Bounds for turbulent shear flow. J. Fluid Mech. 41, 219–240.Google Scholar
Hamilton, J. M., Kim, J. & Waleffe, F. 1995 Regeneration mechanisms of near-wall turbulence structures. J. Fluid Mech. 287, 317–348.Google Scholar
Hoyas, S. & Jiménez, J. 2006 Scaling of the velocity fluctuations in turbulent channels up to ${\mathit{Re}}_{\tau }=2003$ . Phys. Fluids 18 (1), 011702.Google Scholar
Hoyas, S. & Jiménez, J. 2008 Reynolds number effects on the Reynolds-stress budgets in turbulent channels. Phys. Fluids 20 (10), 101511.CrossRefGoogle Scholar
Jiménez, J. 2013 Near-wall turbulence. Phys. Fluids 25 (10), 101302.CrossRefGoogle Scholar
Jiménez, J. & Hoyas, S. 2008 Turbulent fluctuations above the buffer layer of wall-bounded flows. J. Fluid Mech. 611, 215–236.CrossRefGoogle Scholar
Kim, J., Moin, P. & Moser, R. 1987 Turbulence statistics in fully developed channel flows at low Reynolds numbers. J. Fluid Mech. 320, 259–285.Google Scholar
Kitoh, O., Nakabyashi, K. & Nishimura, F. 2005 Experimental study on mean velocity and turbulence characteristics of plane Couette flow: low-Reynolds-number effects and large longitudinal vortical structure. J. Fluid Mech. 539, 199–227.Google Scholar
Kitoh, O. & Umeki, M. 2008 Experimental study on large-scale streak structure in the core region of turbulent plane Couette flow. Phys. Fluids 20 (2), 025107.Google Scholar
Komminaho, J., Lundbladh, A. & Johansson, A. 1996 Very large structures in plane turbulent Couette flow. J. Fluid Mech. 320, 258–259.Google Scholar
Lele, S. K. 1992 Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103 (1), 16–42.CrossRefGoogle Scholar
Lund, K. O. & Bush, W. B. 1980 Asymptotic analysis of plane turbulent Couette–Poiseuille flows. J. Fluid Mech. 96, 81–104.CrossRefGoogle Scholar
Mansour, N. N., Kim, J. & Moin, P. 1988 Reynolds-stress and dissipation-rate budgets in a turbulent channel flow. J. Fluid Mech. 194, 15–44.Google Scholar
Melnikov, K., Kreilos, T. & Eckhardt, B. 2014 Long-wavelength instability of coherent structures in plane Couette flow. Phys. Rev. E 89, 043008-1–8.CrossRefGoogle ScholarPubMed
Moser, R. D., Kim, J. & Mansour, N. N. 1999 Direct numerical simulation of turbulent channel flow up to ${\mathit{Re}}_{\tau }=590$ . Phys. Fluids 11 (4), 943–945.Google Scholar
Pirozzoli, S., Bernardini, M. & Orlandi, P. 2011 Large-scale motions and inner/outer layer interactions in turbulent Couette–Poiseuille flows. J. Fluid Mech. 680, 534–563.CrossRefGoogle Scholar
Reichardt, H. 1959 Gezetzmässigkeiten der geradlinigen turbulenten Couetteströmung, Mitteilungen aus dem Max-Planck-Institut für Strömungsforschung Göttingen, vol. 22.Google Scholar
Spalart, P. R. 1991 Spectral methods for the Navier–Stokes equations with one infinite and two periodic directions. J. Comput. Phys. 96 (2), 297–324.Google Scholar
Tillmark, N.1995 Experiments on transition and turbulence in plane Couette flow. PhD thesis, KTH, Royal Institute of Technology.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flows, 2nd edn. Cambridge University Press.Google Scholar
Tsukahara, T., Kawamura, H. & Shingai, K. 2006 DNS of turbulent Couette flow with emphasis on the large-scale structure in the core region. J. Turbul. 7, 1–16.Google Scholar