Article contents
Vortex-induced vibration of a transversely rotating sphere
Published online by Cambridge University Press: 29 May 2018
Abstract
The effects of transverse rotation on the vortex-induced vibration (VIV) of a sphere in a uniform flow are investigated numerically. The one degree-of-freedom sphere motion is constrained to the cross-stream direction, with the rotation axis orthogonal to flow and vibration directions. For the current simulations, the Reynolds number of the flow, 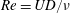 $Re=UD/\unicode[STIX]{x1D708}$ , and the mass ratio of the sphere,
$Re=UD/\unicode[STIX]{x1D708}$ , and the mass ratio of the sphere, 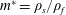 $m^{\ast }=\unicode[STIX]{x1D70C}_{s}/\unicode[STIX]{x1D70C}_{f}$ , were fixed at 300 and 2.865, respectively, while the reduced velocity of the flow was varied over the range
$m^{\ast }=\unicode[STIX]{x1D70C}_{s}/\unicode[STIX]{x1D70C}_{f}$ , were fixed at 300 and 2.865, respectively, while the reduced velocity of the flow was varied over the range 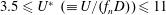 $3.5\leqslant U^{\ast }~(\equiv U/(f_{n}D))\leqslant 11$ , where,
$3.5\leqslant U^{\ast }~(\equiv U/(f_{n}D))\leqslant 11$ , where, 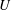 $U$ is the upstream velocity of the flow,
$U$ is the upstream velocity of the flow, 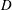 $D$ is the sphere diameter,
$D$ is the sphere diameter,  $\unicode[STIX]{x1D708}$ is the fluid viscosity,
$\unicode[STIX]{x1D708}$ is the fluid viscosity, 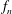 $f_{n}$ is the system natural frequency and
$f_{n}$ is the system natural frequency and 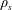 $\unicode[STIX]{x1D70C}_{s}$ and
$\unicode[STIX]{x1D70C}_{s}$ and 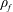 $\unicode[STIX]{x1D70C}_{f}$ are solid and fluid densities, respectively. The effect of sphere rotation on VIV was studied over a wide range of non-dimensional rotation rates:
$\unicode[STIX]{x1D70C}_{f}$ are solid and fluid densities, respectively. The effect of sphere rotation on VIV was studied over a wide range of non-dimensional rotation rates: 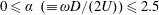 $0\leqslant \unicode[STIX]{x1D6FC}~(\equiv \unicode[STIX]{x1D714}D/(2U))\leqslant 2.5$ , with
$0\leqslant \unicode[STIX]{x1D6FC}~(\equiv \unicode[STIX]{x1D714}D/(2U))\leqslant 2.5$ , with 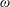 $\unicode[STIX]{x1D714}$ the angular velocity. The flow satisfied the incompressible Navier–Stokes equations while the coupled sphere motion was modelled by a spring–mass–damper system, under zero damping. For zero rotation, the sphere oscillated symmetrically through its initial position with a maximum amplitude of approximately 0.4 diameters. Under forced rotation, it oscillated about a new time-mean position. Rotation also resulted in a decreased oscillation amplitude and a narrowed synchronisation range. VIV was suppressed completely for
$\unicode[STIX]{x1D714}$ the angular velocity. The flow satisfied the incompressible Navier–Stokes equations while the coupled sphere motion was modelled by a spring–mass–damper system, under zero damping. For zero rotation, the sphere oscillated symmetrically through its initial position with a maximum amplitude of approximately 0.4 diameters. Under forced rotation, it oscillated about a new time-mean position. Rotation also resulted in a decreased oscillation amplitude and a narrowed synchronisation range. VIV was suppressed completely for  $\unicode[STIX]{x1D6FC}>1.3$ . Within the
$\unicode[STIX]{x1D6FC}>1.3$ . Within the  $U^{\ast }$ synchronisation range for each rotation rate, the drag force coefficient increased while the lift force coefficient decreased from their respective pre-oscillatory values. The increment of the drag force coefficient and the decrement of the lift force coefficient reduced with increasing reduced velocity as well as with increasing rotation rate. In terms of wake dynamics, in the synchronisation range at zero rotation, two equal-strength trails of interlaced hairpin-type vortex loops were formed behind the sphere. Under rotation, the streamwise vorticity trail on the advancing side of the sphere became stronger than the trail in the retreating side, consistent with wake deflection due to the Magnus effect. This symmetry breaking appears to be associated with the reduction in the observed amplitude response and the narrowing of the synchronisation range. In terms of variation with Reynolds number, the sphere oscillation amplitude was found to increase over the range
$U^{\ast }$ synchronisation range for each rotation rate, the drag force coefficient increased while the lift force coefficient decreased from their respective pre-oscillatory values. The increment of the drag force coefficient and the decrement of the lift force coefficient reduced with increasing reduced velocity as well as with increasing rotation rate. In terms of wake dynamics, in the synchronisation range at zero rotation, two equal-strength trails of interlaced hairpin-type vortex loops were formed behind the sphere. Under rotation, the streamwise vorticity trail on the advancing side of the sphere became stronger than the trail in the retreating side, consistent with wake deflection due to the Magnus effect. This symmetry breaking appears to be associated with the reduction in the observed amplitude response and the narrowing of the synchronisation range. In terms of variation with Reynolds number, the sphere oscillation amplitude was found to increase over the range 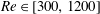 $Re\in [300,1200]$ at
$Re\in [300,1200]$ at  $U^{\ast }=6$ for each of
$U^{\ast }=6$ for each of 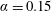 $\unicode[STIX]{x1D6FC}=0.15$ , 0.75 and 1.5. The VIV response depends strongly on Reynolds number, with predictions indicating that VIV will persist for higher rotation rates at higher Reynolds numbers.
$\unicode[STIX]{x1D6FC}=0.15$ , 0.75 and 1.5. The VIV response depends strongly on Reynolds number, with predictions indicating that VIV will persist for higher rotation rates at higher Reynolds numbers.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 26
- Cited by


