Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Xue, S.
Thorne, R. M.
and
Summers, D.
1993.
Electromagnetic ion‐cyclotron instability in space plasmas.
Journal of Geophysical Research: Space Physics,
Vol. 98,
Issue. A10,
p.
17475.
Summers, Danny
Xue, Song
and
Thorne, Richard M.
1994.
Calculation of the dielectric tensor for a generalized Lorentzian (kappa) distribution function.
Physics of Plasmas,
Vol. 1,
Issue. 6,
p.
2012.
Summers, Danny
and
Thorne, Richard M.
1995.
Plasma microinstabilities driven by loss-cone distributions.
Journal of Plasma Physics,
Vol. 53,
Issue. 3,
p.
293.
Mace, R. L.
and
Hellberg, M. A.
1995.
A dispersion function for plasmas containing superthermal particles.
Physics of Plasmas,
Vol. 2,
Issue. 6,
p.
2098.
Summers, Danny
Thorne, Richard M.
and
Matsumoto, Hiroshi
1996.
Evaluation of the modified plasma dispersion function for half-integral indices.
Physics of Plasmas,
Vol. 3,
Issue. 7,
p.
2496.
Mace, R. L.
1996.
A dielectric tensor for a uniform magnetoplasma with a generalized Lorentzian distribution.
Journal of Plasma Physics,
Vol. 55,
Issue. 3,
p.
415.
Mace, R L
1996.
A dielectric tensor for magnetoplasmas comprising components with generalized lorentzian distributions.
Physica Scripta,
Vol. T63,
Issue. ,
p.
207.
Xue, Song
Thorne, Richard M.
and
Summers, Danny
1996.
Parametric study of electromagnetic ion cyclotron instability in the Earth's magnetosphere.
Journal of Geophysical Research: Space Physics,
Vol. 101,
Issue. A7,
p.
15467.
Yoon, Jung-Sik
and
Jung, Young-Dae
1998.
Dynamic orientation phenomena for direct 1s→2p±1 electron–ion excitations in a generalized Lorentzian (kappa) distribution plasma.
Physics of Plasmas,
Vol. 5,
Issue. 12,
p.
4461.
Yoon, Jung-Sik
and
Jung, Young-Dae
1998.
Dynamic plasma screening effects on electron–ion collisional excitations in a generalized Lorenzian (kappa) distribution plasma.
Physics of Plasmas,
Vol. 5,
Issue. 7,
p.
2480.
Kim, Chang-Geun
and
Jung, Young-Dae
2000.
Dynamic plasma screening effects on electron–ion collisional ionizations in a generalized Lorentzian distribution plasma.
Physics of Plasmas,
Vol. 7,
Issue. 10,
p.
4303.
Gunell, H.
and
Skiff, F.
2001.
Weakly damped acoustic-like ion waves in plasmas with non-Maxwellian ion distributions.
Physics of Plasmas,
Vol. 8,
Issue. 8,
p.
3550.
Hellberg, M. A.
and
Mace, R. L.
2002.
Generalized plasma dispersion function for a plasma with a kappa-Maxwellian velocity distribution.
Physics of Plasmas,
Vol. 9,
Issue. 5,
p.
1495.
Petkaki, P.
Watt, C. E. J.
Horne, R. B.
and
Freeman, M. P.
2003.
Anomalous resistivity in non‐Maxwellian plasmas.
Journal of Geophysical Research: Space Physics,
Vol. 108,
Issue. A12,
Mace, R. L.
2003.
A Gordeyev integral for electrostatic waves in a magnetized plasma with a kappa velocity distribution.
Physics of Plasmas,
Vol. 10,
Issue. 6,
p.
2181.
Jung, Young-Dae
2003.
Nonthermal effects on occurrence scattering time in generalized Lorentzian distribution plasmas.
Physics of Plasmas,
Vol. 10,
Issue. 5,
p.
1215.
Podesta, J. J.
2005.
Spatial Landau damping in plasmas with three-dimensional κ distributions.
Physics of Plasmas,
Vol. 12,
Issue. 5,
Hellberg, Manfred
Mace, Richard
and
Cattaert, Tom
2005.
Effects of Superthermal Particles on Waves in Magnetized Space Plasmas.
Space Science Reviews,
Vol. 121,
Issue. 1-4,
p.
127.
Büchner, Jörg
and
Elkina, Nina
2005.
Vlasov Code Simulation of Anomalous Resistivity.
Space Science Reviews,
Vol. 121,
Issue. 1-4,
p.
237.
Büchner, Jörg
and
Elkina, Nina
2006.
Anomalous resistivity of current-driven isothermal plasmas due to phase space structuring.
Physics of Plasmas,
Vol. 13,
Issue. 8,
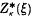 is employed to study the instability of ion-acoustic waves driven by electron drift in a hot isotropic unmagnetized plasma modelled by a kappa distribution. The real and imaginary parts of the wave frequency ω0 + ιγ are obtained as functions of the normalized wavenumber kλD, where λD is the electron Debye length. Marginal stability conditions for instability are obtained for different ion-to-electron temperature ratios. The results for a kappa distribution are compared with the classical results for a Maxwellian. In all cases studied the ion-acoustic waves are strongly damped at short wavelengths, kλD ≫ 1, but they can be destabilized at long wavelengths. The instability for both the kappa and Maxwellian distributions can be quenched by increasing the ion-electron temperature ratio Ti/Te. However, both the marginally unstable electron drift velocities and the growth rates of unstable waves can differ significantly between a generalized Lorentzian and a Maxwellian plasma; these differences are also influenced by the value of Ti/Te.
is employed to study the instability of ion-acoustic waves driven by electron drift in a hot isotropic unmagnetized plasma modelled by a kappa distribution. The real and imaginary parts of the wave frequency ω0 + ιγ are obtained as functions of the normalized wavenumber kλD, where λD is the electron Debye length. Marginal stability conditions for instability are obtained for different ion-to-electron temperature ratios. The results for a kappa distribution are compared with the classical results for a Maxwellian. In all cases studied the ion-acoustic waves are strongly damped at short wavelengths, kλD ≫ 1, but they can be destabilized at long wavelengths. The instability for both the kappa and Maxwellian distributions can be quenched by increasing the ion-electron temperature ratio Ti/Te. However, both the marginally unstable electron drift velocities and the growth rates of unstable waves can differ significantly between a generalized Lorentzian and a Maxwellian plasma; these differences are also influenced by the value of Ti/Te.