Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Caprioli, Damiano
Haggerty, Colby C.
and
Blasi, Pasquale
2020.
Kinetic Simulations of Cosmic-Ray-modified Shocks. II. Particle Spectra.
The Astrophysical Journal,
Vol. 905,
Issue. 1,
p.
2.
Zhdankin, Vladimir
2021.
Particle Energization in Relativistic Plasma Turbulence: Solenoidal versus Compressive Driving.
The Astrophysical Journal,
Vol. 922,
Issue. 2,
p.
172.
Drake, J. F.
Pfrommer, C.
Reynolds, C. S.
Ruszkowski, M.
Swisdak, M.
Einarsson, A.
Thomas, T.
Hassam, A. B.
and
Roberg-Clark, G. T.
2021.
Whistler-regulated Magnetohydrodynamics: Transport Equations for Electron Thermal Conduction in the High-β Intracluster Medium of Galaxy Clusters.
The Astrophysical Journal,
Vol. 923,
Issue. 2,
p.
245.
Bott, A. F. A.
Arzamasskiy, L.
Kunz, M. W.
Quataert, E.
and
Squire, J.
2021.
Adaptive Critical Balance and Firehose Instability in an Expanding, Turbulent, Collisionless Plasma.
The Astrophysical Journal Letters,
Vol. 922,
Issue. 2,
p.
L35.
Parashar, Tulasi N.
and
Matthaeus, William H.
2022.
Observations of cross scale energy transfer in the inner heliosphere by Parker Solar Probe.
Reviews of Modern Plasma Physics,
Vol. 6,
Issue. 1,
Choudhury, Prakriti Pal
and
Reynolds, Christopher S
2022.
Acoustic waves and g-mode turbulence as energy carriers in a viscous intracluster medium.
Monthly Notices of the Royal Astronomical Society,
Vol. 514,
Issue. 3,
p.
3765.
Schekochihin, Alexander A.
2022.
MHD turbulence: a biased review.
Journal of Plasma Physics,
Vol. 88,
Issue. 5,
Coburn, Jesse T.
Chen, Christopher H.K.
and
Squire, Jonathan
2022.
A measurement of the effective mean free path of solar wind protons.
Journal of Plasma Physics,
Vol. 88,
Issue. 5,
Hlavacek-Larrondo, Julie
Li, Yuan
and
Churazov, Eugene
2022.
Handbook of X-ray and Gamma-ray Astrophysics.
p.
1.
Tran, Aaron
Sironi, Lorenzo
Ley, Francisco
Zweibel, Ellen G.
and
Riquelme, Mario A.
2023.
Electron Reacceleration via Ion Cyclotron Waves in the Intracluster Medium.
The Astrophysical Journal,
Vol. 948,
Issue. 2,
p.
130.
Squire, J.
Kunz, M.W.
Arzamasskiy, L.
Johnston, Z.
Quataert, E.
and
Schekochihin, A.A.
2023.
Pressure anisotropy and viscous heating in weakly collisional plasma turbulence.
Journal of Plasma Physics,
Vol. 89,
Issue. 4,
Majeski, S.
Kunz, M.W.
and
Squire, J.
2023.
Microphysically modified magnetosonic modes in collisionless, high-β plasmas.
Journal of Plasma Physics,
Vol. 89,
Issue. 3,
Arzamasskiy, Lev
Kunz, Matthew W.
Squire, Jonathan
Quataert, Eliot
and
Schekochihin, Alexander A.
2023.
Kinetic Turbulence in Collisionless High-
β
Plasmas.
Physical Review X,
Vol. 13,
Issue. 2,
Majeski, S.
Kunz, M.W.
and
Squire, J.
2024.
Self-organization in collisionless, high-β turbulence.
Journal of Plasma Physics,
Vol. 90,
Issue. 6,
Hlavacek-Larrondo, Julie
Li, Yuan
and
Churazov, Eugene
2024.
Handbook of X-ray and Gamma-ray Astrophysics.
p.
4895.
Majeski, S.
and
Kunz, M.W.
2024.
On hydromagnetic wave interactions in collisionless, high-βplasmas.
Journal of Plasma Physics,
Vol. 90,
Issue. 1,
Du, Senbei
Opher, Merav
Giacalone, Joe
Guo, Fan
Richardson, John D.
and
Zieger, Bertalan
2024.
The Effects of Turbulence on Heliosheath Ions and Implications for Energetic Neutral Atoms.
The Astrophysical Journal,
Vol. 974,
Issue. 2,
p.
210.
Bott, Archie F.A.
Cowley, S.C.
and
Schekochihin, A.A.
2024.
Kinetic stability of Chapman–Enskog plasmas.
Journal of Plasma Physics,
Vol. 90,
Issue. 2,
Ioannou, C.
Nicolaou, G.
Owen, C. J.
Verscharen, D.
Coburn, J. T.
and
Franci, L.
2025.
Polytropic Analysis of Large-scale Compressive Fluctuations in the Solar Wind: Fluid and Kinetic Behavior.
The Astrophysical Journal,
Vol. 988,
Issue. 2,
p.
253.
Bott, Archie F. A.
Kunz, Matthew W.
Quataert, Eliot
Squire, Jonathan
and
Arzamasskiy, Lev
2025.
Thermodynamics and collisionality in firehose-susceptible high-
$\beta$
plasmas.
Journal of Plasma Physics,
Vol. 91,
Issue. 5,
 $\delta n/n_0 \gtrsim 2/\beta$ (where
$\delta n/n_0 \gtrsim 2/\beta$ (where 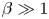 $\beta \gg {1}$ is the ratio of thermal to magnetic pressure) generate sufficient pressure anisotropy to destabilize the plasma to firehose and mirror instabilities. These kinetic instabilities grow rapidly to reduce the pressure anisotropy by pitch-angle scattering and trapping particles, respectively, thereby impeding the maintenance of Landau resonances that enable such waves’ otherwise potent collisionless damping. The result is wave dynamics that evince a weakly collisional plasma: the ion distribution function is near-Maxwellian, the field-parallel flow of heat resembles its Braginskii form (except in regions where large-amplitude magnetic mirrors strongly suppress particle transport), and the relations between various thermodynamic quantities are more ‘fluid-like’ than kinetic. A nonlinear fluctuation–dissipation relation for self-sustaining IAWs is obtained by solving a plasma-kinetic Langevin problem, which demonstrates suppressed damping, enhanced fluctuation levels and weakly collisional thermodynamics when IAWs with
$\beta \gg {1}$ is the ratio of thermal to magnetic pressure) generate sufficient pressure anisotropy to destabilize the plasma to firehose and mirror instabilities. These kinetic instabilities grow rapidly to reduce the pressure anisotropy by pitch-angle scattering and trapping particles, respectively, thereby impeding the maintenance of Landau resonances that enable such waves’ otherwise potent collisionless damping. The result is wave dynamics that evince a weakly collisional plasma: the ion distribution function is near-Maxwellian, the field-parallel flow of heat resembles its Braginskii form (except in regions where large-amplitude magnetic mirrors strongly suppress particle transport), and the relations between various thermodynamic quantities are more ‘fluid-like’ than kinetic. A nonlinear fluctuation–dissipation relation for self-sustaining IAWs is obtained by solving a plasma-kinetic Langevin problem, which demonstrates suppressed damping, enhanced fluctuation levels and weakly collisional thermodynamics when IAWs with 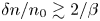 $\delta n/n_0 \gtrsim 2/\beta$ are stochastically driven. We investigate how our results depend upon the scale separation between the wavelength of the IAW and the Larmor radius of the ions, and discuss briefly their implications for our understanding of turbulence and transport in the solar wind and the intracluster medium of galaxy clusters.
$\delta n/n_0 \gtrsim 2/\beta$ are stochastically driven. We investigate how our results depend upon the scale separation between the wavelength of the IAW and the Larmor radius of the ions, and discuss briefly their implications for our understanding of turbulence and transport in the solar wind and the intracluster medium of galaxy clusters.

