Published online by Cambridge University Press: 23 August 2022
In this paper, we study the boundary blow-up problem related to the infinity Laplacian
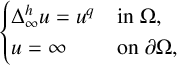 $$ \begin{align*}\begin{cases} \Delta_{\infty}^h u=u^q &\mathrm{in}\; \Omega, \\ u=\infty &\mathrm{on} \;\partial\Omega, \end{cases} \end{align*} $$
$$ \begin{align*}\begin{cases} \Delta_{\infty}^h u=u^q &\mathrm{in}\; \Omega, \\ u=\infty &\mathrm{on} \;\partial\Omega, \end{cases} \end{align*} $$
where
 $\Delta _{\infty }^h u=|Du|^{h-3} \langle D^2uDu,Du \rangle $
is the highly degenerate and h-homogeneous operator associated with the infinity Laplacian arising from the stochastic game named Tug-of-War. When
$\Delta _{\infty }^h u=|Du|^{h-3} \langle D^2uDu,Du \rangle $
is the highly degenerate and h-homogeneous operator associated with the infinity Laplacian arising from the stochastic game named Tug-of-War. When
 $q>h>1$
, we establish the existence of the boundary blow-up viscosity solution. Moreover, when the domain satisfies some regular condition, we establish the asymptotic estimate of the blow-up solution near the boundary. As an application of the asymptotic estimate and the comparison principle, we obtain the uniqueness result of the large solution. We also give the nonexistence of the large solution for the case
$q>h>1$
, we establish the existence of the boundary blow-up viscosity solution. Moreover, when the domain satisfies some regular condition, we establish the asymptotic estimate of the blow-up solution near the boundary. As an application of the asymptotic estimate and the comparison principle, we obtain the uniqueness result of the large solution. We also give the nonexistence of the large solution for the case
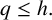 $q \leq h.$
$q \leq h.$
Communicated by Florica Cirstea
This work was supported by National Natural Science Foundation of China (Nos. 11501292, 11871275 and 12141104).