Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Khukhro, E. I.
2014.
Nonsoluble Length of Finite Groups.
Algebra and Logic,
Vol. 53,
Issue. 5,
p.
425.
CONTRERAS–ROJAS, Y.
and
SHUMYATSKY, PAVEL
2015.
Nonsoluble length of finite groups with commutators of small order.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 158,
Issue. 3,
p.
487.
Khukhro, E. I.
2015.
On finite soluble groups with almost fixed-point-free automorphisms of noncoprime order.
Siberian Mathematical Journal,
Vol. 56,
Issue. 3,
p.
541.
Хухро, Е. И.
and
Шумяцкий, П.
2017.
ПАРАМЕТРЫ ТИПА ДЛИНЫ КОНЕЧНЫХ ГРУПП С ПОЧТИ УНИПОТЕНТНЫМИ АВТОМОРФИЗМАМИ, "Доклады Академии наук".
Доклады Академии наук,
p.
265.
Khukhro, E. I.
and
Shumyatsky, P.
2017.
Length-type parameters of finite groups with almost unipotent automorphisms.
Doklady Mathematics,
Vol. 95,
Issue. 1,
p.
43.
Contreras-Rojas, Y.
and
Shumyatsky, P.
2017.
Nonsoluble length of finite groups with restrictions on Sylow subgroups.
Communications in Algebra,
Vol. 45,
Issue. 8,
p.
3606.
Khukhro, E. I.
and
Shumyatsky, P.
2017.
Engel-type subgroups and length parameters of finite groups.
Israel Journal of Mathematics,
Vol. 222,
Issue. 2,
p.
599.
Fumagalli, Francesco
Leinen, Felix
and
Puglisi, Orazio
2019.
A reduction theorem for nonsolvable finite groups.
Israel Journal of Mathematics,
Vol. 232,
Issue. 1,
p.
231.
Detomi, Eloisa
Morigi, Marta
and
Shumyatsky, Pavel
2020.
Profinite groups with restricted centralizers of commutators.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 150,
Issue. 5,
p.
2301.
KHUKHRO, E. I.
SHUMYATSKY, P.
and
TRAUSTASON, G.
2020.
RIGHT ENGEL-TYPE SUBGROUPS AND LENGTH PARAMETERS OF FINITE GROUPS.
Journal of the Australian Mathematical Society,
Vol. 109,
Issue. 3,
p.
340.
Khukhro, E. I.
and
Shumyatsky, P.
2021.
Compact groups in which all elements have countable right Engel sinks.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 151,
Issue. 6,
p.
1790.
Khukhro, E. I.
and
Shumyatsky, P.
2021.
Compact groups with countable Engel sinks.
Bulletin of Mathematical Sciences,
Vol. 11,
Issue. 03,
Khukhro, E. I.
and
Shumyatsky, P.
2023.
Strong conciseness of Engel words in profinite groups.
Mathematische Nachrichten,
Vol. 296,
Issue. 6,
p.
2404.
Azevedo, João
and
Shumyatsky, Pavel
2023.
Compact groups with high commuting probability of monothetic subgroups.
Journal of Algebra,
Vol. 623,
Issue. ,
p.
34.
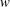 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}w$ be a multilinear commutator word, that is, a commutator of weight
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}w$ be a multilinear commutator word, that is, a commutator of weight  $n$ in
$n$ in  $n$ different group variables. It is proved that if
$n$ different group variables. It is proved that if 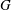 $G$ is a profinite group in which all pronilpotent subgroups generated by
$G$ is a profinite group in which all pronilpotent subgroups generated by  $w$-values are periodic, then the verbal subgroup
$w$-values are periodic, then the verbal subgroup 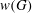 $w(G)$ is locally finite.
$w(G)$ is locally finite.