Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-10T16:51:27.208Z Has data issue: false hasContentIssue false
  • English
  • Français

LOCAL DECAY FOR THE DAMPED WAVE EQUATION IN THE ENERGY SPACE

Part of: Hyperbolic equations and systems Special classes of linear operators Partial differential equations General theory of linear operators

Published online by Cambridge University Press:  01 April 2016

Julien Royer
Julien Royer*
Affiliation:
Institut de Mathématiques de Toulouse, 118 route de Narbonne, 31062 Toulouse Cédex 9, France (julien.royer@math.univ-toulouse.fr)
Get access Rights & Permissions [Opens in a new window]

Abstract

We improve a previous result about the local energy decay for the damped wave equation on 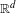$\mathbb{R}^{d}$. The problem is governed by a Laplacian associated with a long-range perturbation of the flat metric and a short-range absorption index. Our purpose is to recover the decay 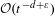${\mathcal{O}}(t^{-d+\unicode[STIX]{x1D700}})$ in the weighted energy spaces. The proof is based on uniform resolvent estimates, given by an improved version of the dissipative Mourre theory. In particular, we have to prove the limiting absorption principle for the powers of the resolvent with inserted weights.

Keywords

local energy decaydamped wave equationMourre’s commutators methodnon-self-adjoint operatorsresolvent estimates

MSC classification

Primary: 35B40: Asymptotic behavior of solutions 35L05: Wave equation 47A40: Scattering theory 47A55: Perturbation theory 47B44: Accretive operators, dissipative operators, etc.
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 17 , Issue 3 , June 2018 , pp. 509 - 540
Copyright
© Cambridge University Press 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aloui, L. and Khenissi, M., Stabilisation pour l’équation des ondes dans un domaine extérieur, Rev. Mat. Iberoam. 18 (2002), 1–16.CrossRefGoogle Scholar
Amrein, W. O., de Monvel, B. and Georgescu, V., C 0 -groups, Commutator Methods and Spectral Theory of N-Body Hamiltonians, Progress in Mathematics, vol. 135 (Birkhäuser, Basel, 1996).Google Scholar
Bardos, C., Lebeau, G. and Rauch, J., Sharp sufficient conditions for the observation, control, and stabilization of waves from the boundary, SIAM J. Control Optim. 30(5) (1992), 1024–1065.Google Scholar
Bony, J.-F. and Häfner, D., Local energy decay for several evolution equations on asymptotically Euclidean manifolds, Ann. Sci. Éc. Norm. Supér. (4) 45(2) (2012), 311–335.Google Scholar
Bouclet, J.-M., Low frequency estimates and local energy decay for asymptotically Euclidean laplacians, Comm. Partial Differential Equations 36 (2011), 1239–1286.Google Scholar
Bouclet, J.-M. and Royer, J., Local energy decay for the damped wave equation, J. Funct. Anal. 266(2) (2014), 4538–4615.Google Scholar
Bouclet, J.-M. and Royer, J., Low frequency resolvent estimates on asymptotically conical manifolds, Comm. Math. Phys. 335(2) (2015), 809–850.Google Scholar
Burq, N., Décroissance de l’énergie locale de l’équation des ondes pour le problème extérieur et absence de résonance au voisinage du réel, Acta Math. 180(1) (1998), 1–29.Google Scholar
Guillarmou, C., Hassell, A. and Sikora, A., Resolvent at low energy III: the spectral measure, Trans. Amer. Math. Soc. 365(11) (2013), 6103–6148.Google Scholar
Hosono, T. and Ogawa, T., Large time behavior and L p –L q estimate of solutions of 2-dimensional nonlinear damped wave equations, J. Differential Equations 203(1) (2004), 82–118.CrossRefGoogle Scholar
Jensen, A., Propagation estimates for Schrödinger-type operators, Trans. Amer. Math. Soc. 291(1) (1985), 129–144.Google Scholar
Jensen, A., Mourre, E. and Perry, P., Multiple commutator estimates and resolvent smoothness in quantum scattering theory, Ann. Inst. H. Poincaré Anal. Non Linéaire 41(2) (1984), 207–225.Google Scholar
Khenissi, M., Équation des ondes amorties dans un domaine extérieur, Bull. Soc. Math. France 131(2) (2003), 211–228.Google Scholar
Khenissi, M. and Royer, J., Local energy decay and smoothing effect for the damped Schrödinger equation. Preprint, arXiv:1505.07200.Google Scholar
Kopylova, E. A., Weighted energy decay for 1D wave equation, J. Math. Anal. Appl. 366(2) (2010), 494–505.Google Scholar
Lax, P. D., Morawetz, C. S. and Phillips, R. S., Exponential decay of solutions of the wave equation in the exterior of a star-shaped obstacle, Comm. Pure Appl. Math. 16 (1963), 477–486.Google Scholar
Marcati, P. and Nishihara, K., The L p –L q estimates of solutions to one-dimensional damped wave equations and their application to the compressible flow through porous media, J. Differential Equations 191(2) (2003), 445–469.Google Scholar
Melrose, R., Singularities and energy decay in acoustical scattering, Duke Math. J. 46(1) (1979), 43–59.Google Scholar
Morawetz, C. S., Ralston, J. V. and Strauss, W. A., Decay of the solution of the wave equation outside non-trapping obstacles, Comm. Pure Appl. Math. 30 (1977), 447–508.Google Scholar
Mourre, E., Absence of singular continuous spectrum for certain self-adjoint operators, Comm. Math. Phys. 78 (1981), 391–408.Google Scholar
Narazaki, T., L p -L q estimates for damped wave equations and their applications to semi-linear problem, J. Math. Soc. Japan 56(2) (2004), 585–626.Google Scholar
Nishihara, K., L p -L q estimates of solutions to the damped wave equation in 3-dimensional space and their application, Math. Z. 244(3) (2003), 631–649.Google Scholar
Ralston, J., Solution of the wave equation with localized energy, Comm. Pure Appl. Math. 22 (1969), 807–823.Google Scholar
Rauch, J., Local decay of scattering solutions to Schrödinger’s equation, Comm. Math. Phys. 61 (1978), 149–168.CrossRefGoogle Scholar
Rauch, J. and Taylor, M., Exponential decay of solutions to hyperbolic equations in bounded domains, Indiana Univ. Math. J. 24(1) (1974), 79–86.Google Scholar
Royer, J., Limiting absorption principle for the dissipative Helmholtz equation, Comm. Partial Differential Equations 35(8) (2010), 1458–1489.Google Scholar
Royer, J., Mourre’s commutators method for a dissipative form perturbation, J. Operator Theory (2016). To appear.Google Scholar
Tataru, D., Local decay of waves on asymptotically flat stationary space-times, Amer. J. Math. 135(2) (2013), 361–401.Google Scholar
Tsutsumi, Y., Local energy decay of solutions to the free Schrödinger equation in exterior domains, J. Fac. Sci. Shinshu Univ. 31 (1984), 97–108.Google Scholar
Wang, X. P., Time-decay of scattering solutions and classical trajectories, Ann. Inst. Henri. Poincaré Probab. Stat. A 47(1) (1987), 25–37.Google Scholar
Zworski, M., Semiclassical Analysis, Graduate Studies in Mathematics, vol. 138 (American Mathematical Society, Providence, RI, 2012).Google Scholar