Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-14T23:25:33.220Z Has data issue: false hasContentIssue false
  • English
  • Français

95.36 Sequences of averages revisited

Published online by Cambridge University Press:  23 January 2015

Nick Lord
Nick Lord*
Affiliation:
Tonbridge School, Kent TN9 1JP
Get access Rights & Permissions [Opens in a new window]

Abstract

An abstract is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
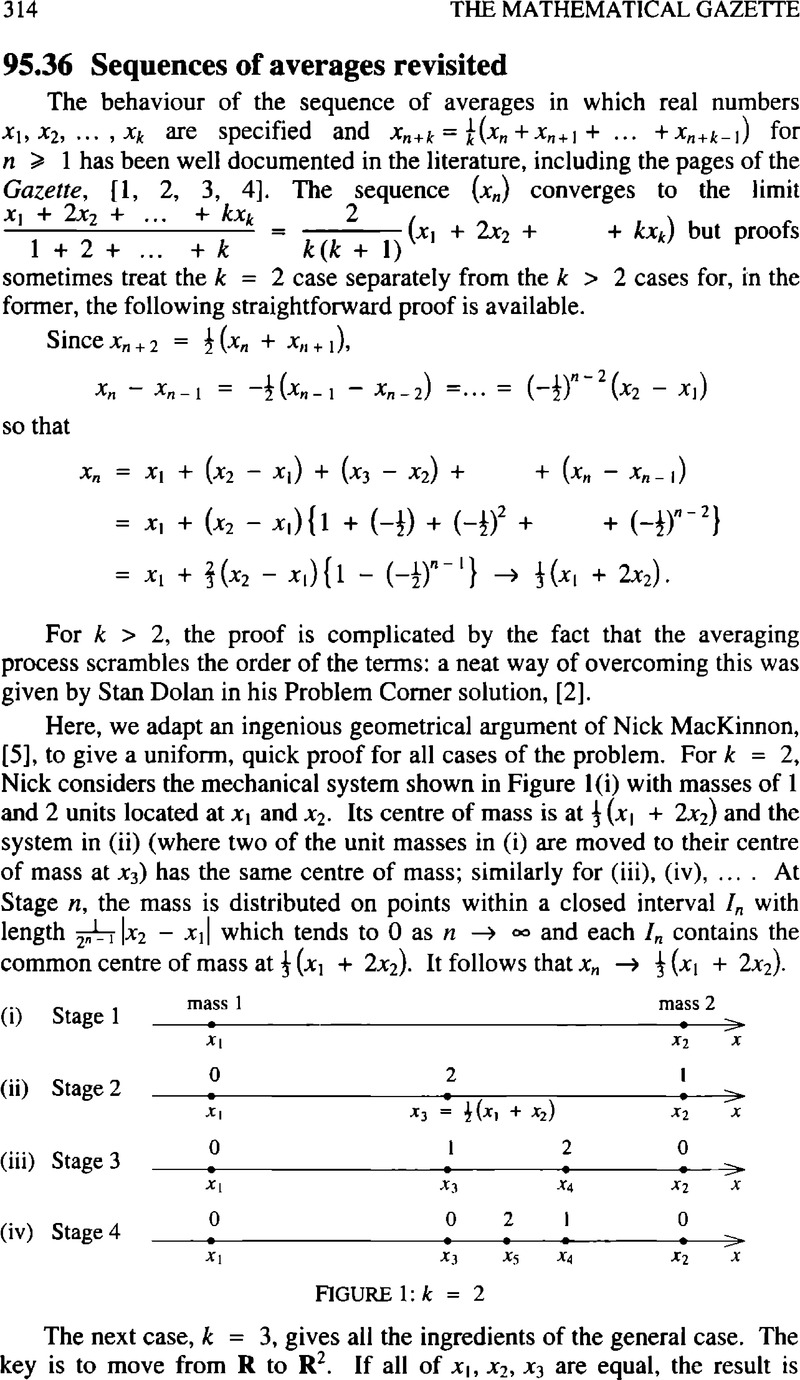
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Notes
Information
The Mathematical Gazette , Volume 95 , Issue 533 , July 2011 , pp. 314 - 317
Copyright
Copyright © The Mathematical Association 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Gowers, J., Davis, G. and Miles, E., Sequences of averages, Math. Gaz. 70 (October 1986) pp. 200–203.CrossRefGoogle Scholar
2. Problem 72.B, Problem Corner, Math. Gaz. 72 (October 1988) pp. 232–233.Google Scholar
3. Sanders, P.. Averaging sequences, Math. Gaz. 78 (November 1994) pp. 326–328.CrossRefGoogle Scholar
4. Flanders, H., Averaging sequences again, Math. Gaz. 80 (March 1996) pp.219–222.Google Scholar
5. MacKinnon, N., Centre of mass by linear transformation, Math. Gaz. 72 (March 1988) pp. 34–36.Google Scholar
6. Lord, N., An n-dimensional vector proof for Σ r2 , Math. Gaz. 72 (March 1988) pp. 33–34.Google Scholar
7. Bentin, J., Regular simplicial distances, Math. Gaz. 79 (March 1995) p. 106.Google Scholar