Rules for Bordered Magic Squares

Extract

Rouse Ball in his Mathematical Recreations tells us that there are no rules for the construction of bordered squares ; I have made the following rules, which are now published for the first time as far as I am aware.

It is remarkable that the rules for odd bordered squares are apparently not known as they are so simple. We use two diagrams, one a skeleton to make the method clear, and the other completed to show the full square.

Rule I. Place the letter M at the 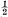(n +1 )th square as shown in Fig. 1, where n denotes the order of the square (here n = 9).

Rule II. Now, commencing at the second column, write the numbers 1, 2, 3, … up to and under M. Then write the next number in the diagonal at the bottom right-hand corner ; the next number in the same column and in the second row, and continue until we arrive at the square above M.