Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Keilson, Julian
and
Rao, S. Subba
1970.
A process with chain dependent growth rate.
Journal of Applied Probability,
Vol. 7,
Issue. 3,
p.
699.
Keilson, Julian
and
Rao, S. Subba
1970.
A process with chain dependent growth rate.
Journal of Applied Probability,
Vol. 7,
Issue. 03,
p.
699.
Brockwell, P. J.
1972.
A storage model in which the net growth-rate is a Markov chain.
Journal of Applied Probability,
Vol. 9,
Issue. 1,
p.
129.
Newbould, M.
1973.
A classification of a random walk defined on a finite Markov chain.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 26,
Issue. 2,
p.
95.
Sumita, Ushio
1984.
The matrix Laguerre transform.
Applied Mathematics and Computation,
Vol. 15,
Issue. 1,
p.
1.
Keilson, Julian
1986.
The Craft of Probabilistic Modelling.
Vol. 1,
Issue. ,
p.
166.
Ramsay, Colin M.
1987.
Advances in the Statistical Sciences: Applied Probability, Stochastic Processes, and Sampling Theory.
p.
189.
Cogger, Kenneth O.
1988.
Proposals for research in time series forecasting.
International Journal of Forecasting,
Vol. 4,
Issue. 3,
p.
403.
Asmussen, S⊘ren
and
Thorisson, Hermann
1988.
Large deviation results for time-dependent queue length distributions.
Communications in Statistics. Stochastic Models,
Vol. 4,
Issue. 1,
p.
99.
Bratiichuk, N. S.
1989.
Boundary-value problems for sums of random variables, defined on a countably valued Markov chain. I.
Ukrainian Mathematical Journal,
Vol. 41,
Issue. 2,
p.
125.
Asmussen, Søren
1989.
Risk theory in a Markovian environment.
Scandinavian Actuarial Journal,
Vol. 1989,
Issue. 2,
p.
69.
Ball, Frank
1999.
Central limit theorems for multivariate semi-Markov sequences and processes, with applications.
Journal of Applied Probability,
Vol. 36,
Issue. 02,
p.
415.
Ball, Frank
1999.
Central limit theorems for multivariate semi-Markov sequences and processes, with applications.
Journal of Applied Probability,
Vol. 36,
Issue. 2,
p.
415.
Lugavov, V. S.
2014.
A Class of Functionals on Markov Chain Transitions.
Journal of Mathematical Sciences,
Vol. 198,
Issue. 5,
p.
580.
Meyfroyt, T.M.M.
Borst, S.C.
Boxma, O.J.
and
Denteneer, D.
2015.
A data propagation model for wireless gossiping.
Performance Evaluation,
Vol. 85-86,
Issue. ,
p.
19.
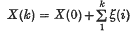 of random increments ξ(i) dependent on the chain, i.e. if the ith transition takes the chain from state r to state s, ξ,(i) is chosen from a distribution function Drs(x) indexed by r and s. The distribution of the bivariate process may be represented by a vector F(x, k) with components
of random increments ξ(i) dependent on the chain, i.e. if the ith transition takes the chain from state r to state s, ξ,(i) is chosen from a distribution function Drs(x) indexed by r and s. The distribution of the bivariate process may be represented by a vector F(x, k) with components
