Published online by Cambridge University Press: 24 October 2008
Let 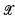 be an open Riemann surface with finite genus and finite number of boundary components, and let
be an open Riemann surface with finite genus and finite number of boundary components, and let 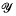 be a closed Riemann surface. An open continuous function from
be a closed Riemann surface. An open continuous function from 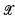 to
to 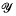 is termed a (p, q)-map, 0 < q < p, if it has a finite number of branch points and assumes every point in
is termed a (p, q)-map, 0 < q < p, if it has a finite number of branch points and assumes every point in 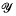 either p or q times, counting multiplicity, with possibly a finite number of exceptions. These comprise the most general class of all non-trivial functions having two valences between
either p or q times, counting multiplicity, with possibly a finite number of exceptions. These comprise the most general class of all non-trivial functions having two valences between 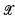 and
and 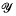 .
.
The object of this paper is to study the geometry of (p, q)-maps and establish a generalized embedding theorem which asserts that the image surfaces of (p, q)-maps embed in p-fold closed coverings possibly having branch points off the image surfaces.