Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Zhizhiashvili, L. V.
and
Topuriya, S. B.
1979.
Fourier-Laplace series on a sphere.
Journal of Soviet Mathematics,
Vol. 12,
Issue. 6,
p.
682.



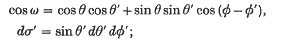
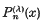 are defined by the following expansion
are defined by the following expansion
