Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-26T05:01:25.602Z Has data issue: false hasContentIssue false
  • English
  • Français

On smoothing and differentiation of tables

Published online by Cambridge University Press:  24 October 2008

H. Jeffreys
H. Jeffreys
Affiliation:
St John's College
Get access Rights & Permissions [Opens in a new window]

Extract

In recent seismological work several questions have arisen concerning the construction of tables from data containing a certain amount of accidental error, and it seems that some of the methods may be of more general interest. The first is the problem of smoothing. A method suggested by Dr L. J. Comrie was as follows. Suppose that we have values of y for five equally spaced values of x, and that we wish to make the second differences vary as smoothly as possible. We try to find a cubic polynomial such that the sum of the squares of the deviations of y from it will be a minimum. It is found that the polynomial is less than the observed value of y at the centre of the range by 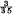of the central fourth difference; or approximately by 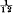of the fourth difference. I have since found that the method had previously been suggested by Sir G. H. Darwin. It gives a great improvement in the steadiness of the differences and thereby makes interpolation much easier.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 30 , Issue 2 , 30 April 1934 , pp. 134 - 138
Copyright
Copyright © Cambridge Philosophical Society 1934

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

* Monthly Notice, Roy. Astron. Soc., Geophys. Suppl. 3 (1932), 10–13.Google Scholar

† Sci. Papers, 4, 298.

* In later work some systematic errors have been traced, but here the published values are given merely for illustration.

* Whittaker, and Robinson, , Calculus of Observations, 303–312.Google Scholar