Published online by Cambridge University Press: 24 October 2008
1. In a recent paper [6], the spectrum of the Cesàro operator C on c0 (the space of null sequences of complex numbers with the sup norm) was obtained by finding the eigenvalues of the adjoint operator on  and showing that the operator (C–λI)−1 lies in B(c0) for all λ outside the closure of this set of eigenvalues. In this paper we apply a similar method to find the spectrum of the two-dimensional Cesàro operator on a space of double sequences c0(c0) (defined in §2). We shall introduce a simplification to the proof in [6] by observing that (C – λI)−1, when it exists, is a Hausdorff summability method (see page 288 of [11] for the single variable case on the space of convergent sequences c), and the crux of our proof is to show that the moment constant associated with the method (C – λI)−1 is regular for the space c0(c0) and the set of λ under consideration. It turns out that c0(c0) ≅ c0
and showing that the operator (C–λI)−1 lies in B(c0) for all λ outside the closure of this set of eigenvalues. In this paper we apply a similar method to find the spectrum of the two-dimensional Cesàro operator on a space of double sequences c0(c0) (defined in §2). We shall introduce a simplification to the proof in [6] by observing that (C – λI)−1, when it exists, is a Hausdorff summability method (see page 288 of [11] for the single variable case on the space of convergent sequences c), and the crux of our proof is to show that the moment constant associated with the method (C – λI)−1 is regular for the space c0(c0) and the set of λ under consideration. It turns out that c0(c0) ≅ c0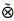 c0 (see page 237 of [7]) and that the two-dimensional Cesàro operator on c0(c0) is the tensor product C ⊗ C of the Cesàro operator C on c0. Thus our result gives a direct proof that the spectrum σ(C ⊗ C) equals σ(C)σ(C), which is a special case of the result of Schechter in [8].
c0 (see page 237 of [7]) and that the two-dimensional Cesàro operator on c0(c0) is the tensor product C ⊗ C of the Cesàro operator C on c0. Thus our result gives a direct proof that the spectrum σ(C ⊗ C) equals σ(C)σ(C), which is a special case of the result of Schechter in [8].