Article contents
Indefinite quadratic forms in many variables
Published online by Cambridge University Press: 26 February 2010
Extract
Let Q(x1, …, xn) be an indefinite quadratic form in n variables with real coefficients. It is conjectured that, provided n ≥ 5, the inequality

is soluble for every ε > 0 in integers x1, …, xn, not all 0. The first progress towards proving this conjecture was made by Davenport in two recent papers; the result obtained involved, however, a condition on the type of the form as well as on n. We say that a non-singular Q is of type (r, n—r) if, when Q is expressed as a sum of squares of n real linear forms with positive and negative signs, there are r positive signs and n—r negative signs. It was proved that (1) is always soluble provided that
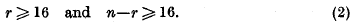
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 1958
References
page 8 note * Mathematika, 3 (1956), 81–101;CrossRefGoogle ScholarProc. London Math. Soc. (3), 8 (1958), 109–126.Google Scholar
page 8 note † Proc. Cambridge Phil. Soc, 51 (1955), 262–264CrossRefGoogle Scholar and 52 (1956), 604.
page 9 note * Bull. American Math. Soc., 51 (1945), 749–755.CrossRefGoogle Scholar
page 9 note † Journal London Math. Soc., 21 (1946), 185–193.Google Scholar
page 9 note ‡ Proc. Cambridge Phil. Soc., 54 (1958), 135–138.CrossRefGoogle Scholar
page 9 note § Mathematika, 4 (1957), 102–105.CrossRefGoogle Scholar
- 18
- Cited by

