No CrossRef data available.
Article contents
Functions meromorphic on some Riemann surfaces
Published online by Cambridge University Press: 22 January 2016
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Consider an open Riemann surface R and a single-valued meromorphic function w = f(p) defined on R. A value w0 in the extended complex plane is said to be a cluster value for w = f(p) if there exists a sequence {pn } in R accumulating at the ideal boundary of R such that
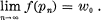
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Editorial Board of Nagoya Mathematical Journal 1978
References
[1]
Segawa, S.: On global cluster sets for functions meromorphic on some Riemann surfaces, Nagoya Math. J., 70 (1978), 1–6.Google Scholar
[2]
Stoïlow, S.: Lecon sur les principes topologiques de la théorie des fonctions analytiques, Gauthier-Villars (1956).Google Scholar
[3]
Stoïlow, S.: Sur la théorie topologique des recouvrements Riemanniens, Ann. Acad. Sci. Fenn. A.I. 250/35 (1958).Google Scholar

