Published online by Cambridge University Press: 20 January 2009
We shall concern ourselves with the class of continuous, four-parameter, one-sided probability distributions which can be characterized by the probability density function (pdf) class
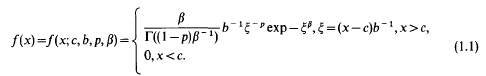
It depends on the four parameters: shift c ∈ R, scale b > 0, initial shape p < 1, and terminal shape β > 0. For p ≦ 0, the definition of f(x) can be completed by setting f(c) = β/bΓ(β−1)>0 if p = 0, and f(c) = 0 if p < 0. For 0 < p < 1, f(x) remains undefined at x = c; f(x)↑ + ∞ as x↓c.