No CrossRef data available.
Article contents
Decay properties for the incompressible Navier-Stokes flows in a half space
Published online by Cambridge University Press: 11 October 2021
Abstract
In this article, we give a comprehensive characterization of 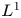 $L^1$-summability for the Navier-Stokes flows in the half space, which is a long-standing problem. The main difficulties are that
$L^1$-summability for the Navier-Stokes flows in the half space, which is a long-standing problem. The main difficulties are that  $L^q-L^r$ estimates for the Stokes flow don't work in this end-point case:
$L^q-L^r$ estimates for the Stokes flow don't work in this end-point case:  $q=r=1$; the projection operator
$q=r=1$; the projection operator  $P: L^1\longrightarrow L^1_\sigma$ is not bounded any more; useful information on the pressure function is missing, which arises in the net force exerted by the fluid on the noncompact boundary. In order to achieve our aims, by making full use of the special structure of the half space, we decompose the pressure function into two parts. Then the knotty problem of handling the pressure term can be transformed into establishing a crucial and new weighted
$P: L^1\longrightarrow L^1_\sigma$ is not bounded any more; useful information on the pressure function is missing, which arises in the net force exerted by the fluid on the noncompact boundary. In order to achieve our aims, by making full use of the special structure of the half space, we decompose the pressure function into two parts. Then the knotty problem of handling the pressure term can be transformed into establishing a crucial and new weighted 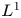 $L^1$-estimate, which plays a fundamental role. In addition, we overcome the unboundedness of the projection
$L^1$-estimate, which plays a fundamental role. In addition, we overcome the unboundedness of the projection 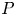 $P$ by solving an elliptic problem with homogeneous Neumann boundary condition.
$P$ by solving an elliptic problem with homogeneous Neumann boundary condition.
MSC classification
Information
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 152 , Issue 6 , December 2022 , pp. 1509 - 1532
- Copyright
- Copyright © The Author(s), 2021. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh


