Suspension splittings and James–Hopf invariants
Published online by Cambridge University Press: 30 January 2014
Abstract
James constructed a functorial homotopy decomposition 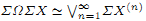 for path-connected, p ointed CW-complexes X. We generalize this to a p-local functorial decomposition of ΣA, where A is any functorial retract of a looped co-H-space. This is used to construct Hopf invariants in a more general context. In addition, when A = ΩY is the loops space of a co-H-space, we show that the wedge summands of ΣΩY further functorially decompose by using an action of an appropriate symmetric group. As a valuable example, we give an application to the theory of quasi-symmetric functions.
for path-connected, p ointed CW-complexes X. We generalize this to a p-local functorial decomposition of ΣA, where A is any functorial retract of a looped co-H-space. This is used to construct Hopf invariants in a more general context. In addition, when A = ΩY is the loops space of a co-H-space, we show that the wedge summands of ΣΩY further functorially decompose by using an action of an appropriate symmetric group. As a valuable example, we give an application to the theory of quasi-symmetric functions.
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 144 , Issue 1 , February 2014 , pp. 87 - 108
- Copyright
- Copyright © Royal Society of Edinburgh 2014
- 5
- Cited by


