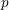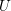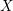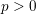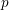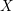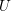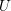Introduction
Let
![]() $k$
be a perfect field of characteristic
$k$
be a perfect field of characteristic
![]() $p>0$
and let
$p>0$
and let
![]() $X$
be a smooth proper variety of dimension
$X$
be a smooth proper variety of dimension
![]() $d$
over
$d$
over
![]() $k$
. The logarithmic de Rham–Witt sheaves
$k$
. The logarithmic de Rham–Witt sheaves
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{r}$
are defined as the subsheaves of the de Rham–Witt sheaves
$W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{r}$
are defined as the subsheaves of the de Rham–Witt sheaves
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}$
, which are étale locally generated by sections
$W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}$
, which are étale locally generated by sections
![]() $d\log [x_{1}]_{m}\wedge \cdots \wedge d\log [x_{r}]_{m}$
with
$d\log [x_{1}]_{m}\wedge \cdots \wedge d\log [x_{r}]_{m}$
with
![]() $x_{\unicode[STIX]{x1D708}}\in {\mathcal{O}}_{X}^{\times }$
for all
$x_{\unicode[STIX]{x1D708}}\in {\mathcal{O}}_{X}^{\times }$
for all
![]() $\unicode[STIX]{x1D708}$
[Reference IllusieIll79]. By the Gersten resolution [Reference RostRos96, Reference KerzKer10, Reference Gros and SuwaGS88] and the Bloch–Gabber–Kato theorem [Reference Bloch and KatoBK86], the
$\unicode[STIX]{x1D708}$
[Reference IllusieIll79]. By the Gersten resolution [Reference RostRos96, Reference KerzKer10, Reference Gros and SuwaGS88] and the Bloch–Gabber–Kato theorem [Reference Bloch and KatoBK86], the
![]() $d\log$
map induces an isomorphism of étale sheaves
$d\log$
map induces an isomorphism of étale sheaves
where
![]() ${\mathcal{K}}_{r,X}^{M}$
is the sheaf of Milnor
${\mathcal{K}}_{r,X}^{M}$
is the sheaf of Milnor
![]() $K$
-groups. It is conceived as a
$K$
-groups. It is conceived as a
![]() $p$
-adic analogue of the
$p$
-adic analogue of the
![]() $\ell$
-adic sheaf
$\ell$
-adic sheaf
![]() $\unicode[STIX]{x1D707}_{\ell ^{m}}^{\otimes r}$
with
$\unicode[STIX]{x1D707}_{\ell ^{m}}^{\otimes r}$
with
![]() $\ell \neq p$
. If
$\ell \neq p$
. If
![]() $k$
is a finite field, there is a non-degenerate pairing of finite groups due to Milne [Reference MilneMil86]:
$k$
is a finite field, there is a non-degenerate pairing of finite groups due to Milne [Reference MilneMil86]:
It induces a natural isomorphism
where
![]() $A^{\vee }$
is the Pontryagin dual of a discrete abelian group and
$A^{\vee }$
is the Pontryagin dual of a discrete abelian group and
![]() $\unicode[STIX]{x1D70B}_{1}^{ab}(X)$
is the maximal abelian quotient of Grothendieck’s étale fundamental group of
$\unicode[STIX]{x1D70B}_{1}^{ab}(X)$
is the maximal abelian quotient of Grothendieck’s étale fundamental group of
![]() $X$
. This gives a description of
$X$
. This gives a description of
![]() $\unicode[STIX]{x1D70B}_{1}^{ab}(X)/p^{m}$
in terms of étale cohomology with
$\unicode[STIX]{x1D70B}_{1}^{ab}(X)/p^{m}$
in terms of étale cohomology with
![]() $p$
-adic coefficient. For
$p$
-adic coefficient. For
![]() $\ell$
-adic étale cohomology, we also have a non-degenerate pairing of finite groups for a smooth non-proper variety
$\ell$
-adic étale cohomology, we also have a non-degenerate pairing of finite groups for a smooth non-proper variety
![]() $U$
of dimension
$U$
of dimension
![]() $d$
over a finite field
$d$
over a finite field
![]() $k$
[SGA4½, Reference SaitoSai89]
$k$
[SGA4½, Reference SaitoSai89]
which can be used to describe
![]() $\unicode[STIX]{x1D70B}_{1}^{ab}(U)/\ell ^{m}$
by
$\unicode[STIX]{x1D70B}_{1}^{ab}(U)/\ell ^{m}$
by
![]() $\ell$
-adic étale cohomology:
$\ell$
-adic étale cohomology:
In the
![]() $p$
-adic setting there is no obvious analogue of étale cohomology with compact support for logarithmic de Rham–Witt sheaves.
$p$
-adic setting there is no obvious analogue of étale cohomology with compact support for logarithmic de Rham–Witt sheaves.
In this paper, we propose a new approach. Let
![]() $X$
be a proper smooth variety over a perfect field
$X$
be a proper smooth variety over a perfect field
![]() $k$
as before, and let
$k$
as before, and let
![]() $j:U{\hookrightarrow}X$
be the complement of an effective divisor
$j:U{\hookrightarrow}X$
be the complement of an effective divisor
![]() $D$
such that Supp(
$D$
such that Supp(
![]() $D$
) has simple normal crossings. We introduce new
$D$
) has simple normal crossings. We introduce new
![]() $p$
-primary torsion sheaves
$p$
-primary torsion sheaves
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
(see Definition 1.1.1), which we call relative logarithmic de Rham–Witt sheaves. It is defined as the subsheaf of the de Rham–Witt sheaf
$W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
(see Definition 1.1.1), which we call relative logarithmic de Rham–Witt sheaves. It is defined as the subsheaf of the de Rham–Witt sheaf
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}$
which is étale locally generated by sections
$W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}$
which is étale locally generated by sections
![]() $d\log [x_{1}]_{m}\wedge \ldots \wedge d\log [x_{r}]_{m}$
with
$d\log [x_{1}]_{m}\wedge \ldots \wedge d\log [x_{r}]_{m}$
with
![]() $x_{1}\in \text{Ker}({\mathcal{O}}_{X}^{\times }\rightarrow {\mathcal{O}}_{D}^{\times })$
, and
$x_{1}\in \text{Ker}({\mathcal{O}}_{X}^{\times }\rightarrow {\mathcal{O}}_{D}^{\times })$
, and
![]() $x_{\unicode[STIX]{x1D708}}\in j_{\ast }{\mathcal{O}}_{U}^{\times }$
for all
$x_{\unicode[STIX]{x1D708}}\in j_{\ast }{\mathcal{O}}_{U}^{\times }$
for all
![]() $\unicode[STIX]{x1D708}$
. As in the classical situation, we have the following theorem.
$\unicode[STIX]{x1D708}$
. As in the classical situation, we have the following theorem.
Theorem 1 (see Theorem 1.1.5).
The map
![]() $d\log$
induces an isomorphism
$d\log$
induces an isomorphism
Here
![]() ${\mathcal{K}}_{r,X|D}^{M}$
is the sheaf of relative Milnor
${\mathcal{K}}_{r,X|D}^{M}$
is the sheaf of relative Milnor
![]() $K$
-groups which has been studied by one of the authors (Saito) and Rülling in [Reference Rülling and SaitoRS18].
$K$
-groups which has been studied by one of the authors (Saito) and Rülling in [Reference Rülling and SaitoRS18].
If
![]() $D_{1}\geqslant D_{2}$
, we have inclusions (see Proposition 1.1.4)
$D_{1}\geqslant D_{2}$
, we have inclusions (see Proposition 1.1.4)
and thus obtain a pro-system of
![]() $\mathbb{Z}/p^{m}\mathbb{Z}$
-sheaves “
$\mathbb{Z}/p^{m}\mathbb{Z}$
-sheaves “
![]() $\mathop{\varprojlim }\nolimits_{D}$
”
$\mathop{\varprojlim }\nolimits_{D}$
”
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
, where
$W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
, where
![]() $D$
runs over the set of all effective divisors with
$D$
runs over the set of all effective divisors with
![]() $\text{Supp}(D)\subset X-U$
.
$\text{Supp}(D)\subset X-U$
.
In case
![]() $m=1$
these sheaves are related to sheaves of differential forms by the exact sequence (see Theorem 1.2.1)
$m=1$
these sheaves are related to sheaves of differential forms by the exact sequence (see Theorem 1.2.1)
where
![]() $\unicode[STIX]{x1D6FA}_{X|D}^{r}=\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)\otimes _{{\mathcal{O}}_{X}}{\mathcal{O}}_{X}(-D)$
and
$\unicode[STIX]{x1D6FA}_{X|D}^{r}=\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)\otimes _{{\mathcal{O}}_{X}}{\mathcal{O}}_{X}(-D)$
and
![]() $C^{-1}$
is the inverse Cartier morphism. In order to extend the above exact sequence to the case
$C^{-1}$
is the inverse Cartier morphism. In order to extend the above exact sequence to the case
![]() $m>1$
, we need introduce the filtered relative de Rham–Witt complex
$m>1$
, we need introduce the filtered relative de Rham–Witt complex
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D}^{\bullet }$
for which we have
$W_{m}\unicode[STIX]{x1D6FA}_{X|D}^{\bullet }$
for which we have
![]() $W_{1}\unicode[STIX]{x1D6FA}_{X|D}^{\bullet }=\unicode[STIX]{x1D6FA}_{X|D}^{\bullet }$
(see § 2.3 and Theorem 2.3.1). Its construction uses the de Rham–Witt complexes in log geometry [Reference Hyodo and KatoHK94], which can be seen as the higher analogue of
$W_{1}\unicode[STIX]{x1D6FA}_{X|D}^{\bullet }=\unicode[STIX]{x1D6FA}_{X|D}^{\bullet }$
(see § 2.3 and Theorem 2.3.1). Its construction uses the de Rham–Witt complexes in log geometry [Reference Hyodo and KatoHK94], which can be seen as the higher analogue of
![]() $\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)$
.
$\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)$
.
Using the generalization of (3) to the case
![]() $m>1$
, we can define a pairing between
$m>1$
, we can define a pairing between
![]() $W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
and the pro-system “
$W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
and the pro-system “
![]() $\mathop{\varprojlim }\nolimits_{D}$
”
$\mathop{\varprojlim }\nolimits_{D}$
”
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r}$
and obtain the following theorem.
$W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r}$
and obtain the following theorem.
Theorem 2 (see Theorem 4.1.4).
Let
![]() $X$
,
$X$
,
![]() $D$
and
$D$
and
![]() $U$
be as above and assume that
$U$
be as above and assume that
![]() $k$
is finite. Then the groups
$k$
is finite. Then the groups
![]() $H^{j}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r})$
are finite and there are natural perfect pairings of topological abelian groups
$H^{j}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r})$
are finite and there are natural perfect pairings of topological abelian groups
where the first group is endowed with discrete topology, and the second is endowed with profinite topology.
From the case
![]() $i=1$
and
$i=1$
and
![]() $r=0$
of the above theorem, we get a natural isomorphism
$r=0$
of the above theorem, we get a natural isomorphism
which gives rise to a series of quotients
![]() $\unicode[STIX]{x1D70B}_{1}^{\text{ab}}(X,D)/p^{m}$
of
$\unicode[STIX]{x1D70B}_{1}^{\text{ab}}(X,D)/p^{m}$
of
![]() $\unicode[STIX]{x1D70B}_{1}^{\text{ab}}(U)/p^{m}$
using the inverse limit. It is thought of as classifying abelian étale covering of
$\unicode[STIX]{x1D70B}_{1}^{\text{ab}}(U)/p^{m}$
using the inverse limit. It is thought of as classifying abelian étale covering of
![]() $U$
whose degree divides
$U$
whose degree divides
![]() $p^{m}$
and ramification is bounded by the divisor
$p^{m}$
and ramification is bounded by the divisor
![]() $D$
.
$D$
.
One of the authors (Zhao) [Reference ZhaoZha16] has proved a similar duality theorem for a projective semi-stable scheme over an equi-characteristic discrete valuation ring
![]() $k[[t]]$
with
$k[[t]]$
with
![]() $k$
finite.
$k$
finite.
When the base field
![]() $k$
is prefect but not necessarily finite, we follow the method of Milne [Reference MilneMil86] and work in the category
$k$
is prefect but not necessarily finite, we follow the method of Milne [Reference MilneMil86] and work in the category
![]() $\mathscr{S}(p^{m})$
of
$\mathscr{S}(p^{m})$
of
![]() $\mathbb{Z}/p^{m}\mathbb{Z}$
-sheaves on perfect étale site
$\mathbb{Z}/p^{m}\mathbb{Z}$
-sheaves on perfect étale site
![]() $(\mathit{Pf}/k)_{\acute{\text{e}}\text{t}}$
(see § 5.1). Let
$(\mathit{Pf}/k)_{\acute{\text{e}}\text{t}}$
(see § 5.1). Let
![]() $D^{b}(\mathscr{S}(p^{m}))$
be the derived category of bounded complexes in
$D^{b}(\mathscr{S}(p^{m}))$
be the derived category of bounded complexes in
![]() $\mathscr{S}(p^{m})$
. We then get from
$\mathscr{S}(p^{m})$
. We then get from
![]() $X,\;D$
objects of
$X,\;D$
objects of
![]() $D^{b}(\mathscr{S}(p^{m}))$
:
$D^{b}(\mathscr{S}(p^{m}))$
:
where
![]() $\unicode[STIX]{x1D70B}:X\rightarrow S=\text{Spec}(k)$
is the structure morphism and
$\unicode[STIX]{x1D70B}:X\rightarrow S=\text{Spec}(k)$
is the structure morphism and
![]() $j:U\rightarrow X$
is the open immersion. Then our duality theorem reads as follows.
$j:U\rightarrow X$
is the open immersion. Then our duality theorem reads as follows.
Theorem 3 (see Theorem 5.2.1).
There is a natural isomorphism in
![]() $D^{b}(\mathscr{S}(p^{m}))$
:
$D^{b}(\mathscr{S}(p^{m}))$
:
where
![]() $R\,\mathop{\varprojlim }\nolimits_{D}$
denotes the homotopy limit over effective Cartier divisors supported on
$R\,\mathop{\varprojlim }\nolimits_{D}$
denotes the homotopy limit over effective Cartier divisors supported on
![]() $X-U$
.
$X-U$
.
The paper is organized as follows.
In § 1, we study the two important results on the relative logarithmic de Rham–Witt sheaves: the first one is a computation of the kernel of the restriction map
![]() $R^{m-1}:W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}\rightarrow W_{1}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
; the second is the exact sequence (3).
$R^{m-1}:W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}\rightarrow W_{1}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
; the second is the exact sequence (3).
In order to define the desired pairing, we introduce filtered de Rham–Witt complexes in § 2, and study the behavior of Frobenius and Verschiebung morphisms on these complexes.
Using two-term complexes, we define the pairing in § 3 and prove its perfectness when the base field
![]() $k$
is finite in § 4. The last § 5 is on the duality over a general perfect field.
$k$
is finite in § 4. The last § 5 is on the duality over a general perfect field.
1 Relative logarithmic de Rham–Witt sheaves
Let
![]() $X$
be a smooth proper variety of dimension
$X$
be a smooth proper variety of dimension
![]() $d$
over a perfect field
$d$
over a perfect field
![]() $k$
of characteristic
$k$
of characteristic
![]() $p>0$
, let
$p>0$
, let
![]() $D$
be an effective divisor such that
$D$
be an effective divisor such that
![]() $\text{Supp}(D)$
is a simple normal crossing divisor on
$\text{Supp}(D)$
is a simple normal crossing divisor on
![]() $X$
, and let
$X$
, and let
![]() $j:U:=X-D{\hookrightarrow}X$
be the complement of
$j:U:=X-D{\hookrightarrow}X$
be the complement of
![]() $D$
.
$D$
.
1.1 Basic properties
Definition 1.1.1. For
![]() $r\in \mathbb{N}$
let
$r\in \mathbb{N}$
let
be the subsheaf generated étale locally by sections
For
![]() $r\in \mathbb{N}$
let
$r\in \mathbb{N}$
let
![]() ${\mathcal{K}}_{r,X}^{M}$
be the
${\mathcal{K}}_{r,X}^{M}$
be the
![]() $r$
th Milnor
$r$
th Milnor
![]() $K$
-sheaf on
$K$
-sheaf on
![]() $X_{\acute{\text{e}}\text{t}}$
given by
$X_{\acute{\text{e}}\text{t}}$
given by
where
![]() $V^{(i)}$
is the set of points of codimension
$V^{(i)}$
is the set of points of codimension
![]() $i$
in
$i$
in
![]() $V$
, for
$V$
, for
![]() $i=0,1$
, and
$i=0,1$
, and
![]() $\unicode[STIX]{x2202}_{x}:K_{r}^{M}(k(\unicode[STIX]{x1D702}))\rightarrow K_{r}^{M}(k(x))$
is the tame symbol from [Reference Bass and TateBT73, § 4]. By [Reference KerzKer10, Proposition 10(8) and Theorem 13],
$\unicode[STIX]{x2202}_{x}:K_{r}^{M}(k(\unicode[STIX]{x1D702}))\rightarrow K_{r}^{M}(k(x))$
is the tame symbol from [Reference Bass and TateBT73, § 4]. By [Reference KerzKer10, Proposition 10(8) and Theorem 13],
![]() ${\mathcal{K}}_{r,X}^{M}$
is étale locally generated by symbols
${\mathcal{K}}_{r,X}^{M}$
is étale locally generated by symbols
![]() $\{x_{1},\ldots ,x_{r}\}$
with
$\{x_{1},\ldots ,x_{r}\}$
with
![]() $x_{i}\in {\mathcal{O}}_{X,x}^{\times }$
. We have a natural isomorphism of étale sheaves
$x_{i}\in {\mathcal{O}}_{X,x}^{\times }$
. We have a natural isomorphism of étale sheaves
This follows from the Gersten resolutions of
![]() $\unicode[STIX]{x1D716}_{\ast }{\mathcal{K}}_{r,X}^{M}$
and
$\unicode[STIX]{x1D716}_{\ast }{\mathcal{K}}_{r,X}^{M}$
and
![]() $\unicode[STIX]{x1D716}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{r}$
from [Reference RostRos96] and [Reference Gros and SuwaGS88] together with the Bloch–Gabber–Kato theorem [Reference Bloch and KatoBK86], where
$\unicode[STIX]{x1D716}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{r}$
from [Reference RostRos96] and [Reference Gros and SuwaGS88] together with the Bloch–Gabber–Kato theorem [Reference Bloch and KatoBK86], where
![]() $\unicode[STIX]{x1D716}:X_{\acute{\text{e}}\text{t}}\rightarrow X_{\text{Zar}}$
is the map of sites.
$\unicode[STIX]{x1D716}:X_{\acute{\text{e}}\text{t}}\rightarrow X_{\text{Zar}}$
is the map of sites.
Definition 1.1.2 [Reference Rülling and SaitoRS18, Definition 2.4].
For
![]() $r\in \mathbb{N}$
, we define the relative Milnor
$r\in \mathbb{N}$
, we define the relative Milnor
![]() $K$
-sheaf
$K$
-sheaf
![]() ${\mathcal{K}}_{r,X|D}^{M}$
to be image of the map
${\mathcal{K}}_{r,X|D}^{M}$
to be image of the map
Using some symbol calculations, we get the following proposition.
Proposition 1.1.3 [Reference Rülling and SaitoRS18, Corollary 2.9].
Let
![]() $D_{1},D_{2}$
be two effective divisors on
$D_{1},D_{2}$
be two effective divisors on
![]() $X$
whose supports are simple normal crossing divisors. Assume
$X$
whose supports are simple normal crossing divisors. Assume
![]() $D_{1}\leqslant D_{2}$
. Then we have the inclusions of sheaves
$D_{1}\leqslant D_{2}$
. Then we have the inclusions of sheaves
Corollary 1.1.4. Under the assumption of Proposition 1.1.3, we have inclusions
Proof. This follows from the fact that the sheaf
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
is the image of
$W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
is the image of
![]() ${\mathcal{K}}_{r,X|D}^{M}$
under the map
${\mathcal{K}}_{r,X|D}^{M}$
under the map
![]() $d\log [-]$
.◻
$d\log [-]$
.◻
The isomorphism (1.1.1) also has the following relative version.
Theorem 1.1.5. The
![]() $d\log$
map induces an isomorphism of étale sheaves
$d\log$
map induces an isomorphism of étale sheaves
Proof. The assertion follows directly by the following commutative diagram.

In the rest of this section, we will prove two fundamental results for the relative logarithmic de Rham–Witt sheaves.
Theorem 1.1.6. Write
![]() $D=\sum _{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}n_{\unicode[STIX]{x1D706}}D_{\unicode[STIX]{x1D706}}$
, where
$D=\sum _{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}n_{\unicode[STIX]{x1D706}}D_{\unicode[STIX]{x1D706}}$
, where
![]() $D_{\unicode[STIX]{x1D706}}$
(
$D_{\unicode[STIX]{x1D706}}$
(
![]() $\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}$
) are irreducible components of
$\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}$
) are irreducible components of
![]() $D$
. Then we have an exact sequence
$D$
. Then we have an exact sequence
where
![]() $[D/p]=\sum _{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}[n_{\unicode[STIX]{x1D706}}/p]D_{\unicode[STIX]{x1D706}}$
with
$[D/p]=\sum _{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}[n_{\unicode[STIX]{x1D706}}/p]D_{\unicode[STIX]{x1D706}}$
with
![]() $[n/p]=\min \{n^{\prime }\in \mathbb{Z}\mid pn^{\prime }\geqslant n\}.$
$[n/p]=\min \{n^{\prime }\in \mathbb{Z}\mid pn^{\prime }\geqslant n\}.$
Let
![]() $R$
be the henselization of a local ring of a smooth scheme over a field
$R$
be the henselization of a local ring of a smooth scheme over a field
![]() $k$
of characteristic
$k$
of characteristic
![]() $p>0$
. Let
$p>0$
. Let
![]() $(T_{1},\ldots ,T_{d})\subset R$
be a part of a system of regular parameters and put
$(T_{1},\ldots ,T_{d})\subset R$
be a part of a system of regular parameters and put
![]() $T=T_{1}\cdots T_{d}$
. We endow
$T=T_{1}\cdots T_{d}$
. We endow
![]() $\mathbb{N}^{d}$
with a semi-order by
$\mathbb{N}^{d}$
with a semi-order by
and put
Following [Reference Bloch and KatoBK86, § 4], we define
![]() $U^{\text{}\underline{n}}K_{r}^{M}(R)\subset K_{r}^{M}(R)$
for
$U^{\text{}\underline{n}}K_{r}^{M}(R)\subset K_{r}^{M}(R)$
for
![]() $\text{}\underline{n}=(n_{1},\ldots ,n_{d})\in \mathbb{N}^{d}$
as the subgroup generated by symbols
$\text{}\underline{n}=(n_{1},\ldots ,n_{d})\in \mathbb{N}^{d}$
as the subgroup generated by symbols
(Here having the injectivity of
![]() $K_{r}^{M}(R)\rightarrow K_{r}^{M}(K)$
with the quotient field
$K_{r}^{M}(R)\rightarrow K_{r}^{M}(K)$
with the quotient field
![]() $K$
of
$K$
of
![]() $R$
, the above symbols are considered in
$R$
, the above symbols are considered in
![]() $K_{r}^{M}(K)$
.) For an integer
$K_{r}^{M}(K)$
.) For an integer
![]() $m>0$
, put
$m>0$
, put
Theorem 1.1.7. We have the following exact sequence:
where
![]() $[\text{}\underline{n}/p]=\min \{\text{}\underline{\unicode[STIX]{x1D708}}\in \mathbb{N}^{d}|\;p\text{}\underline{\unicode[STIX]{x1D708}}\geqslant \text{}\underline{n}\}\in \mathbb{N}^{d}$
.
$[\text{}\underline{n}/p]=\min \{\text{}\underline{\unicode[STIX]{x1D708}}\in \mathbb{N}^{d}|\;p\text{}\underline{\unicode[STIX]{x1D708}}\geqslant \text{}\underline{n}\}\in \mathbb{N}^{d}$
.
For the proof we compute
We need some preliminaries. For
![]() $\text{}\underline{n}\in \mathbb{N}^{d}$
and
$\text{}\underline{n}\in \mathbb{N}^{d}$
and
![]() $1\leqslant i\leqslant d$
and an integer
$1\leqslant i\leqslant d$
and an integer
![]() $q\geqslant 1$
put
$q\geqslant 1$
put
where
![]() $I^{\text{}\underline{n}}=(T_{1}^{n_{1}}\cdots T_{d}^{n_{d}})\subset R$
and
$I^{\text{}\underline{n}}=(T_{1}^{n_{1}}\cdots T_{d}^{n_{d}})\subset R$
and
![]() $\unicode[STIX]{x1D6FA}_{R}^{q}(\log T)$
is the sheaf of (absolute) differential
$\unicode[STIX]{x1D6FA}_{R}^{q}(\log T)$
is the sheaf of (absolute) differential
![]() $q$
-forms of
$q$
-forms of
![]() $R$
with logarithmic poles along
$R$
with logarithmic poles along
![]() $T=0$
. It is easy to check the exterior derivative induces
$T=0$
. It is easy to check the exterior derivative induces
Put
We can easily check the following.
Lemma 1.1.8 [Reference Rülling and SaitoRS18, Theorem 2.16].
Let the notation be as above. Then the inverse Cartier morphism
induces an isomorphism
We define subgroups
by the inductive formulae
Proposition 1.1.9. Fix
![]() $\text{}\underline{n}=(n_{1},\ldots ,n_{d})\in \mathbb{N}^{d}$
and
$\text{}\underline{n}=(n_{1},\ldots ,n_{d})\in \mathbb{N}^{d}$
and
![]() $1\leqslant i\leqslant d$
.
$1\leqslant i\leqslant d$
.
(1) There is a natural map
such that for $$\begin{eqnarray}\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}:\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}\rightarrow gr^{\text{}\underline{n},i}k_{r}^{M}(R)_{m}\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}:\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}\rightarrow gr^{\text{}\underline{n},i}k_{r}^{M}(R)_{m}\end{eqnarray}$$
 $a\in R$
,
$a\in R$
,
 $b_{2},\ldots ,b_{d}\in R[1/T]^{\times }$
,
$b_{2},\ldots ,b_{d}\in R[1/T]^{\times }$
,  $$\begin{eqnarray}\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}\bigg(a(T_{1}^{n_{1}}\cdots T_{d}^{n_{d}})\displaystyle \frac{db_{2}}{b_{2}}\wedge \cdots \wedge \displaystyle \frac{db_{r}}{b_{r}}\bigg)=\{1+aT_{1}^{n_{1}}\cdots T_{d}^{n_{d}},b_{2},\ldots ,b_{r}\}\in U^{\text{}\underline{n}}K_{r}^{M}(R).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}\bigg(a(T_{1}^{n_{1}}\cdots T_{d}^{n_{d}})\displaystyle \frac{db_{2}}{b_{2}}\wedge \cdots \wedge \displaystyle \frac{db_{r}}{b_{r}}\bigg)=\{1+aT_{1}^{n_{1}}\cdots T_{d}^{n_{d}},b_{2},\ldots ,b_{r}\}\in U^{\text{}\underline{n}}K_{r}^{M}(R).\end{eqnarray}$$
(2) Write
 $n_{i}=p^{s}\cdot n^{\prime }$
with
$n_{i}=p^{s}\cdot n^{\prime }$
with
 $p\not |n^{\prime }$
. If
$p\not |n^{\prime }$
. If
 $m>s$
,
$m>s$
,
 $\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}$
induces an isomorphism If
$\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}$
induces an isomorphism If $$\begin{eqnarray}\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}/B_{s|\text{}\underline{n},i}^{r-1}\xrightarrow[{}]{\cong }gr^{\text{}\underline{n},i}k_{r}^{M}(R)_{m}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}/B_{s|\text{}\underline{n},i}^{r-1}\xrightarrow[{}]{\cong }gr^{\text{}\underline{n},i}k_{r}^{M}(R)_{m}.\end{eqnarray}$$
 $m\leqslant s$
,
$m\leqslant s$
,
 $\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}$
induces an isomorphism
$\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}$
induces an isomorphism  $$\begin{eqnarray}\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}/Z_{m|\text{}\underline{n},i}^{r-1}\xrightarrow[{}]{\cong }gr^{\text{}\underline{n},i}k_{r}^{M}(R)_{m}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}/Z_{m|\text{}\underline{n},i}^{r-1}\xrightarrow[{}]{\cong }gr^{\text{}\underline{n},i}k_{r}^{M}(R)_{m}.\end{eqnarray}$$
Proof. The existence of
![]() $\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}$
together with the fact that it induces the surjective maps as in (2) is shown by the same argument as [Reference Bloch and KatoBK86, (4.5) and (4.6)]. Note that
$\unicode[STIX]{x1D70C}_{\text{}\underline{n},i}$
together with the fact that it induces the surjective maps as in (2) is shown by the same argument as [Reference Bloch and KatoBK86, (4.5) and (4.6)]. Note that
![]() $\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}/B_{s|\text{}\underline{n},i}^{r-1}$
and
$\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}/B_{s|\text{}\underline{n},i}^{r-1}$
and
![]() $\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}/Z_{m|\text{}\underline{n},i}^{r-1}$
are free
$\unicode[STIX]{x1D714}_{\text{}\underline{n},i}^{r-1}/Z_{m|\text{}\underline{n},i}^{r-1}$
are free
![]() $R_{i}^{p^{e}}$
-modules, for some
$R_{i}^{p^{e}}$
-modules, for some
![]() $e\gg 0$
. By localization, the injectivity of the maps is reduced to the case
$e\gg 0$
. By localization, the injectivity of the maps is reduced to the case
![]() $R$
is a discrete valuation ring, which has been treated in [Reference Bloch and KatoBK86, (4.8)].◻
$R$
is a discrete valuation ring, which has been treated in [Reference Bloch and KatoBK86, (4.8)].◻
Now we prove Theorem 1.1.7. It is easy to see that we have a complex as in the theorem. Its exactness on the left follows from the fact that
![]() $K_{r}^{M}(R)$
is
$K_{r}^{M}(R)$
is
![]() $p$
-torsion free (cf. [Reference Geisser and LevineGL00, Theorem 8.1] and [Reference RostRos96, Theorem 6.1]). It remains to show the exactness in the middle. For this it suffices to show the injectivity of the map induced by multiplication by
$p$
-torsion free (cf. [Reference Geisser and LevineGL00, Theorem 8.1] and [Reference RostRos96, Theorem 6.1]). It remains to show the exactness in the middle. For this it suffices to show the injectivity of the map induced by multiplication by
![]() $p$
:
$p$
:
This follows from the following claims.
Claim 1.1.10. The multiplication by
![]() $p$
induces an injective map:
$p$
induces an injective map:
Proof. We have a map (cf. [Reference Rülling and SaitoRS18, the first displayed formula in the proof of Proposition 2.10])
where
![]() $(a~\text{mod}~T_{i})\in R_{i}$
is the image of
$(a~\text{mod}~T_{i})\in R_{i}$
is the image of
![]() $a\in R$
. By [Reference Rülling and SaitoRS18, Proposition 2.10] and Proposition 1.1.3, we see that this map is injective. Combining with the fact that
$a\in R$
. By [Reference Rülling and SaitoRS18, Proposition 2.10] and Proposition 1.1.3, we see that this map is injective. Combining with the fact that
![]() $\bigoplus _{1\leqslant i\leqslant d}K_{r}^{M}(R_{i})$
is
$\bigoplus _{1\leqslant i\leqslant d}K_{r}^{M}(R_{i})$
is
![]() $p$
-torsion free, we conclude this claim.◻
$p$
-torsion free, we conclude this claim.◻
Claim 1.1.11. For
![]() $\text{}\underline{n}$
and
$\text{}\underline{n}$
and
![]() $i$
as in Proposition 1.1.9, the multiplication by
$i$
as in Proposition 1.1.9, the multiplication by
![]() $p$
induces an injective map:
$p$
induces an injective map:
Proof. It is easy to check that the multiplication by
![]() $p$
induces such a map. Its injectivity follows from the commutative diagram
$p$
induces such a map. Its injectivity follows from the commutative diagram

and the commutative diagram

where the vertical isomorphisms are from Proposition 1.1.9. ◻
1.2 Relation with differential forms
The sheaf
![]() $\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
relates to coherent sheaves as follows.
$\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
relates to coherent sheaves as follows.
Theorem 1.2.1. We have an exact sequence
where
![]() $\unicode[STIX]{x1D6FA}_{X|D}^{r}=\unicode[STIX]{x1D6FA}_{X/k}^{r}(\log D)\otimes _{{\mathcal{O}}_{X}}{\mathcal{O}}_{X}(-D)$
.
$\unicode[STIX]{x1D6FA}_{X|D}^{r}=\unicode[STIX]{x1D6FA}_{X/k}^{r}(\log D)\otimes _{{\mathcal{O}}_{X}}{\mathcal{O}}_{X}(-D)$
.
Proof. For the exactness on the right, it suffices to show the surjectivity of
![]() $1-C^{-1}$
on sections over the strict henselization of a local ring of
$1-C^{-1}$
on sections over the strict henselization of a local ring of
![]() $X$
. In fact, by the argument in the classical case where
$X$
. In fact, by the argument in the classical case where
![]() $D=\emptyset$
[Reference MilneMil76, Lemma 1.3], it suffices to show the following claim.
$D=\emptyset$
[Reference MilneMil76, Lemma 1.3], it suffices to show the following claim.
Claim 1.2.2. Let
![]() $A$
be a strictly henselian regular local ring of equi-characteristic
$A$
be a strictly henselian regular local ring of equi-characteristic
![]() $p>0$
and
$p>0$
and
![]() $\mathfrak{m}\subset A$
be the maximal ideal. Let
$\mathfrak{m}\subset A$
be the maximal ideal. Let
![]() $\unicode[STIX]{x1D70B}\in \mathfrak{m}$
and
$\unicode[STIX]{x1D70B}\in \mathfrak{m}$
and
![]() $a\in A$
. If
$a\in A$
. If
![]() $a\in \unicode[STIX]{x1D70B}A$
, then there exists
$a\in \unicode[STIX]{x1D70B}A$
, then there exists
![]() $b\in A$
, such that
$b\in A$
, such that
![]() $b\in \unicode[STIX]{x1D70B}A$
and
$b\in \unicode[STIX]{x1D70B}A$
and
![]() $b^{p}-b=a$
.
$b^{p}-b=a$
.
Proof of Claim 1.2.2.
Let
![]() $k$
be the residue field of
$k$
be the residue field of
![]() $A$
. Since
$A$
. Since
![]() $\unicode[STIX]{x1D719}:A\rightarrow A$
is surjective, there exists
$\unicode[STIX]{x1D719}:A\rightarrow A$
is surjective, there exists
![]() $\tilde{b}\in A$
such that
$\tilde{b}\in A$
such that
![]() $\tilde{b}^{p}-\tilde{b}=a$
. Letting
$\tilde{b}^{p}-\tilde{b}=a$
. Letting
![]() $\unicode[STIX]{x1D6FD}\in k$
be the image of
$\unicode[STIX]{x1D6FD}\in k$
be the image of
![]() $\tilde{b}$
,
$\tilde{b}$
,
![]() $\unicode[STIX]{x1D6FD}^{p}-\unicode[STIX]{x1D6FD}=0\in k$
by the assumption
$\unicode[STIX]{x1D6FD}^{p}-\unicode[STIX]{x1D6FD}=0\in k$
by the assumption
![]() $a\in \unicode[STIX]{x1D70B}A\subset \mathfrak{m}$
. Hence
$a\in \unicode[STIX]{x1D70B}A\subset \mathfrak{m}$
. Hence
![]() $\unicode[STIX]{x1D6FD}\in \mathbb{F}_{p}\subset A$
and we put
$\unicode[STIX]{x1D6FD}\in \mathbb{F}_{p}\subset A$
and we put
![]() $b=\tilde{b}-\unicode[STIX]{x1D6FD}\in A$
. Then
$b=\tilde{b}-\unicode[STIX]{x1D6FD}\in A$
. Then
Since
![]() $b\in \mathfrak{m}_{A}$
by the construction,
$b\in \mathfrak{m}_{A}$
by the construction,
![]() $b^{p-1}-1\in A^{\times }$
and we get
$b^{p-1}-1\in A^{\times }$
and we get
![]() $b\in \unicode[STIX]{x1D70B}A$
.◻
$b\in \unicode[STIX]{x1D70B}A$
.◻
It remains to show the exactness in the middle, i.e., to show that
![]() $\unicode[STIX]{x1D6FA}_{X|D}^{r}\cap \unicode[STIX]{x1D6FA}_{X,\log }^{r}=\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
. This is a étale local question, which is a consequence of Proposition 1.2.3 below, which is a refinement of [Reference Kato and DennisKat82, Proposition 1].◻
$\unicode[STIX]{x1D6FA}_{X|D}^{r}\cap \unicode[STIX]{x1D6FA}_{X,\log }^{r}=\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
. This is a étale local question, which is a consequence of Proposition 1.2.3 below, which is a refinement of [Reference Kato and DennisKat82, Proposition 1].◻
Let
![]() $R$
be the henselization of a local ring of
$R$
be the henselization of a local ring of
![]() $X$
and choose a system
$X$
and choose a system
![]() $T_{1},\ldots ,T_{d}$
of regular parameters of
$T_{1},\ldots ,T_{d}$
of regular parameters of
![]() $R$
such that
$R$
such that
![]() $\text{Supp}(D)=\text{Spec}(R/(T_{1}\cdots T_{e}))\subset \text{Spec}(R)$
for some
$\text{Supp}(D)=\text{Spec}(R/(T_{1}\cdots T_{e}))\subset \text{Spec}(R)$
for some
![]() $e\leqslant d=\text{dim}(R)$
. Let
$e\leqslant d=\text{dim}(R)$
. Let
![]() $\unicode[STIX]{x1D6FA}_{R}^{1}(\log D)$
denotes the module of differentials with logarithmic poles along
$\unicode[STIX]{x1D6FA}_{R}^{1}(\log D)$
denotes the module of differentials with logarithmic poles along
![]() $D$
and put
$D$
and put
![]() $\unicode[STIX]{x1D6FA}_{R}^{q}(\log D)=\overset{q}{\wedge }\;\unicode[STIX]{x1D6FA}_{R}^{1}(\log D)$
. For a tuple of integers
$\unicode[STIX]{x1D6FA}_{R}^{q}(\log D)=\overset{q}{\wedge }\;\unicode[STIX]{x1D6FA}_{R}^{1}(\log D)$
. For a tuple of integers
![]() $\text{}\underline{n}=(n_{1},\ldots ,n_{e})$
with
$\text{}\underline{n}=(n_{1},\ldots ,n_{e})$
with
![]() $n_{i}\geqslant 1$
, put
$n_{i}\geqslant 1$
, put
Proposition 1.2.3.
![]() $G^{\text{}\underline{n}}\unicode[STIX]{x1D708}_{R}(q)$
is generated by elements of the form
$G^{\text{}\underline{n}}\unicode[STIX]{x1D708}_{R}(q)$
is generated by elements of the form
Proof. The following argument is a variant of Part (B) of the proof of [Reference Kato and DennisKat82, Proposition 1 (see p. 224)]. By [Reference ArtinArt69], we may replace
![]() $R$
by
$R$
by
![]() $R=k[[T_{1},\ldots ,T_{d}]]$
. Indeed, to use Artin approximation we have to equip any
$R=k[[T_{1},\ldots ,T_{d}]]$
. Indeed, to use Artin approximation we have to equip any
![]() $R$
-algebra with the log structure coming via pullback from the canonical one on
$R$
-algebra with the log structure coming via pullback from the canonical one on
![]() $(R,D)$
to extend the group
$(R,D)$
to extend the group
![]() $G^{\text{}\underline{n}}\unicode[STIX]{x1D708}_{R}(q)$
to a functor on
$G^{\text{}\underline{n}}\unicode[STIX]{x1D708}_{R}(q)$
to a functor on
![]() $R$
-algebras
$R$
-algebras
![]() $S\mapsto G^{\text{}\underline{n}}\unicode[STIX]{x1D708}_{S}(q)$
. Put
$S\mapsto G^{\text{}\underline{n}}\unicode[STIX]{x1D708}_{S}(q)$
. Put
![]() $A=k[[T_{1},\ldots ,T_{d-1}]]$
and
$A=k[[T_{1},\ldots ,T_{d-1}]]$
and
![]() $T=T_{d}$
so that
$T=T_{d}$
so that
![]() $R=A[[T]]$
. Let
$R=A[[T]]$
. Let
![]() $\unicode[STIX]{x1D6FA}_{A}^{q}(\log E)$
be the module of differential
$\unicode[STIX]{x1D6FA}_{A}^{q}(\log E)$
be the module of differential
![]() $q$
-forms on
$q$
-forms on
![]() $\text{Spec}(A)$
with logarithmic poles along
$\text{Spec}(A)$
with logarithmic poles along
![]() $E=\text{Spec}(A/(T_{1}\cdots T_{d-1}))\subset \text{Spec}\,A$
. By [Reference Kato and DennisKat82, Proposition 1], we have an isomorphism
$E=\text{Spec}(A/(T_{1}\cdots T_{d-1}))\subset \text{Spec}\,A$
. By [Reference Kato and DennisKat82, Proposition 1], we have an isomorphism
For each
![]() $n\geqslant 1$
, let
$n\geqslant 1$
, let
![]() $V_{n}\subset \unicode[STIX]{x1D6FA}_{R}^{q}(\log D)$
be the image of
$V_{n}\subset \unicode[STIX]{x1D6FA}_{R}^{q}(\log D)$
be the image of
We easily check the following.
Claim 1.2.4. For a tuple of integers
![]() $\text{}\underline{n}=(n_{1},\ldots ,n_{d-1},n)$
with
$\text{}\underline{n}=(n_{1},\ldots ,n_{d-1},n)$
with
![]() $n,n_{i}\geqslant 1$
, we have
$n,n_{i}\geqslant 1$
, we have
![]() $G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}\subset V_{n}$
and it coincides with the image of
$G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}\subset V_{n}$
and it coincides with the image of
The map (1.2.1) restricted on
![]() $V_{n}$
induces an isomorphism
$V_{n}$
induces an isomorphism
 $$\begin{eqnarray}\displaystyle & \displaystyle (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})\cdot \unicode[STIX]{x1D6FA}_{A}^{q}(\log E)\oplus (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})\cdot \unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)\xrightarrow[{}]{\cong }G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}/G^{\text{}\underline{n}^{\prime }}\unicode[STIX]{x1D6FA}_{R}^{q}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle (w,v)\rightarrow T^{n}\biggl(w+v\wedge \displaystyle \frac{dT}{T}\biggr), & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})\cdot \unicode[STIX]{x1D6FA}_{A}^{q}(\log E)\oplus (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})\cdot \unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)\xrightarrow[{}]{\cong }G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}/G^{\text{}\underline{n}^{\prime }}\unicode[STIX]{x1D6FA}_{R}^{q}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle (w,v)\rightarrow T^{n}\biggl(w+v\wedge \displaystyle \frac{dT}{T}\biggr), & \displaystyle \nonumber\end{eqnarray}$$
where
![]() $\text{}\underline{n}^{\prime }=(n_{1},\ldots ,n_{d-1},n+1)$
.
$\text{}\underline{n}^{\prime }=(n_{1},\ldots ,n_{d-1},n+1)$
.
Let
![]() $I_{q}$
be the set of strictly increasing functions
$I_{q}$
be the set of strictly increasing functions
![]() $\{1,\ldots ,q\}\rightarrow \{1,\ldots ,d-1\}$
. For
$\{1,\ldots ,q\}\rightarrow \{1,\ldots ,d-1\}$
. For
![]() $s\in I_{q}$
write
$s\in I_{q}$
write
Then
![]() $\unicode[STIX]{x1D714}_{s}$
(
$\unicode[STIX]{x1D714}_{s}$
(
![]() $s\in I_{q}$
) form a basis of
$s\in I_{q}$
) form a basis of
![]() $\unicode[STIX]{x1D6FA}_{A}^{q}(\log E)$
over
$\unicode[STIX]{x1D6FA}_{A}^{q}(\log E)$
over
![]() $A$
. Put
$A$
. Put
We have the following description of
![]() $U_{n}/U_{n+1}$
(see Part (B) of the proof of [Reference Kato and DennisKat82, Proposition 1]).
$U_{n}/U_{n+1}$
(see Part (B) of the proof of [Reference Kato and DennisKat82, Proposition 1]).
If
![]() $(p,n)=1$
, we have an isomorphism
$(p,n)=1$
, we have an isomorphism
 $$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D70C}_{n}:\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)\xrightarrow[{}]{\cong }U_{n}/U_{n+1}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \mathop{\sum }_{s\in I_{q-1}}a_{s}\unicode[STIX]{x1D714}_{s}\mapsto \mathop{\sum }_{s\in I_{q-1}}\displaystyle \frac{d(1+a_{s}T^{n})}{(1+a_{s}T^{n})}\wedge \unicode[STIX]{x1D714}_{s}\quad (a_{s}\in A). & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D70C}_{n}:\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)\xrightarrow[{}]{\cong }U_{n}/U_{n+1}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \mathop{\sum }_{s\in I_{q-1}}a_{s}\unicode[STIX]{x1D714}_{s}\mapsto \mathop{\sum }_{s\in I_{q-1}}\displaystyle \frac{d(1+a_{s}T^{n})}{(1+a_{s}T^{n})}\wedge \unicode[STIX]{x1D714}_{s}\quad (a_{s}\in A). & \displaystyle\end{eqnarray}$$
If
![]() $p|n$
, we have an isomorphism
$p|n$
, we have an isomorphism
 $$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D70C}_{n}:\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)/\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)_{d=0}\oplus \unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)/\unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)_{d=0}\xrightarrow[{}]{\cong }U_{n}/U_{n+1}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \biggl(\mathop{\sum }_{s\in I_{q-1}}a_{s}\unicode[STIX]{x1D714}_{s},\mathop{\sum }_{t\in I_{q-2}}b_{t}\unicode[STIX]{x1D714}_{t}\biggr)\mapsto \mathop{\sum }_{s\in I_{q-1}}\displaystyle \frac{d(1+a_{s}T^{n})}{(1+a_{s}T^{n})}\wedge \unicode[STIX]{x1D714}_{s}+\mathop{\sum }_{t\in I_{q-2}}\displaystyle \frac{d(1+b_{t}T^{n})}{(1+b_{t}T^{n})}\wedge \displaystyle \frac{dT}{T}\wedge \unicode[STIX]{x1D714}_{t}, & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D70C}_{n}:\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)/\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)_{d=0}\oplus \unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)/\unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)_{d=0}\xrightarrow[{}]{\cong }U_{n}/U_{n+1}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \biggl(\mathop{\sum }_{s\in I_{q-1}}a_{s}\unicode[STIX]{x1D714}_{s},\mathop{\sum }_{t\in I_{q-2}}b_{t}\unicode[STIX]{x1D714}_{t}\biggr)\mapsto \mathop{\sum }_{s\in I_{q-1}}\displaystyle \frac{d(1+a_{s}T^{n})}{(1+a_{s}T^{n})}\wedge \unicode[STIX]{x1D714}_{s}+\mathop{\sum }_{t\in I_{q-2}}\displaystyle \frac{d(1+b_{t}T^{n})}{(1+b_{t}T^{n})}\wedge \displaystyle \frac{dT}{T}\wedge \unicode[STIX]{x1D714}_{t}, & \displaystyle\end{eqnarray}$$
where
![]() $a_{s},b_{t}\in A$
.
$a_{s},b_{t}\in A$
.
Claim 1.2.5. Fix a tuple of integers
![]() $\text{}\underline{n}=(n_{1},\ldots ,n_{d-1},n)$
with
$\text{}\underline{n}=(n_{1},\ldots ,n_{d-1},n)$
with
![]() $n_{i}\geqslant 1$
.
$n_{i}\geqslant 1$
.
(1) Assume
 $(p,n)=1$
and
$(p,n)=1$
and
 $\unicode[STIX]{x1D70C}_{n}(\unicode[STIX]{x1D714})\in G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}\hspace{0.6em}{\rm mod}\hspace{0.2em}U_{n+1}$
for Then we have
$\unicode[STIX]{x1D70C}_{n}(\unicode[STIX]{x1D714})\in G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}\hspace{0.6em}{\rm mod}\hspace{0.2em}U_{n+1}$
for Then we have $$\begin{eqnarray}\unicode[STIX]{x1D714}=\underset{s\in I_{q-1}}{\sum }a_{s}\unicode[STIX]{x1D714}_{s}\in \unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D714}=\underset{s\in I_{q-1}}{\sum }a_{s}\unicode[STIX]{x1D714}_{s}\in \unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E).\end{eqnarray}$$
 $a_{s}\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})$
for all
$a_{s}\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})$
for all
 $s\in I_{q-1}$
.
$s\in I_{q-1}$
.(2) Assume
 $p|n$
and
$p|n$
and
 $\unicode[STIX]{x1D70C}_{n}(\unicode[STIX]{x1D714})\in G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}\hspace{0.6em}{\rm mod}\hspace{0.2em}U_{n+1}$
for Then one can write
$\unicode[STIX]{x1D70C}_{n}(\unicode[STIX]{x1D714})\in G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}\hspace{0.6em}{\rm mod}\hspace{0.2em}U_{n+1}$
for Then one can write $$\begin{eqnarray}\unicode[STIX]{x1D714}=(\unicode[STIX]{x1D714}_{1},\unicode[STIX]{x1D714}_{2})\in \unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)/\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)_{d=0}\oplus \unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)/\unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)_{d=0}.\end{eqnarray}$$
with
$$\begin{eqnarray}\unicode[STIX]{x1D714}=(\unicode[STIX]{x1D714}_{1},\unicode[STIX]{x1D714}_{2})\in \unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)/\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)_{d=0}\oplus \unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)/\unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)_{d=0}.\end{eqnarray}$$
with $$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D714}_{1}=\underset{s\in I_{q-1}}{\sum }a_{s}\unicode[STIX]{x1D714}_{s}\hspace{0.6em}{\rm mod}\hspace{0.2em}\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)_{d=0}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D714}_{2}=\underset{t\in I_{q-2}}{\sum }b_{t}\unicode[STIX]{x1D714}_{t}\hspace{0.6em}{\rm mod}\hspace{0.2em}\unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)_{d=0}, & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D714}_{1}=\underset{s\in I_{q-1}}{\sum }a_{s}\unicode[STIX]{x1D714}_{s}\hspace{0.6em}{\rm mod}\hspace{0.2em}\unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)_{d=0}, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D714}_{2}=\underset{t\in I_{q-2}}{\sum }b_{t}\unicode[STIX]{x1D714}_{t}\hspace{0.6em}{\rm mod}\hspace{0.2em}\unicode[STIX]{x1D6FA}_{A}^{q-2}(\log E)_{d=0}, & \displaystyle \nonumber\end{eqnarray}$$
 $a_{s},b_{t}\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})$
for all
$a_{s},b_{t}\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})$
for all
 $s\in I_{q-1}$
and
$s\in I_{q-1}$
and
 $t\in I_{q-2}$
.
$t\in I_{q-2}$
.
Proof of Claim 1.2.5.
Assume
![]() $(p,n)=1$
. From (1.2.2) we get
$(p,n)=1$
. From (1.2.2) we get
Hence (1) follows from Claim 1.2.4 noting
![]() $da_{s}\wedge \unicode[STIX]{x1D714}_{s}\in \unicode[STIX]{x1D6FA}_{A}^{q}(\log E)$
. Next assume
$da_{s}\wedge \unicode[STIX]{x1D714}_{s}\in \unicode[STIX]{x1D6FA}_{A}^{q}(\log E)$
. Next assume
![]() $p|n$
. From (1.2.3) we get
$p|n$
. From (1.2.3) we get
By Claim 1.2.4, if the left-hand side lies in
![]() $G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}\hspace{0.6em}{\rm mod}\hspace{0.2em}U_{n+1}$
, we get
$G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}\hspace{0.6em}{\rm mod}\hspace{0.2em}U_{n+1}$
, we get
Thus the desired assertion follows from the following.
Claim 1.2.6. Assume
![]() $d\unicode[STIX]{x1D702}\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})\cdot \unicode[STIX]{x1D6FA}_{A}^{q}(\log E)$
for
$d\unicode[STIX]{x1D702}\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})\cdot \unicode[STIX]{x1D6FA}_{A}^{q}(\log E)$
for
![]() $\unicode[STIX]{x1D702}=\sum _{s\in I_{q-1}}a_{s}\unicode[STIX]{x1D714}_{s}\in \unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)$
. Then there exist
$\unicode[STIX]{x1D702}=\sum _{s\in I_{q-1}}a_{s}\unicode[STIX]{x1D714}_{s}\in \unicode[STIX]{x1D6FA}_{A}^{q-1}(\log E)$
. Then there exist
![]() $\unicode[STIX]{x1D6FC}_{s}\in A$
for
$\unicode[STIX]{x1D6FC}_{s}\in A$
for
![]() $s\in I_{q-1}$
such that
$s\in I_{q-1}$
such that
![]() $a_{s}-\unicode[STIX]{x1D6FC}_{s}\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})$
for all
$a_{s}-\unicode[STIX]{x1D6FC}_{s}\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})$
for all
![]() $s$
and that
$s$
and that
![]() $d\unicode[STIX]{x1D709}=0$
for
$d\unicode[STIX]{x1D709}=0$
for
![]() $\unicode[STIX]{x1D709}=\sum _{s\in I_{q-1}}\unicode[STIX]{x1D6FC}_{s}\unicode[STIX]{x1D714}_{s}$
.
$\unicode[STIX]{x1D709}=\sum _{s\in I_{q-1}}\unicode[STIX]{x1D6FC}_{s}\unicode[STIX]{x1D714}_{s}$
.
Indeed write
![]() $a_{s}=\unicode[STIX]{x1D6FC}_{s}+a_{s}^{\prime }$
where
$a_{s}=\unicode[STIX]{x1D6FC}_{s}+a_{s}^{\prime }$
where
![]() $a_{s}^{\prime }\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})$
and
$a_{s}^{\prime }\in (T_{1}^{n_{1}}\cdots T_{d-1}^{n_{d-1}})$
and
![]() $\unicode[STIX]{x1D6FC}_{s}$
are expanded as
$\unicode[STIX]{x1D6FC}_{s}$
are expanded as
where
![]() $i_{1},\ldots ,i_{d-1}$
range over non-negative integers such that there exists
$i_{1},\ldots ,i_{d-1}$
range over non-negative integers such that there exists
![]() $1\leqslant \unicode[STIX]{x1D708}\leqslant d-1$
with
$1\leqslant \unicode[STIX]{x1D708}\leqslant d-1$
with
![]() $i_{\unicode[STIX]{x1D708}}<n_{\unicode[STIX]{x1D708}}$
. Then one easily check that
$i_{\unicode[STIX]{x1D708}}<n_{\unicode[STIX]{x1D708}}$
. Then one easily check that
![]() $\unicode[STIX]{x1D6FC}_{s}$
satisfy the desired condition.◻
$\unicode[STIX]{x1D6FC}_{s}$
satisfy the desired condition.◻
Now we can finish the proof of Proposition 1.2.3. In the following we fix a tuple of integers
![]() $\text{}\underline{n}=(n_{1},\ldots ,n_{d-1},n_{d})$
with
$\text{}\underline{n}=(n_{1},\ldots ,n_{d-1},n_{d})$
with
![]() $n_{i}\geqslant 1$
and take
$n_{i}\geqslant 1$
and take
![]() $\unicode[STIX]{x1D714}\in G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}$
. By Claim 1.2.5 there exist a series of elements
$\unicode[STIX]{x1D714}\in G^{\text{}\underline{n}}\unicode[STIX]{x1D6FA}_{R}^{q}$
. By Claim 1.2.5 there exist a series of elements
such that
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D714} & = & \displaystyle \underset{n\geqslant n_{d}}{\sum }\;\underset{s\in I_{q-1}}{\sum }\displaystyle \frac{d(1+a_{s,n}T^{n})}{(1+a_{s,n}T^{n})}\wedge \unicode[STIX]{x1D714}_{s}+\underset{pm\geqslant n_{d}}{\sum }\;\underset{t\in I_{q-2}}{\sum }\displaystyle \frac{d(1+b_{t,m}T^{pm})}{(1+b_{t,m}T^{pm})}\wedge \displaystyle \frac{dT}{T}\wedge \unicode[STIX]{x1D714}_{t}\nonumber\\ \displaystyle & = & \displaystyle \underset{s\in I_{q-1}}{\sum }\bigg(\underset{n\geqslant n_{d}}{\sum }\displaystyle \frac{d(1+a_{s,n}T^{n})}{(1+a_{s,n}T^{n})}\bigg)\wedge \unicode[STIX]{x1D714}_{s}+\underset{t\in I_{q-2}}{\sum }\bigg(\underset{pm\geqslant n_{d}}{\sum }\displaystyle \frac{d(1+b_{t,m}T^{pm})}{(1+b_{t,m}T^{pm})}\bigg)\wedge \displaystyle \frac{dT}{T}\wedge \unicode[STIX]{x1D714}_{t}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D714} & = & \displaystyle \underset{n\geqslant n_{d}}{\sum }\;\underset{s\in I_{q-1}}{\sum }\displaystyle \frac{d(1+a_{s,n}T^{n})}{(1+a_{s,n}T^{n})}\wedge \unicode[STIX]{x1D714}_{s}+\underset{pm\geqslant n_{d}}{\sum }\;\underset{t\in I_{q-2}}{\sum }\displaystyle \frac{d(1+b_{t,m}T^{pm})}{(1+b_{t,m}T^{pm})}\wedge \displaystyle \frac{dT}{T}\wedge \unicode[STIX]{x1D714}_{t}\nonumber\\ \displaystyle & = & \displaystyle \underset{s\in I_{q-1}}{\sum }\bigg(\underset{n\geqslant n_{d}}{\sum }\displaystyle \frac{d(1+a_{s,n}T^{n})}{(1+a_{s,n}T^{n})}\bigg)\wedge \unicode[STIX]{x1D714}_{s}+\underset{t\in I_{q-2}}{\sum }\bigg(\underset{pm\geqslant n_{d}}{\sum }\displaystyle \frac{d(1+b_{t,m}T^{pm})}{(1+b_{t,m}T^{pm})}\bigg)\wedge \displaystyle \frac{dT}{T}\wedge \unicode[STIX]{x1D714}_{t}.\nonumber\end{eqnarray}$$
The products
converge in
![]() $1+(T_{1}^{n_{1}}\cdots T_{d}^{n_{d}})\subset R^{\times }$
and we get
$1+(T_{1}^{n_{1}}\cdots T_{d}^{n_{d}})\subset R^{\times }$
and we get
This completes the proof of Proposition 1.2.3. ◻
Remark 1.2.7. In fact, the above proof shows that the exactness in the middle of the complex in Theorem 1.2.1 already holds in the Nisnevich topology.
2 Filtered de Rham–Witt complexes
Let
![]() $X,D,j::U{\hookrightarrow}X$
be as before. Let
$X,D,j::U{\hookrightarrow}X$
be as before. Let
![]() $\{D_{\unicode[STIX]{x1D706}}\}_{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}$
be the (smooth) irreducible components of
$\{D_{\unicode[STIX]{x1D706}}\}_{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}$
be the (smooth) irreducible components of
![]() $D$
. We endow
$D$
. We endow
![]() $\mathbb{Z}^{\unicode[STIX]{x1D6EC}}$
with a semi-order by
$\mathbb{Z}^{\unicode[STIX]{x1D6EC}}$
with a semi-order by
For
![]() $\text{}\underline{n}=(n_{\unicode[STIX]{x1D706}})_{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}\in \mathbb{Z}^{\unicode[STIX]{x1D6EC}}$
let
$\text{}\underline{n}=(n_{\unicode[STIX]{x1D706}})_{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}\in \mathbb{Z}^{\unicode[STIX]{x1D6EC}}$
let
be the associated divisor.
2.1 Definition and basic properties
Let
![]() $E$
be a Cartier divisor on
$E$
be a Cartier divisor on
![]() $X$
. It is given by
$X$
. It is given by
![]() $\{V_{i},f_{i}\}$
, where
$\{V_{i},f_{i}\}$
, where
![]() $\{V_{i}\}_{i}$
is an open cover of
$\{V_{i}\}_{i}$
is an open cover of
![]() $X$
and
$X$
and
![]() $f_{i}\in \unicode[STIX]{x1D6E4}(V_{i},{\mathcal{M}}_{X}^{\times })$
is a section of the sheaf of total fractional ring.
$f_{i}\in \unicode[STIX]{x1D6E4}(V_{i},{\mathcal{M}}_{X}^{\times })$
is a section of the sheaf of total fractional ring.
Definition 2.1.1. We define an invertible
![]() $W_{m}{\mathcal{O}}_{X}$
-module
$W_{m}{\mathcal{O}}_{X}$
-module
![]() $W_{m}{\mathcal{O}}_{X}(E)$
associated to
$W_{m}{\mathcal{O}}_{X}(E)$
associated to
![]() $E$
as
$E$
as
where
![]() $[\cdot ]_{m}:{\mathcal{O}}\rightarrow W_{m}{\mathcal{O}}$
the Teichmüller lifting.
$[\cdot ]_{m}:{\mathcal{O}}\rightarrow W_{m}{\mathcal{O}}$
the Teichmüller lifting.
This definition gives us an invertible sheaf
![]() $W_{m}{\mathcal{O}}_{X}(D_{\text{}\underline{n}})$
for any
$W_{m}{\mathcal{O}}_{X}(D_{\text{}\underline{n}})$
for any
![]() $D_{\text{}\underline{n}}$
as above.
$D_{\text{}\underline{n}}$
as above.
Lemma 2.1.2. We have:
(i)
 $F(W_{m+1}{\mathcal{O}}_{X}(D_{\text{}\underline{n}}))\subset W_{m}{\mathcal{O}}_{X}(D_{p\text{}\underline{n}})$
;
$F(W_{m+1}{\mathcal{O}}_{X}(D_{\text{}\underline{n}}))\subset W_{m}{\mathcal{O}}_{X}(D_{p\text{}\underline{n}})$
;(ii)
 $V(W_{m}{\mathcal{O}}_{X}(D_{p\text{}\underline{n}}))\subset W_{m+1}{\mathcal{O}}_{X}(D_{\text{}\underline{n}})$
;
$V(W_{m}{\mathcal{O}}_{X}(D_{p\text{}\underline{n}}))\subset W_{m+1}{\mathcal{O}}_{X}(D_{\text{}\underline{n}})$
;(iii)
 $R(W_{m+1}{\mathcal{O}}_{X}(D_{\text{}\underline{n}}))\subset W_{m}{\mathcal{O}}_{X}(D_{\text{}\underline{n}})$
.
$R(W_{m+1}{\mathcal{O}}_{X}(D_{\text{}\underline{n}}))\subset W_{m}{\mathcal{O}}_{X}(D_{\text{}\underline{n}})$
.
Proof. The claims (i) and (iii) are clear by the definition. Claim (ii) follows from the equalities
![]() $V(x\cdot Fy)=V(x)\cdot y$
and
$V(x\cdot Fy)=V(x)\cdot y$
and
![]() $F[y]_{m+1}=[y^{p}]_{m}$
.◻
$F[y]_{m+1}=[y^{p}]_{m}$
.◻
Let
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X}^{\ast }(\log D)$
be the de Rham–Witt complex with respect to the canonical log structure
$W_{m}\unicode[STIX]{x1D6FA}_{X}^{\ast }(\log D)$
be the de Rham–Witt complex with respect to the canonical log structure
![]() $(X,j_{\ast }{\mathcal{O}}_{U}^{\times }\cap {\mathcal{O}}_{X})$
defined in [Reference Hyodo and KatoHK94, § 4].
$(X,j_{\ast }{\mathcal{O}}_{U}^{\times }\cap {\mathcal{O}}_{X})$
defined in [Reference Hyodo and KatoHK94, § 4].
Definition 2.1.3. For
![]() $\text{}\underline{n}=(n_{\unicode[STIX]{x1D706}})_{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}\in \mathbb{Z}^{\unicode[STIX]{x1D6EC}}$
, we define the filtered de Rham–Witt complex as
$\text{}\underline{n}=(n_{\unicode[STIX]{x1D706}})_{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}\in \mathbb{Z}^{\unicode[STIX]{x1D6EC}}$
, we define the filtered de Rham–Witt complex as
where
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X}^{\ast }(\log D)$
is canonically viewed as a subsheaf of
$W_{m}\unicode[STIX]{x1D6FA}_{X}^{\ast }(\log D)$
is canonically viewed as a subsheaf of
![]() $j_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U}^{\ast }$
(cf. [Reference Hyodo and KatoHK94, (4.20)]).
$j_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U}^{\ast }$
(cf. [Reference Hyodo and KatoHK94, (4.20)]).
Note that
In particular,
![]() $W_{1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast }=\unicode[STIX]{x1D6FA}_{X}^{\ast }(\log D)\otimes {\mathcal{O}}_{X}(-D_{\text{}\underline{n}})=\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast }$
(cf. notation in Theorem 1.2.1).
$W_{1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast }=\unicode[STIX]{x1D6FA}_{X}^{\ast }(\log D)\otimes {\mathcal{O}}_{X}(-D_{\text{}\underline{n}})=\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast }$
(cf. notation in Theorem 1.2.1).
Lemma 2.1.4. We have the following inclusions:
(i)
 $F(W_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast })\subset W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{\ast }$
;
$F(W_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast })\subset W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{\ast }$
;(ii)
 $V(W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{\ast })\subset W_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast }$
;
$V(W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{\ast })\subset W_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast }$
;(iii)
 $R(W_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast })\subset W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast }$
.
$R(W_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast })\subset W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{\ast }$
.
Proof. This follows from Lemma 2.1.2 and the basic properties of de Rham–Witt complex [Reference Hyodo and KatoHK94, § 4.1] [Reference LorenzonLor02, Proposition 1.5]. ◻
2.2 Canonical filtration
On
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X}^{\ast }(\log D)$
, we can define the canonical filtration as in [Reference IllusieIll79, I (3.1.1)]:
$W_{m}\unicode[STIX]{x1D6FA}_{X}^{\ast }(\log D)$
, we can define the canonical filtration as in [Reference IllusieIll79, I (3.1.1)]:
 $$\begin{eqnarray}\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D):=\left\{\begin{array}{@{}ll@{}}W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)\quad & \text{if}\;s\leqslant 0\;\text{or}\;r\leqslant 0,\\ \text{Ker}(R^{m-s}:W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)\rightarrow W_{s}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D))\quad & \text{if}~1\leqslant s\leqslant m,\\ 0\quad & \text{if}~s\geqslant m.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D):=\left\{\begin{array}{@{}ll@{}}W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)\quad & \text{if}\;s\leqslant 0\;\text{or}\;r\leqslant 0,\\ \text{Ker}(R^{m-s}:W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)\rightarrow W_{s}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D))\quad & \text{if}~1\leqslant s\leqslant m,\\ 0\quad & \text{if}~s\geqslant m.\end{array}\right.\end{eqnarray}$$
For
![]() $1\leqslant s\leqslant m$
, we have [Reference LorenzonLor02, Proposition 1.16]
$1\leqslant s\leqslant m$
, we have [Reference LorenzonLor02, Proposition 1.16]
Definition 2.2.1. For
![]() $1\leqslant s\leqslant m$
, we define
$1\leqslant s\leqslant m$
, we define
 $$\begin{eqnarray}\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}:=\left\{\begin{array}{@{}ll@{}}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}\quad & \text{if}\;s\leqslant 0\;\text{or}\;r\leqslant 0,\\ \text{Ker}(R^{m-s}:W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}\rightarrow W_{s}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r})\quad & \text{if}~1\leqslant s\leqslant m,\\ 0\quad & \text{if}~s\geqslant m.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}:=\left\{\begin{array}{@{}ll@{}}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}\quad & \text{if}\;s\leqslant 0\;\text{or}\;r\leqslant 0,\\ \text{Ker}(R^{m-s}:W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}\rightarrow W_{s}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r})\quad & \text{if}~1\leqslant s\leqslant m,\\ 0\quad & \text{if}~s\geqslant m.\end{array}\right.\end{eqnarray}$$
Theorem 2.2.2. We have
Proof. We only need to show the inclusion ‘
![]() $\subseteq$
’. By the definition of the canonical filtration and the fact that
$\subseteq$
’. By the definition of the canonical filtration and the fact that
![]() $W_{m}{\mathcal{O}}_{X}(-D_{\text{}\underline{n}})$
is an invertible sheaf, we have
$W_{m}{\mathcal{O}}_{X}(-D_{\text{}\underline{n}})$
is an invertible sheaf, we have
and it suffices to show that the group on the right-hand side is contained in
Using the formula
![]() $x\cdot Vy=V(F(x)\cdot y)$
repeatedly, we see that for any
$x\cdot Vy=V(F(x)\cdot y)$
repeatedly, we see that for any
![]() $\unicode[STIX]{x1D714}\in W_{m-s}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)$
,
$\unicode[STIX]{x1D714}\in W_{m-s}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)$
,
![]() $\unicode[STIX]{x1D714}^{\prime }\in W_{m-s}\unicode[STIX]{x1D6FA}_{X}^{r-1}(\log D)$
and
$\unicode[STIX]{x1D714}^{\prime }\in W_{m-s}\unicode[STIX]{x1D6FA}_{X}^{r-1}(\log D)$
and
![]() $x\in W_{m}{\mathcal{O}}_{X}(-D_{\text{}\underline{n}})$
,
$x\in W_{m}{\mathcal{O}}_{X}(-D_{\text{}\underline{n}})$
,
By our definition, we have
![]() $F^{s}(x)\cdot \unicode[STIX]{x1D714}\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r}$
and
$F^{s}(x)\cdot \unicode[STIX]{x1D714}\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r}$
and
![]() $F^{s}(x)\cdot \unicode[STIX]{x1D714}^{\prime }\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r-1}$
. It suffices to prove that
$F^{s}(x)\cdot \unicode[STIX]{x1D714}^{\prime }\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r-1}$
. It suffices to prove that
![]() $dx\cdot V^{s}(\unicode[STIX]{x1D714}^{\prime })\in V^{s}W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r}$
. Since the problem is local on
$dx\cdot V^{s}(\unicode[STIX]{x1D714}^{\prime })\in V^{s}W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r}$
. Since the problem is local on
![]() $X$
, it is enough to show the following claim.
$X$
, it is enough to show the following claim.
Claim 2.2.3. For any
![]() $t\in {\mathcal{O}}_{X}$
, and
$t\in {\mathcal{O}}_{X}$
, and
![]() $z^{\prime }\in W_{m-s}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)$
,
$z^{\prime }\in W_{m-s}\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)$
,
Indeed, we know (cf. [Reference IllusieIll79, I, Proposition 1.5.2]),
Using this formula and
![]() $x\cdot Vy=V(F(x)\cdot y)$
repeatedly, we get the claim.◻
$x\cdot Vy=V(F(x)\cdot y)$
repeatedly, we get the claim.◻
Corollary 2.2.4. There are the following inclusions:
(i)
 $F(\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r})\subset \text{Fil}^{s-1}W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}$
;
$F(\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r})\subset \text{Fil}^{s-1}W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}$
;(ii)
 $V(\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r})\subset \text{Fil}^{s+1}W_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
;
$V(\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r})\subset \text{Fil}^{s+1}W_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
;(iii)
 $R(\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r})\subset \text{Fil}^{s}W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
.
$R(\text{Fil}^{s}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r})\subset \text{Fil}^{s}W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
.
Proof. This follows from Lemma 2.1.4,
![]() $FV=p=VF$
and
$FV=p=VF$
and
![]() $FdV=d$
.◻
$FdV=d$
.◻
For
![]() $\text{}\underline{n}\geqslant \text{}\underline{1}$
, i.e.
$\text{}\underline{n}\geqslant \text{}\underline{1}$
, i.e.
![]() $\text{}\underline{n}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
, we have
$\text{}\underline{n}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
, we have
Indeed, for
![]() $m=1$
this follows from the fact
$m=1$
this follows from the fact
![]() $W_{1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}=\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)(-D_{\text{}\underline{n}})\subset \unicode[STIX]{x1D6FA}_{X}^{r}$
. Then the claim follows by induction on
$W_{1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}=\unicode[STIX]{x1D6FA}_{X}^{r}(\log D)(-D_{\text{}\underline{n}})\subset \unicode[STIX]{x1D6FA}_{X}^{r}$
. Then the claim follows by induction on
![]() $m$
using Theorem 2.2.2.
$m$
using Theorem 2.2.2.
Lemma 2.2.5. For
![]() $\text{}\underline{n}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
, we have
$\text{}\underline{n}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
, we have
and
In particular the multiplication by
![]() $p^{s}$
induces an injective homomorphism
$p^{s}$
induces an injective homomorphism
Proof. The first equality follows from the following commutative diagram.

The second equality follows from the first and the fact (cf. [Reference IllusieIll79, I, Proposition 3.11])
Recall (cf. the proof of [Reference IllusieIll79, I, Proposition 3.11, p. 575])
We have the following analogues for the filtered de Rham–Witt sheaves.
Proposition 2.2.6. For
![]() $\text{}\underline{n}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
, we have:
$\text{}\underline{n}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
, we have:
(i)
 $\text{Ker}(F^{m-1}:W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}\rightarrow \unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}}}^{r})=VW_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}$
, i.e.,
$\text{Ker}(F^{m-1}:W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}\rightarrow \unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}}}^{r})=VW_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}$
, i.e.,  $$\begin{eqnarray}VW_{m-1}\unicode[STIX]{x1D6FA}_{X}^{r}\cap W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}=VW_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r};\end{eqnarray}$$
$$\begin{eqnarray}VW_{m-1}\unicode[STIX]{x1D6FA}_{X}^{r}\cap W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}=VW_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r};\end{eqnarray}$$
(ii)
 $\text{Ker}(F^{m-1}d:W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}\rightarrow \unicode[STIX]{x1D6FA}_{X|D_{p^{m}\text{}\underline{n}}}^{r+1})=FW_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
, i.e.,
$\text{Ker}(F^{m-1}d:W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}\rightarrow \unicode[STIX]{x1D6FA}_{X|D_{p^{m}\text{}\underline{n}}}^{r+1})=FW_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
, i.e.,  $$\begin{eqnarray}FW_{m+1}\unicode[STIX]{x1D6FA}_{X}^{r}\cap W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}=FW_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}.\end{eqnarray}$$
$$\begin{eqnarray}FW_{m+1}\unicode[STIX]{x1D6FA}_{X}^{r}\cap W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}=FW_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}.\end{eqnarray}$$
Proof. This is proved by the same argument as in the proof of [Reference IllusieIll79, I, Proposition 3.11], which we recall below.
(i) For
![]() $m=1$
it is trivial. For
$m=1$
it is trivial. For
![]() $m>1$
we have
$m>1$
we have
by Theorem 2.2.2 and Lemma 2.2.5. It suffices to show that, for
![]() $1\leqslant s\leqslant m$
,
$1\leqslant s\leqslant m$
,
Let
![]() $z=Vx+dV^{s}y$
with
$z=Vx+dV^{s}y$
with
![]() $x\in W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}$
,
$x\in W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}$
,
![]() $y\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r-1}$
be such that
$y\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r-1}$
be such that
![]() $F^{m-1}z=0$
. Noting
$F^{m-1}z=0$
. Noting
![]() $F^{m-1}Vx=pF^{m-2}x=0$
and
$F^{m-1}Vx=pF^{m-2}x=0$
and
![]() $F^{m-1}dV^{s}=F^{m-1-s}d$
, it follows that
$F^{m-1}dV^{s}=F^{m-1-s}d$
, it follows that
![]() $F^{m-1-s}dy=0$
. Let
$F^{m-1-s}dy=0$
. Let
![]() $\overline{y}$
be the image of
$\overline{y}$
be the image of
![]() $y$
in
$y$
in
![]() $\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r-1}$
under the restriction map
$\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r-1}$
under the restriction map
![]() $R^{m-1-s}$
. Then, by [Reference IllusieIll79, I, Proposition 3.3], we get
$R^{m-1-s}$
. Then, by [Reference IllusieIll79, I, Proposition 3.3], we get
![]() $C^{-(m-1-s)}d\overline{y}=0$
and
$C^{-(m-1-s)}d\overline{y}=0$
and
![]() $d\overline{y}=0$
in
$d\overline{y}=0$
in
![]() $\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r}$
. By Lemma 1.1.8 there exists (locally)
$\unicode[STIX]{x1D6FA}_{X|D_{p^{s}\text{}\underline{n}}}^{r}$
. By Lemma 1.1.8 there exists (locally)
![]() $y^{\prime }\in \unicode[STIX]{x1D6FA}_{X|D_{p^{s-1}\text{}\underline{n}}}^{r-1}$
such that
$y^{\prime }\in \unicode[STIX]{x1D6FA}_{X|D_{p^{s-1}\text{}\underline{n}}}^{r-1}$
such that
![]() $\overline{y}=C^{-1}(y^{\prime })$
. We can then take a lift
$\overline{y}=C^{-1}(y^{\prime })$
. We can then take a lift
![]() ${\tilde{y}}$
of
${\tilde{y}}$
of
![]() $y^{\prime }$
in
$y^{\prime }$
in
![]() $W_{m+1-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s-1}\text{}\underline{n}}}^{r-1}$
. Indeed, writing
$W_{m+1-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s-1}\text{}\underline{n}}}^{r-1}$
. Indeed, writing
![]() $y^{\prime }=\sum _{\unicode[STIX]{x1D6FC}}a_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D714}_{\unicode[STIX]{x1D6FC}}$
with
$y^{\prime }=\sum _{\unicode[STIX]{x1D6FC}}a_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D714}_{\unicode[STIX]{x1D6FC}}$
with
![]() $a_{\unicode[STIX]{x1D6FC}}\in {\mathcal{O}}_{X}(-D_{p^{s-1}\text{}\underline{n}})$
and
$a_{\unicode[STIX]{x1D6FC}}\in {\mathcal{O}}_{X}(-D_{p^{s-1}\text{}\underline{n}})$
and
![]() $\unicode[STIX]{x1D714}_{\unicode[STIX]{x1D6FC}}\in \unicode[STIX]{x1D6FA}_{X}^{r-1}(\log D)$
, we take
$\unicode[STIX]{x1D714}_{\unicode[STIX]{x1D6FC}}\in \unicode[STIX]{x1D6FA}_{X}^{r-1}(\log D)$
, we take
![]() ${\tilde{y}}=\sum _{\unicode[STIX]{x1D6FC}}[a_{\unicode[STIX]{x1D6FC}}]_{m+1-s}\tilde{\unicode[STIX]{x1D714}}_{\unicode[STIX]{x1D6FC}}$
, where
${\tilde{y}}=\sum _{\unicode[STIX]{x1D6FC}}[a_{\unicode[STIX]{x1D6FC}}]_{m+1-s}\tilde{\unicode[STIX]{x1D714}}_{\unicode[STIX]{x1D6FC}}$
, where
![]() $\tilde{\unicode[STIX]{x1D714}}_{\unicode[STIX]{x1D6FC}}\in W_{m+1-s}\unicode[STIX]{x1D6FA}_{X}^{r-1}(\log D)$
is a lift of
$\tilde{\unicode[STIX]{x1D714}}_{\unicode[STIX]{x1D6FC}}\in W_{m+1-s}\unicode[STIX]{x1D6FA}_{X}^{r-1}(\log D)$
is a lift of
![]() $\unicode[STIX]{x1D714}_{\unicode[STIX]{x1D6FC}}$
. By the construction we have
$\unicode[STIX]{x1D714}_{\unicode[STIX]{x1D6FC}}$
. By the construction we have
By taking
![]() $V^{s}$
on both sides, we get
$V^{s}$
on both sides, we get
Hence
That is
Hence
![]() $z=Vx+dV^{s}y\in VW_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}+\text{Fil}^{s+1}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
, which proves (2.2.5).
$z=Vx+dV^{s}y\in VW_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}+\text{Fil}^{s+1}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
, which proves (2.2.5).
(ii) It suffices to prove that, for
![]() $1\leqslant s\leqslant m$
,
$1\leqslant s\leqslant m$
,
Let
![]() $z=V^{s}x+dV^{s}y$
with
$z=V^{s}x+dV^{s}y$
with
![]() $x\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s+1}\text{}\underline{n}}}^{r}$
,
$x\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s+1}\text{}\underline{n}}}^{r}$
,
![]() $y\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s+1}\text{}\underline{n}}}^{r-1}$
be such that
$y\in W_{m-s}\unicode[STIX]{x1D6FA}_{X|D_{p^{s+1}\text{}\underline{n}}}^{r-1}$
be such that
![]() $F^{m-1}dz=0$
. Noting
$F^{m-1}dz=0$
. Noting
![]() $F^{m-1}dV^{s}=F^{m-1-s}d$
, it follows that
$F^{m-1}dV^{s}=F^{m-1-s}d$
, it follows that
![]() $F^{m-1-s}dx=0$
. Let
$F^{m-1-s}dx=0$
. Let
![]() $\overline{x}$
be the image of
$\overline{x}$
be the image of
![]() $x$
in
$x$
in
![]() $\unicode[STIX]{x1D6FA}_{X|D_{p^{s+1}\text{}\underline{n}}}^{r}$
. As in (i), there exist
$\unicode[STIX]{x1D6FA}_{X|D_{p^{s+1}\text{}\underline{n}}}^{r}$
. As in (i), there exist
![]() $\tilde{x}\in W_{m-s+1}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}n}}^{r}$
, such that
$\tilde{x}\in W_{m-s+1}\unicode[STIX]{x1D6FA}_{X|D_{p^{s}n}}^{r}$
, such that
By taking
![]() $V^{s}$
on both sides, we obtain
$V^{s}$
on both sides, we obtain
Noting that
![]() $dV^{s}y=FdV^{s+1}y\in FW_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
, we obtain the inclusion (2.2.6).◻
$dV^{s}y=FdV^{s+1}y\in FW_{m+1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
, we obtain the inclusion (2.2.6).◻
Corollary 2.2.7. For
![]() $\text{}\underline{n}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
and
$\text{}\underline{n}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
and
![]() $x\in W_{m-1}\unicode[STIX]{x1D6FA}_{X}^{r}$
,
$x\in W_{m-1}\unicode[STIX]{x1D6FA}_{X}^{r}$
,
![]() $\text{}\underline{p}\cdot x\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
(cf. (2.2.2)) implies
$\text{}\underline{p}\cdot x\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
(cf. (2.2.2)) implies
![]() $x\in W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
.
$x\in W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
.
Proof. Recall we have the following diagram [Reference IllusieIll79, Proposition 3.4].

Hence there exists
![]() $\tilde{x}\in W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}$
such that
$\tilde{x}\in W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}$
such that
![]() $p\tilde{x}=\text{}\underline{p}\cdot x$
and
$p\tilde{x}=\text{}\underline{p}\cdot x$
and
![]() $R\tilde{x}=x$
. By the assumption, we have
$R\tilde{x}=x$
. By the assumption, we have
![]() $VF\tilde{x}=p\tilde{x}=\text{}\underline{p}\cdot x\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
. Thanks to Corollary 2.2.6(i), it follows that there exists
$VF\tilde{x}=p\tilde{x}=\text{}\underline{p}\cdot x\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
. Thanks to Corollary 2.2.6(i), it follows that there exists
![]() $y^{\prime }\in W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}$
such that
$y^{\prime }\in W_{m-1}\unicode[STIX]{x1D6FA}_{X|D_{p\text{}\underline{n}}}^{r}$
such that
Recall the identity in [Reference IllusieIll79, I. 3.21.1.4]:
Therefore there exists
![]() $z^{\prime }\in \unicode[STIX]{x1D6FA}_{X}^{r-1}$
such that
$z^{\prime }\in \unicode[STIX]{x1D6FA}_{X}^{r-1}$
such that
![]() $F\tilde{x}-y^{\prime }=FdV^{m-1}z^{\prime }$
. That is
$F\tilde{x}-y^{\prime }=FdV^{m-1}z^{\prime }$
. That is
Corollary 2.2.6(ii) implies that there exists
![]() $y^{\prime \prime }\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
such that
$y^{\prime \prime }\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
such that
Thanks to the identity [Reference IllusieIll79, I. 3.21.1.2]
we find
![]() $z^{\prime \prime }\in \unicode[STIX]{x1D6FA}_{X}^{r}$
such that
$z^{\prime \prime }\in \unicode[STIX]{x1D6FA}_{X}^{r}$
such that
Noting that
![]() $\text{Ker}(R:W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}\rightarrow W_{m-1}\unicode[STIX]{x1D6FA}_{X}^{r})=V^{m-1}\unicode[STIX]{x1D6FA}_{X}^{r}+dV^{m-1}\unicode[STIX]{x1D6FA}_{X}^{r-1}$
, we get
$\text{Ker}(R:W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}\rightarrow W_{m-1}\unicode[STIX]{x1D6FA}_{X}^{r})=V^{m-1}\unicode[STIX]{x1D6FA}_{X}^{r}+dV^{m-1}\unicode[STIX]{x1D6FA}_{X}^{r-1}$
, we get
2.3 Logarithmic part of filtered de Rham–Witt complexes
The relation between the filtered de Rham–Witt sheaves and the relative logarithmic de Rham–Witt sheaves is given by the following theorem, which is a generalization of Theorem 1.2.1.
We first introduce some notations. Let
be the set of effective divisors with supports in
![]() $X-U$
, whose irreducible components are same as those of
$X-U$
, whose irreducible components are same as those of
![]() $D$
. The semi-order on
$D$
. The semi-order on
![]() $\mathbb{Z}^{\unicode[STIX]{x1D6EC}}$
defined in (2.0.1) induces a semi-order on
$\mathbb{Z}^{\unicode[STIX]{x1D6EC}}$
defined in (2.0.1) induces a semi-order on
![]() $\unicode[STIX]{x1D6F4}$
:
$\unicode[STIX]{x1D6F4}$
:
For
![]() $D_{1},D_{2}\in \unicode[STIX]{x1D6F4}$
with
$D_{1},D_{2}\in \unicode[STIX]{x1D6F4}$
with
![]() $D_{1}\geqslant D_{2}$
, we have a natural injective map
$D_{1}\geqslant D_{2}$
, we have a natural injective map
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D_{1},\log }^{r}{\hookrightarrow}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{2},\log }^{r}$
(see Corollary 1.1.4), which gives a pro-system of sheaves
$W_{m}\unicode[STIX]{x1D6FA}_{X|D_{1},\log }^{r}{\hookrightarrow}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{2},\log }^{r}$
(see Corollary 1.1.4), which gives a pro-system of sheaves
In order to simplify the notation, we simply write it as “
![]() $\mathop{\varprojlim }\nolimits_{D}$
”
$\mathop{\varprojlim }\nolimits_{D}$
”
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
.
$W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
.
Theorem 2.3.1. We have the following exact sequence of pro-sheaves,
where
![]() $D$
runs over the set
$D$
runs over the set
![]() $\unicode[STIX]{x1D6F4}$
.
$\unicode[STIX]{x1D6F4}$
.
We need the following lemma, which follows from easy calculations with Witt vectors.
Lemma 2.3.2 [Reference Geisser and HesselholtGH06, Lemma 1.2.3].
Let
![]() $R$
be any ring, and
$R$
be any ring, and
![]() $t\in R$
, then
$t\in R$
, then
![]() $[1+t]_{m}-[1]_{m}=(y_{0},\ldots ,y_{m-1})$
with
$[1+t]_{m}-[1]_{m}=(y_{0},\ldots ,y_{m-1})$
with
![]() $y_{i}\equiv t$
mod
$y_{i}\equiv t$
mod
![]() $t^{2}R$
for
$t^{2}R$
for
![]() $0\leqslant i\leqslant m-1$
. Here
$0\leqslant i\leqslant m-1$
. Here
![]() $[x]_{m}=(x,0,\ldots ,0)\in W_{m}(R)$
is the Teichmüller representative of
$[x]_{m}=(x,0,\ldots ,0)\in W_{m}(R)$
is the Teichmüller representative of
![]() $x\in R$
.
$x\in R$
.
Proof of Theorem 2.3.1.
First we show that
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}},\log }^{r}\subset j_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
(cf. Definition 1.1.1) lies in
$W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}},\log }^{r}\subset j_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
(cf. Definition 1.1.1) lies in
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
. This is a local question so that we may assume that
$W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
. This is a local question so that we may assume that
![]() $X=\text{Spec}(A)$
and
$X=\text{Spec}(A)$
and
![]() $D=(t)$
for some
$D=(t)$
for some
![]() $t\in A$
. By Lemma 2.3.2 we can write
$t\in A$
. By Lemma 2.3.2 we can write
with
![]() $y_{i}\in A$
for
$y_{i}\in A$
for
![]() $0\leqslant i\leqslant m-1$
. Noting
$0\leqslant i\leqslant m-1$
. Noting
![]() $dx=0$
for
$dx=0$
for
![]() $x\in W_{m}(\mathbb{F}_{p})$
, we get
$x\in W_{m}(\mathbb{F}_{p})$
, we get
with
![]() $c_{i}\in A$
for
$c_{i}\in A$
for
![]() $0\leqslant i\leqslant m-1$
, where the second equality follows from the formula
$0\leqslant i\leqslant m-1$
, where the second equality follows from the formula
Hence we get
noting
![]() $[1+t^{p^{m-1}n}]_{m}$
is a unit of
$[1+t^{p^{m-1}n}]_{m}$
is a unit of
![]() $W_{m}{\mathcal{O}}_{X}$
.
$W_{m}{\mathcal{O}}_{X}$
.
The surjectivity of
![]() $1-F$
as pro-systems follows by the same argument as in the proof of [Reference IllusieIll79, I, Proposition 3.26]. Indeed, the formula
$1-F$
as pro-systems follows by the same argument as in the proof of [Reference IllusieIll79, I, Proposition 3.26]. Indeed, the formula
![]() $dx=(F-1)(dVx+dV^{2}x+\cdots +dV^{m-1}x)$
implies that
$dx=(F-1)(dVx+dV^{2}x+\cdots +dV^{m-1}x)$
implies that
Therefore it is enough to show that
is surjective.
Theorem 2.2.2 implies that
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D_{n}}^{r}/dW_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r-1}$
is generated by sections
$W_{m}\unicode[STIX]{x1D6FA}_{X|D_{n}}^{r}/dW_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r-1}$
is generated by sections
where
![]() $x\in {\mathcal{O}}_{X}(-D_{p^{i}\text{}\underline{n}^{\prime }})$
for some
$x\in {\mathcal{O}}_{X}(-D_{p^{i}\text{}\underline{n}^{\prime }})$
for some
![]() $\text{}\underline{n}^{\prime }\leqslant \text{}\underline{n}$
and
$\text{}\underline{n}^{\prime }\leqslant \text{}\underline{n}$
and
![]() $y_{j}\in {\mathcal{O}}_{X}^{\times }$
for
$y_{j}\in {\mathcal{O}}_{X}^{\times }$
for
![]() $1\leqslant j\leqslant r$
such that
$1\leqslant j\leqslant r$
such that
(Note that in view of (2.3.1),
![]() $d\log [y_{i}]_{m}$
may also contribute to the multiplicity.) We may then choose (étale locally)
$d\log [y_{i}]_{m}$
may also contribute to the multiplicity.) We may then choose (étale locally)
![]() $y\in {\mathcal{O}}_{X}(-D_{p^{i}\text{}\underline{n}^{\prime }})$
such that
$y\in {\mathcal{O}}_{X}(-D_{p^{i}\text{}\underline{n}^{\prime }})$
such that
![]() $y^{p}-y=x$
. Then we have
$y^{p}-y=x$
. Then we have
which implies the desired surjectivity.
Finally we show the exactness in the middle. It suffices to show the following equality in
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}$
:
$W_{m}\unicode[STIX]{x1D6FA}_{X}^{r}$
:
We prove this by induction on
![]() $m$
. For
$m$
. For
![]() $m=1$
, this is Theorem 1.2.1.
$m=1$
, this is Theorem 1.2.1.
Let
![]() $x\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}\cap W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{r}$
, then we have
$x\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}\cap W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{r}$
, then we have
By induction hypothesis, we have
On the other hand, there is a commutative diagram

where the lower sequence is exact by [Reference Colliot-Thélène, Sansuc and SouléCSS83, Lemma 3]. Hence there exist
![]() $y\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}},\log }^{r}$
and
$y\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}},\log }^{r}$
and
![]() $z\in \unicode[STIX]{x1D6FA}_{X,\log }^{r}$
, such that
$z\in \unicode[STIX]{x1D6FA}_{X,\log }^{r}$
, such that
![]() $x-y=\text{}\underline{p}^{m-1}\cdot z$
.
$x-y=\text{}\underline{p}^{m-1}\cdot z$
.
Since
![]() $\text{}\underline{p}^{m-1}\cdot z=x-y\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
, Corollary 2.2.7 implies
$\text{}\underline{p}^{m-1}\cdot z=x-y\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
, Corollary 2.2.7 implies
![]() $z\in \unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
. By Theorem 1.2.1, this implies
$z\in \unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}}}^{r}$
. By Theorem 1.2.1, this implies
![]() $z\in \unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}},\log }^{r}$
and hence
$z\in \unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}},\log }^{r}$
and hence
![]() $\text{}\underline{p}^{m-1}\cdot z\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}},\log }^{r}$
(cf. Theorem 1.1.6). This proves
$\text{}\underline{p}^{m-1}\cdot z\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}},\log }^{r}$
(cf. Theorem 1.1.6). This proves
![]() $x=y+\text{}\underline{p}^{m-1}\cdot z\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}},\log }^{r}$
as desired.◻
$x=y+\text{}\underline{p}^{m-1}\cdot z\in W_{m}\unicode[STIX]{x1D6FA}_{X|D_{p^{m-1}\text{}\underline{n}},\log }^{r}$
as desired.◻
3 The pairing on the relative logarithmic de Rham–Witt sheaves
Let
![]() $X,D,j:U{\hookrightarrow}X$
be as in § 2. In the following we want to define a pairing between cohomology group of
$X,D,j:U{\hookrightarrow}X$
be as in § 2. In the following we want to define a pairing between cohomology group of
![]() $W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
and cohomology group of “
$W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
and cohomology group of “
![]() $\mathop{\varprojlim }\nolimits_{D}$
”
$\mathop{\varprojlim }\nolimits_{D}$
”
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r}$
. In order to define a pairing on the sheaves level, we have to write
$W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r}$
. In order to define a pairing on the sheaves level, we have to write
![]() $W_{n}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
as ind-system of sheaves on
$W_{n}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
as ind-system of sheaves on
![]() $X$
.
$X$
.
3.1 The pairing
To define our desired pairing, we will use the notation of two-term complexes. We briefly recall the definition. In [Reference MilneMil86], Milne defined a pairing of two-term complexes as follows.
Let
and
be two-term complexes. A pairing of two-term complexes
is a system of pairings
 $$\begin{eqnarray}\displaystyle \langle \,,\rangle _{0,0}^{0}:\mathscr{F}^{0}\times \mathscr{G}^{0}\rightarrow \mathscr{H}^{0}; & & \displaystyle \nonumber\\ \displaystyle \langle \,,\rangle _{0,1}^{1}:\mathscr{F}^{0}\times \mathscr{G}^{1}\rightarrow \mathscr{H}^{1}; & & \displaystyle \nonumber\\ \displaystyle \langle \,,\rangle _{1,0}^{1}:\mathscr{F}^{1}\times \mathscr{G}^{0}\rightarrow \mathscr{H}^{1}, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \langle \,,\rangle _{0,0}^{0}:\mathscr{F}^{0}\times \mathscr{G}^{0}\rightarrow \mathscr{H}^{0}; & & \displaystyle \nonumber\\ \displaystyle \langle \,,\rangle _{0,1}^{1}:\mathscr{F}^{0}\times \mathscr{G}^{1}\rightarrow \mathscr{H}^{1}; & & \displaystyle \nonumber\\ \displaystyle \langle \,,\rangle _{1,0}^{1}:\mathscr{F}^{1}\times \mathscr{G}^{0}\rightarrow \mathscr{H}^{1}, & & \displaystyle \nonumber\end{eqnarray}$$
such that
for all
![]() $x\in \mathscr{F}^{0}$
,
$x\in \mathscr{F}^{0}$
,
![]() $y\in \mathscr{G}^{0}$
. Such a pairing is the same as a mapping
$y\in \mathscr{G}^{0}$
. Such a pairing is the same as a mapping
In our situation, for any tuple of integers
![]() $\text{}\underline{n}\geqslant \text{}\underline{1}$
we set
$\text{}\underline{n}\geqslant \text{}\underline{1}$
we set
where
![]() $Z_{1}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r}:=j_{\ast }Z_{1}W_{m}\unicode[STIX]{x1D6FA}_{U}^{r}\cap W_{m}\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r}$
with
$Z_{1}W_{m}\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r}:=j_{\ast }Z_{1}W_{m}\unicode[STIX]{x1D6FA}_{U}^{r}\cap W_{m}\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r}$
with
![]() $j:U\rightarrow X$
the canonical map and
$j:U\rightarrow X$
the canonical map and
and
![]() $C$
is the higher Cartier map [Reference KatoKat85, § 4]:
$C$
is the higher Cartier map [Reference KatoKat85, § 4]:
We also set
By [Reference MilneMil86, Lemma 1.1] we have a canonical isomorphism
Lemma 3.1.1. For any tuple of integers
![]() $\text{}\underline{n}\geqslant \text{}\underline{1}$
we have a natural pairing of two-term complexes
$\text{}\underline{n}\geqslant \text{}\underline{1}$
we have a natural pairing of two-term complexes
Proof. By the definition of filtered de Rham–Witt complexes, the cup product induces pairings
and
By composing with the higher Cartier operators, we have the following pairing:
It is easy to see that these pairings are compatible. ◻
If we now let
![]() $\text{}\underline{n}$
run over
$\text{}\underline{n}$
run over
![]() $\mathbb{N}^{\unicode[STIX]{x1D6EC}}$
, we get a pairing between an ind-object and a pro-object
$\mathbb{N}^{\unicode[STIX]{x1D6EC}}$
, we get a pairing between an ind-object and a pro-object
or equivalently, a morphism in the category of pro-objects of complexes of abelian sheaves
where
![]() $W_{m}\mathscr{H}^{\bullet }$
is viewed as a constant pro-object.
$W_{m}\mathscr{H}^{\bullet }$
is viewed as a constant pro-object.
Remark 3.1.2. To construct the pairing (3.1.7) in a more natural way, we could use a full subcategory of the ind-category of pro-objects of coherent complexes (cf. [Reference KatoKat00, § 2.1]).
4 Duality over finite fields
In this section we assume that the base field
![]() $k$
is finite. By taking hypercohomology groups of the pairing (3.1.7) using (3.1.5), we get a pairing
$k$
is finite. By taking hypercohomology groups of the pairing (3.1.7) using (3.1.5), we get a pairing
Note that there is an isomorphism in the bounded derived category
![]() $D^{b}(X,\mathbb{Z}/p^{m}\mathbb{Z})$
of étale
$D^{b}(X,\mathbb{Z}/p^{m}\mathbb{Z})$
of étale
![]() $\mathbb{Z}/p^{m}\mathbb{Z}$
-modules:
$\mathbb{Z}/p^{m}\mathbb{Z}$
-modules:
where the second isomorphism comes from the fact that
![]() $j$
is affine. Hence we get
$j$
is affine. Hence we get
Theorem 2.3.1 implies that
Combining these facts, we obtain the following corollary.
Corollary 4.1.1. We have a natural pairing of abelian groups
where the trace map is the canonical trace map of logarithmic de Rham–Witt sheaves (cf. [Reference MilneMil86, Corollary 1.12]).
Noting that
![]() $H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
are finite, we can endow
$H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
are finite, we can endow
![]() $\mathop{\varprojlim }\nolimits_{D}\,H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
with the inverse limit topology, i.e, the profinite topology.
$\mathop{\varprojlim }\nolimits_{D}\,H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
with the inverse limit topology, i.e, the profinite topology.
Proposition 4.1.2. The pairing is continuous if we endow
![]() $H^{i}(U,W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r})$
with the discrete topology and
$H^{i}(U,W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r})$
with the discrete topology and
![]() $\mathop{\varprojlim }\nolimits_{D}\,H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
with the profinite topology.
$\mathop{\varprojlim }\nolimits_{D}\,H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
with the profinite topology.
Proof. It suffices to show that the annihilator of each
![]() $\unicode[STIX]{x1D6FC}\in H^{i}(U,W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r})$
is open in the projective limit
$\unicode[STIX]{x1D6FC}\in H^{i}(U,W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r})$
is open in the projective limit
![]() $\mathop{\varprojlim }\nolimits_{D}\,H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
. This follows directly from the lemma below.◻
$\mathop{\varprojlim }\nolimits_{D}\,H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
. This follows directly from the lemma below.◻
Lemma 4.1.3. For any
![]() $\unicode[STIX]{x1D6FC}\in H^{i}(U,W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r})$
, the morphism induced by (4.1.2)
$\unicode[STIX]{x1D6FC}\in H^{i}(U,W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r})$
, the morphism induced by (4.1.2)
factors through
![]() $H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
for some
$H^{d+1-i}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r})$
for some
![]() $D\in \unicode[STIX]{x1D6F4}$
.
$D\in \unicode[STIX]{x1D6F4}$
.
Proof. This follows directly by the construction of the pairing. ◻
Our main result in this section is the following duality theorem.
Theorem 4.1.4. The pairing (4.1.2) is a perfect pairing of topological
![]() $\mathbb{Z}/p^{m}\mathbb{Z}$
-modules, i.e, it induces an isomorphism of profinite groups
$\mathbb{Z}/p^{m}\mathbb{Z}$
-modules, i.e, it induces an isomorphism of profinite groups
where
![]() $A^{\vee }$
is the Pontryagin dual of a discrete group
$A^{\vee }$
is the Pontryagin dual of a discrete group
![]() $A$
.
$A$
.
The proof is divided into two steps, the first step is to reduce the theorem to the case where
![]() $m=1$
; then we prove this special case in the second step.
$m=1$
; then we prove this special case in the second step.
Proof. Step 1. We have the following commutative diagram with exact rows

where the first row is induced by Theorem 1.1.6, and it is exact since the inverse limit is exact for projective system of finite groups. The exactness of the second row is clear. Using this commutative diagram and induction on
![]() $m$
, we reduce our question to the case
$m$
, we reduce our question to the case
![]() $m=1$
.
$m=1$
.
Step 2. For
![]() $m=1$
the pairing (3.1.6) is identified with
$m=1$
the pairing (3.1.6) is identified with
where for any
![]() $\text{}\underline{n}=(n_{\unicode[STIX]{x1D706}})_{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
(cf. the notation in (3.1.2)),
$\text{}\underline{n}=(n_{\unicode[STIX]{x1D706}})_{\unicode[STIX]{x1D706}\in \unicode[STIX]{x1D6EC}}\in \mathbb{N}^{\unicode[STIX]{x1D6EC}}$
(cf. the notation in (3.1.2)),
The perfectness of the pairings
implies that the following pairings
are perfect. In fact the perfectness of the pairing (4.1.5) follows from [Reference MilneMil76, Lemma 1.7].
By Grothendieck–Serre duality, we obtain the following isomorphisms as
![]() $k$
-vector spaces:
$k$
-vector spaces:
and
Note that, for any two
![]() $k$
-vector spaces
$k$
-vector spaces
![]() $V$
and
$V$
and
![]() $W$
, an isomorphism of
$W$
, an isomorphism of
![]() $k$
-vector spaces
$k$
-vector spaces
uniquely corresponds to an isomorphism of
![]() $\mathbb{F}_{p}$
-vector spaces
$\mathbb{F}_{p}$
-vector spaces
The above two isomorphisms give the isomorphism
![]() $(1)$
in the following commutative diagram
$(1)$
in the following commutative diagram

where the isomorphism (2) is induced by Theorem 1.2.1, (3) follows from the observation that
![]() $j_{\ast }\unicode[STIX]{x1D6FA}_{U}^{r}=\mathop{\varinjlim }\nolimits_{\text{}\underline{n}}\,\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r}$
, and the isomorphism (4) is due to the fact that
$j_{\ast }\unicode[STIX]{x1D6FA}_{U}^{r}=\mathop{\varinjlim }\nolimits_{\text{}\underline{n}}\,\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r}$
, and the isomorphism (4) is due to the fact that
![]() $Rj_{\ast }\unicode[STIX]{x1D6FA}_{U,\log }^{r}\cong [j_{\ast }Z\unicode[STIX]{x1D6FA}_{U}^{r}\xrightarrow[{}]{1-C}j_{\ast }\unicode[STIX]{x1D6FA}_{U}^{r}]$
. Therefore the last horizontal map is an isomorphism.◻
$Rj_{\ast }\unicode[STIX]{x1D6FA}_{U,\log }^{r}\cong [j_{\ast }Z\unicode[STIX]{x1D6FA}_{U}^{r}\xrightarrow[{}]{1-C}j_{\ast }\unicode[STIX]{x1D6FA}_{U}^{r}]$
. Therefore the last horizontal map is an isomorphism.◻
In particular, for
![]() $i=1$
and
$i=1$
and
![]() $r=0$
we get isomorphisms
$r=0$
we get isomorphisms
and
These isomorphisms can be used to define a measure of ramification for étale abelian covers of
![]() $U$
whose degree divides
$U$
whose degree divides
![]() $p^{m}$
.
$p^{m}$
.
Definition 4.1.5. For any
![]() $D\in \unicode[STIX]{x1D6F4}$
, we define
$D\in \unicode[STIX]{x1D6F4}$
, we define
 $$\begin{eqnarray}\displaystyle & \displaystyle \text{Fil}_{D}H^{1}(U,\mathbb{Z}/p^{m}\mathbb{Z}):=H^{d}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d})^{\vee }, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \text{Fil}_{D}H^{1}(U,\mathbb{Q}/\mathbb{Z}):=H^{1}(U,\mathbb{Q}/\mathbb{Z})\{p^{\prime }\}\bigoplus \mathop{\bigcup }_{m\geqslant 1}\text{Fil}_{D}H^{1}(U,\mathbb{Z}/p^{m}\mathbb{Z}), & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \text{Fil}_{D}H^{1}(U,\mathbb{Z}/p^{m}\mathbb{Z}):=H^{d}(X,W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d})^{\vee }, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \text{Fil}_{D}H^{1}(U,\mathbb{Q}/\mathbb{Z}):=H^{1}(U,\mathbb{Q}/\mathbb{Z})\{p^{\prime }\}\bigoplus \mathop{\bigcup }_{m\geqslant 1}\text{Fil}_{D}H^{1}(U,\mathbb{Z}/p^{m}\mathbb{Z}), & \displaystyle \nonumber\end{eqnarray}$$
where
![]() $H^{1}(U,\mathbb{Q}/\mathbb{Z})\{p^{\prime }\}$
is the prime-to-
$H^{1}(U,\mathbb{Q}/\mathbb{Z})\{p^{\prime }\}$
is the prime-to-
![]() $p$
part of
$p$
part of
![]() $H^{1}(U,\mathbb{Q}/\mathbb{Z})$
. Dually we define
$H^{1}(U,\mathbb{Q}/\mathbb{Z})$
. Dually we define
The group
![]() $\unicode[STIX]{x1D70B}_{1}^{\text{ab}}(X,D)/p^{m}$
is a quotient of
$\unicode[STIX]{x1D70B}_{1}^{\text{ab}}(X,D)/p^{m}$
is a quotient of
![]() $\unicode[STIX]{x1D70B}_{1}^{\text{ab}}(U)/p^{m}$
, which can be thought of as classifying abelian étale coverings of
$\unicode[STIX]{x1D70B}_{1}^{\text{ab}}(U)/p^{m}$
, which can be thought of as classifying abelian étale coverings of
![]() $U$
whose degree divides
$U$
whose degree divides
![]() $p^{m}$
with ramification bounded by
$p^{m}$
with ramification bounded by
![]() $D$
. These groups are important objects in higher-dimensional class field theory.
$D$
. These groups are important objects in higher-dimensional class field theory.
5 Duality over perfect fields
When the base field
![]() $k$
is finite, our duality theory is formulated by endowing the cohomology groups with the structure of topological groups. When the base field
$k$
is finite, our duality theory is formulated by endowing the cohomology groups with the structure of topological groups. When the base field
![]() $k$
is not finite, it is necessary to endow the cohomology groups with stronger structures, namely the structures of proalgebraic and indalgebraic groups, and use Breen–Serre duality instead of Pontryagin duality. In this section,
$k$
is not finite, it is necessary to endow the cohomology groups with stronger structures, namely the structures of proalgebraic and indalgebraic groups, and use Breen–Serre duality instead of Pontryagin duality. In this section,
![]() $k$
denotes a perfect field of characteristic
$k$
denotes a perfect field of characteristic
![]() $p>0$
, not necessarily finite, and we put
$p>0$
, not necessarily finite, and we put
![]() $S=\text{Spec}(k)$
.
$S=\text{Spec}(k)$
.
5.1 The relative perfect étale site
Recall a scheme
![]() $T$
is said to be perfect if the absolute Frobenius morphism
$T$
is said to be perfect if the absolute Frobenius morphism
![]() $F:T\rightarrow T$
is an isomorphism. For any
$F:T\rightarrow T$
is an isomorphism. For any
![]() $S$
-scheme
$S$
-scheme
![]() $X$
, the perfection
$X$
, the perfection
![]() $X^{\text{pf}}$
of
$X^{\text{pf}}$
of
![]() $X$
is the projective limit of the system
$X$
is the projective limit of the system
where
![]() $X_{\text{red}}^{(p^{-n})}$
is the scheme
$X_{\text{red}}^{(p^{-n})}$
is the scheme
![]() $X_{\text{red}}$
with the structure map
$X_{\text{red}}$
with the structure map
![]() $F^{n}\circ \unicode[STIX]{x1D70B}:X\rightarrow S$
. It is a perfect scheme, and has the universal property that
$F^{n}\circ \unicode[STIX]{x1D70B}:X\rightarrow S$
. It is a perfect scheme, and has the universal property that
for any perfect
![]() $S$
-scheme
$S$
-scheme
![]() $Y$
. A perfect
$Y$
. A perfect
![]() $S$
-scheme
$S$
-scheme
![]() $X$
is said to be algebraic if it is the perfection of a scheme of finite type over
$X$
is said to be algebraic if it is the perfection of a scheme of finite type over
![]() $S$
. One sees easily that the perfect algebraic group schemes over
$S$
. One sees easily that the perfect algebraic group schemes over
![]() $S$
form an abelian category. Let
$S$
form an abelian category. Let
![]() $(\mathit{Pf}/S)_{\acute{\text{e}}\text{t}}$
be the perfect étale site over
$(\mathit{Pf}/S)_{\acute{\text{e}}\text{t}}$
be the perfect étale site over
![]() $S$
, i.e., the category of perfect schemes over
$S$
, i.e., the category of perfect schemes over
![]() $S$
with étale topology.
$S$
with étale topology.
In what follows we fix a smooth proper morphism
![]() $\unicode[STIX]{x1D70B}:X\rightarrow S$
and an effective divisor
$\unicode[STIX]{x1D70B}:X\rightarrow S$
and an effective divisor
![]() $D$
such that Supp(
$D$
such that Supp(
![]() $D$
) is a simple normal crossing divisor on
$D$
) is a simple normal crossing divisor on
![]() $X$
. Let
$X$
. Let
![]() $j:U:=X-D{\hookrightarrow}X$
be the complement of
$j:U:=X-D{\hookrightarrow}X$
be the complement of
![]() $D$
. Let
$D$
. Let
![]() $(\mathit{Pf}X/S)_{\acute{\text{e}}\text{t}}$
be the relative perfect étale site over
$(\mathit{Pf}X/S)_{\acute{\text{e}}\text{t}}$
be the relative perfect étale site over
![]() $X/S$
, i.e., the category of pairs
$X/S$
, i.e., the category of pairs
![]() $(T,Y)$
, where
$(T,Y)$
, where
![]() $T$
is a perfect scheme over
$T$
is a perfect scheme over
![]() $S$
and
$S$
and
![]() $Y$
is étale over
$Y$
is étale over
![]() $X\times _{S}T$
equipped with étale topology. We define
$X\times _{S}T$
equipped with étale topology. We define
![]() $\mathscr{X}$
and
$\mathscr{X}$
and
![]() $\mathscr{S}$
to be the category of abelian sheaves on
$\mathscr{S}$
to be the category of abelian sheaves on
![]() $(\mathit{Pf}X/S)_{\acute{\text{e}}\text{t}}$
and on
$(\mathit{Pf}X/S)_{\acute{\text{e}}\text{t}}$
and on
![]() $(\mathit{Pf}/S)_{\acute{\text{e}}\text{t}}$
, respectively. For any integer
$(\mathit{Pf}/S)_{\acute{\text{e}}\text{t}}$
, respectively. For any integer
![]() $m\geqslant 1$
, we denote
$m\geqslant 1$
, we denote
![]() $\mathscr{X}(p^{m})$
(respectively
$\mathscr{X}(p^{m})$
(respectively
![]() $\mathscr{S}(p^{m})$
) to be the category of sheaves of
$\mathscr{S}(p^{m})$
) to be the category of sheaves of
![]() $\mathbb{Z}/p^{m}\mathbb{Z}$
-modules on
$\mathbb{Z}/p^{m}\mathbb{Z}$
-modules on
![]() $(\mathit{Pf}X/S)_{\acute{\text{e}}\text{t}}$
(respectively
$(\mathit{Pf}X/S)_{\acute{\text{e}}\text{t}}$
(respectively
![]() $(\mathit{Pf}/S)_{\acute{\text{e}}\text{t}}$
). The structure morphism
$(\mathit{Pf}/S)_{\acute{\text{e}}\text{t}}$
). The structure morphism
![]() $\unicode[STIX]{x1D70B}:X\rightarrow S$
induces a morphism of sites
$\unicode[STIX]{x1D70B}:X\rightarrow S$
induces a morphism of sites
which gives rise to adjoint functors
Definition 1.1.1 gives an object
![]() $W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
of
$W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
of
![]() $\mathscr{X}(p^{m})$
such that
$\mathscr{X}(p^{m})$
such that
![]() $R^{i}\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
is the sheaf on
$R^{i}\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{r}$
is the sheaf on
![]() $(\mathit{Pf}/S)_{\acute{\text{e}}\text{t}}$
associated to the presheaf
$(\mathit{Pf}/S)_{\acute{\text{e}}\text{t}}$
associated to the presheaf
5.2 Duality theorem
By (3.1.2) we have an isomorphism
where the second isomorphism follows from the fact that
![]() $j$
is affine. Therefore
$j$
is affine. Therefore
since
![]() $R\,\mathop{\varinjlim }\nolimits_{\text{}\underline{n}}$
commutes with
$R\,\mathop{\varinjlim }\nolimits_{\text{}\underline{n}}$
commutes with
![]() $R\unicode[STIX]{x1D70B}_{\ast }$
. By (3.1.6) and (3.1.5), we have a map
$R\unicode[STIX]{x1D70B}_{\ast }$
. By (3.1.6) and (3.1.5), we have a map
By taking the homotopy limit
![]() $R\,\mathop{\varprojlim }\nolimits_{\text{}\underline{n}}$
on both sides, we obtain a map
$R\,\mathop{\varprojlim }\nolimits_{\text{}\underline{n}}$
on both sides, we obtain a map
 $$\begin{eqnarray}\displaystyle R\,\underset{\text{}\underline{n}}{\varprojlim }\,R\unicode[STIX]{x1D70B}_{\ast }W_{m}\mathscr{G}_{\text{}\underline{n}+\text{}\underline{1}}^{d-r,\bullet } & \rightarrow & \displaystyle R\,\underset{\text{}\underline{n}}{\varprojlim }\,R{\mathcal{H}}om_{\mathscr{S}}(R\unicode[STIX]{x1D70B}_{\ast }W_{m}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet },R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{d})\nonumber\\ \displaystyle & \simeq & \displaystyle R{\mathcal{H}}om_{\mathscr{S}}\biggl(R\,\underset{\text{}\underline{n}}{\varinjlim }\,R\unicode[STIX]{x1D70B}_{\ast }W_{m}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet },R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{d}\biggr)\nonumber\\ \displaystyle & \simeq & \displaystyle R{\mathcal{H}}om_{\mathscr{S}}(R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r},R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{d})\nonumber\\ \displaystyle & \rightarrow & \displaystyle R{\mathcal{H}}om_{\mathscr{S}}(R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r},\mathbb{Z}/p^{m}\mathbb{Z})[-d]\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle R\,\underset{\text{}\underline{n}}{\varprojlim }\,R\unicode[STIX]{x1D70B}_{\ast }W_{m}\mathscr{G}_{\text{}\underline{n}+\text{}\underline{1}}^{d-r,\bullet } & \rightarrow & \displaystyle R\,\underset{\text{}\underline{n}}{\varprojlim }\,R{\mathcal{H}}om_{\mathscr{S}}(R\unicode[STIX]{x1D70B}_{\ast }W_{m}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet },R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{d})\nonumber\\ \displaystyle & \simeq & \displaystyle R{\mathcal{H}}om_{\mathscr{S}}\biggl(R\,\underset{\text{}\underline{n}}{\varinjlim }\,R\unicode[STIX]{x1D70B}_{\ast }W_{m}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet },R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{d}\biggr)\nonumber\\ \displaystyle & \simeq & \displaystyle R{\mathcal{H}}om_{\mathscr{S}}(R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r},R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{d})\nonumber\\ \displaystyle & \rightarrow & \displaystyle R{\mathcal{H}}om_{\mathscr{S}}(R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r},\mathbb{Z}/p^{m}\mathbb{Z})[-d]\nonumber\end{eqnarray}$$
where the second isomorphism follows from (5.2.1) and the last map is induced by the trace map
![]() $\text{Tr}:R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{d}\rightarrow \mathbb{Z}/p^{m}\mathbb{Z}[-d]$
. Thus Theorem 2.3.1 gives rise to a map
$\text{Tr}:R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X,\log }^{d}\rightarrow \mathbb{Z}/p^{m}\mathbb{Z}[-d]$
. Thus Theorem 2.3.1 gives rise to a map
Theorem 5.2.1. The map (5.2.2) is an isomorphism in
![]() $D^{b}(\mathscr{S}(p^{m}))$
.
$D^{b}(\mathscr{S}(p^{m}))$
.
Proof. By the same method as in the proof of Theorem 4.1.4, we reduce the claim to the case
![]() $m=1$
. We then use the following result from [Reference MilneMil76, Proposition 2.1], [Reference BerthelotBer81, Lemma 3.6].
$m=1$
. We then use the following result from [Reference MilneMil76, Proposition 2.1], [Reference BerthelotBer81, Lemma 3.6].
Proposition 5.2.2. Let
![]() $\mathscr{L}$
be a locally free
$\mathscr{L}$
be a locally free
![]() ${\mathcal{O}}_{X}$
-module of finite rank and put
${\mathcal{O}}_{X}$
-module of finite rank and put
![]() $\mathscr{L}^{\vee }={\mathcal{H}}om_{{\mathcal{O}}_{X}}(\mathscr{L},{\mathcal{O}}_{X})$
. Then the natural pairing
$\mathscr{L}^{\vee }={\mathcal{H}}om_{{\mathcal{O}}_{X}}(\mathscr{L},{\mathcal{O}}_{X})$
. Then the natural pairing
and the trace map
![]() $R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X,\log }^{d}\rightarrow \mathbb{Z}/p\mathbb{Z}[-d]$
induces an isomorphism
$R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X,\log }^{d}\rightarrow \mathbb{Z}/p\mathbb{Z}[-d]$
induces an isomorphism
Corollary 5.2.3. The perfect pairings (4.1.4) and (4.1.5) induce isomorphisms
 $$\begin{eqnarray}\displaystyle & R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}+\text{}\underline{1}}}^{d-r}\xrightarrow[{}]{\cong }R{\mathcal{H}}om_{\mathscr{S}(p)}(R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r},\mathbb{Z}/p\mathbb{Z})[-d+1]; & \displaystyle \nonumber\\ \displaystyle & R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}+\text{}\underline{1}}}^{d-r}/d\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}+\text{}\underline{1}}}^{d-r-1}\xrightarrow[{}]{\cong }R{\mathcal{H}}om_{\mathscr{S}(p)}(R\unicode[STIX]{x1D70B}_{\ast }Z\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r},\mathbb{Z}/p\mathbb{Z})[-d+1]. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}+\text{}\underline{1}}}^{d-r}\xrightarrow[{}]{\cong }R{\mathcal{H}}om_{\mathscr{S}(p)}(R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r},\mathbb{Z}/p\mathbb{Z})[-d+1]; & \displaystyle \nonumber\\ \displaystyle & R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}+\text{}\underline{1}}}^{d-r}/d\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}+\text{}\underline{1}}}^{d-r-1}\xrightarrow[{}]{\cong }R{\mathcal{H}}om_{\mathscr{S}(p)}(R\unicode[STIX]{x1D70B}_{\ast }Z\unicode[STIX]{x1D6FA}_{X|D_{-\text{}\underline{n}}}^{r},\mathbb{Z}/p\mathbb{Z})[-d+1]. & \displaystyle \nonumber\end{eqnarray}$$
Therefore, we have an isomorphism
where
![]() $W_{1}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet }$
and
$W_{1}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet }$
and
![]() $W_{1}\mathscr{G}_{\text{}\underline{n}+\text{}\underline{1}}^{d-r,\bullet }$
were defined in (3.1.2) and (3.1.3).
$W_{1}\mathscr{G}_{\text{}\underline{n}+\text{}\underline{1}}^{d-r,\bullet }$
were defined in (3.1.2) and (3.1.3).
Proof of Theorem 5.2.1(continued).
By taking the limit, we obtain
 $$\begin{eqnarray}\displaystyle R\,\underset{D}{\varprojlim }\,R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r} & \xrightarrow[{}]{\cong } & \displaystyle R\,\underset{\text{}\underline{n}}{\varprojlim }\,R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}+\text{}\underline{1}},\log }^{d-r}\xrightarrow[{}]{\cong }R\,\underset{\text{}\underline{n}}{\varprojlim }\,R\unicode[STIX]{x1D70B}_{\ast }W_{1}\mathscr{G}_{\text{}\underline{n}+\text{}\underline{1}}^{d-r,\bullet }\nonumber\\ \displaystyle & \xrightarrow[{}]{\cong } & \displaystyle R\,\underset{\text{}\underline{n}}{\varprojlim }\,R{\mathcal{H}}om_{\mathscr{S}(p)}(R\unicode[STIX]{x1D70B}_{\ast }W_{1}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet },\mathbb{Z}/p\mathbb{Z})[-d]\nonumber\\ \displaystyle & \xrightarrow[{}]{\cong } & \displaystyle R{\mathcal{H}}om_{\mathscr{S}(p)}(R\,\underset{\text{}\underline{n}}{\varinjlim }\,R\unicode[STIX]{x1D70B}_{\ast }W_{1}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet },\mathbb{Z}/p\mathbb{Z})[-d]\nonumber\\ \displaystyle & \xrightarrow[{}]{\cong } & \displaystyle R{\mathcal{H}}om_{\mathscr{S}(p)}(R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }\unicode[STIX]{x1D6FA}_{U,\log }^{r},\mathbb{Z}/p\mathbb{Z})[-d].\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle R\,\underset{D}{\varprojlim }\,R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r} & \xrightarrow[{}]{\cong } & \displaystyle R\,\underset{\text{}\underline{n}}{\varprojlim }\,R\unicode[STIX]{x1D70B}_{\ast }\unicode[STIX]{x1D6FA}_{X|D_{\text{}\underline{n}+\text{}\underline{1}},\log }^{d-r}\xrightarrow[{}]{\cong }R\,\underset{\text{}\underline{n}}{\varprojlim }\,R\unicode[STIX]{x1D70B}_{\ast }W_{1}\mathscr{G}_{\text{}\underline{n}+\text{}\underline{1}}^{d-r,\bullet }\nonumber\\ \displaystyle & \xrightarrow[{}]{\cong } & \displaystyle R\,\underset{\text{}\underline{n}}{\varprojlim }\,R{\mathcal{H}}om_{\mathscr{S}(p)}(R\unicode[STIX]{x1D70B}_{\ast }W_{1}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet },\mathbb{Z}/p\mathbb{Z})[-d]\nonumber\\ \displaystyle & \xrightarrow[{}]{\cong } & \displaystyle R{\mathcal{H}}om_{\mathscr{S}(p)}(R\,\underset{\text{}\underline{n}}{\varinjlim }\,R\unicode[STIX]{x1D70B}_{\ast }W_{1}\mathscr{F}_{-\text{}\underline{n}}^{r,\bullet },\mathbb{Z}/p\mathbb{Z})[-d]\nonumber\\ \displaystyle & \xrightarrow[{}]{\cong } & \displaystyle R{\mathcal{H}}om_{\mathscr{S}(p)}(R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }\unicode[STIX]{x1D6FA}_{U,\log }^{r},\mathbb{Z}/p\mathbb{Z})[-d].\nonumber\end{eqnarray}$$
This is our theorem in the case that
![]() $m=1$
.◻
$m=1$
.◻
Remark 5.2.4. In fact we can endow
![]() $R\,\mathop{\varprojlim }\nolimits_{D}\,R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r}$
with a structure of a complex of proalgebraic groups, i.e., as an object in the bounded derived category of quasi-unipotent proalgebraic groups, and similarly view
$R\,\mathop{\varprojlim }\nolimits_{D}\,R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r}$
with a structure of a complex of proalgebraic groups, i.e., as an object in the bounded derived category of quasi-unipotent proalgebraic groups, and similarly view
![]() $R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
as an object in the bounded derived category of quasi-unipotent indalgebraic groups. Then Theorem 5.2.2 identifies
$R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
as an object in the bounded derived category of quasi-unipotent indalgebraic groups. Then Theorem 5.2.2 identifies
![]() $R\,\mathop{\varprojlim }\nolimits_{D}\,R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r}$
with the Breen–Serre dual of
$R\,\mathop{\varprojlim }\nolimits_{D}\,R\unicode[STIX]{x1D70B}_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{X|D,\log }^{d-r}$
with the Breen–Serre dual of
![]() $R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
(cf. [Reference PépinPép14, § 2.5]).
$R\unicode[STIX]{x1D70B}_{\ast }Rj_{\ast }W_{m}\unicode[STIX]{x1D6FA}_{U,\log }^{r}$
(cf. [Reference PépinPép14, § 2.5]).
Acknowledgements
The authors thank Moritz Kerz for his advice, especially on the construction of a pairing. The authors would like to thank the anonymous referee for his/her numerous valuable comments and suggestions to improve the quality of this paper. In particular, his/her suggestion on a simplification of the construction of the filtered de Rham–Witt complexes in § 2 is invaluable.