Published online by Cambridge University Press: 04 October 2023
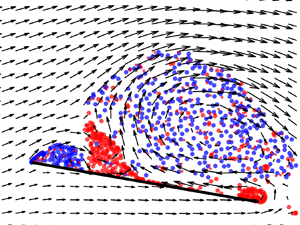
The lift generation mechanism of leading-edge vortex (LEV) in the case of a pitching and plunging plate is studied using an experimental approach and the improved discrete vortex method in this research. A formation condition of the secondary structure is introduced into the traditional discrete vortex method to compensate for the shortcomings in the simulation of the viscous effect between LEV and plate. The simulation of the secondary structure helps the improved method perform better in flow-field reconstruction and lift prediction. Accordingly, the lift generation mechanism of the LEV and influence of the secondary structure are studied. The lift contribution of the vortex structure is isolated and linearly decomposed into two parts according to sources of flow field: the quasi-potential flow part and the vortex-induced flow part. The vortex lift is defined as the lift contribution of the vortex structure in vortex-induced flow, which gives a new insight into the production of lift of the LEV. The lift generation mechanism through the discrete vortex method is verified and extended in viscous flow through experimental measurement. In addition, a vortex lift indicator based on the reverse flow of the LEV is proposed to examine the change of vortex lift in experimental measurement. The flow mechanism for the decline of vortex lift for different maximum effective angles of attack is revealed based on the vortex lift indicator. Furthermore, for the LEV-dominating flow, the indicator can also be applied in estimating the maximum value and corresponding critical time of overall lift in experiments.