Human capital and pensions with endogenous fertility and retirement

Abstract

We study an OLG model with child policies and a PAYG pension with endogenous retirement and fertility. The result of the planned economy is compared to the decentralized competitive equilibrium deriving optimal policies. We show that in the presence of a PAYG pension system, the optimal policy mix includes an education subsidy and a subsidy for the supply of labor in old age. Fertility should be taxed or incentivized depending on whether there is full or partial retirement, and on the parameters. We focus on the parameter reflecting the deterioration of human capital and show that a child tax may be required.

1. Introduction

This paper develops an overlapping generations model for a small open economy, where fertility, human capital and old agent’s labor supply are endogenously determined. Reflecting the recent trends of increase in the retirement age and labor force participation along with educational attainment (Grigoli et al. (2018) and Geppert et al. (2019)), we extend the model of van Groezen et al. (2003) by adding human capital and endogenous retirement choice. This enables us to study the impact of various government policy combinations in order to make market and socially optimum allocations coincide. In particular, we focus on the role of child benefits, education subsidization, and subsidies to the elderly labor supply.

The motivation behind our analysis is that, although in the past decade pension reforms have been widespread in all OECD countries, pressures nevertheless persist to ensure the maintenance of sufficient and financially sustainable levels of pensions in most developed economies (OECD (2019a)) and, according to Geppert et al. (2019) educational attainment is forecast to make the greatest contribution to pension schemes, a fact that should more than compensate for the negative impact of population aging.

Thus, the contribution of this paper to the existing literature is the focus not only on policies to increase fertility but also on their combination with policies to incentivize human capital accumulation in a model with social security and endogenous retirement. In particular, in a PAYG social security system, the market solution is not necessarily socially optimal because agents do not have the right incentive to choose the optimal fertility rate, education, and retirement age, given that they do not take into account the social externalities associated with their choices. As far as fertility choices are concerned, the literature emphasizes three main external effects associated with fertility choice: the intergenerational transfer effect, the child quality effect, and the capital dilution effect (see e.g., Cremer et al. (2011) and Cipriani (2014)). The first effect arises from the fact that each person’s fertility decisions affect the size of the future labor force and therefore pension benefits. However, agents do not take into consideration this positive effect on pensions, leading to a market equilibrium with a low fertility rate (see among others Cigno and Rosati (1996), Cigno (2006, 2009), Ehrlich and Lui (1998), Nishimura and Zhang (1992), and Cremer et al. (2006)). The child quality effect is linked to the externality from the investment in children’s education. Higher investments in children’s human capital, in fact, raise the return of a PAYG pension system because higher future wages increase tax revenues which can be allocated to the pension system (see Meier and Wrede (2010), Cremer et al. (2011)). Finally, the third effect, the capital dilution externality, travels in the opposite direction: a higher fertility rate reduces the capital:labour ratio and therefore also per capita income and pension benefits (see Michel and Pestieau (1993) and Cigno (1993)). Child subsidies and fertility-related pensions are the two main policy instruments highlighted in the literature in order to internalize these fiscal externalities. Concerning investment in education, in addition to the child quality effect, in a PAYG system with elderly labor supply, underinvestment in human capital also arises because agents do not fully internalize the marginal gain from the investment in education on labor earnings in old age. Education subsidization is the principal instrument highlighted in the literature to tackle underinvestment in human capital (Zhang (2003), Yew and Zhang (2009, 2013)). In a framework characterized by a PAYG pension system and both endogenous fertility and education, it is essential to consider the relationship between pension schemes and the trade-off between the quality and quantity of children. In fact if, on the one hand, education subsidization increases human capital accumulation; on the other hand, fertility may decrease via the trade-off between the quantity and quality of children and therefore, because of these opposing forces, the rate of return of the PAYG pension system may decrease. Thus, it is crucial to determine the optimum combination of child and education subsidies in order to correct both externalities from fertility and education decisions. An early example of this is Peters (1995) who uses a general social welfare function in order to derive the optimal combination of child and educational subsidies by analyzing US and German pension systems. His main result is that while investment in education must always be subsidized, it is not necessary however to provide child benefits and in fact, in some cases may be necessary to introduce a tax on children. Cremer et al. (2011) and Stauvermann and Kumar (2018) have obtained similar results. In particular, Cremer et al. (2011) add the possibility that children’s abilities are in part stochastic, in part determined by family background and in part determined through education.

Overall, these models focus on fertility and education policies but do not consider retirement choices and therefore ignore the interaction between child policies and those devoted to increasing labor supply in old age. We add to this literature by extending the framework developed by Cipriani and Fioroni (2022) to include investment in human capital. To the best of our knowledge, no study to date has analyzed the optimal policies that aim to attain the first-best allocation and their welfare implications in a PAYG pension system with elderly labor supply and both endogenous education and fertility. This enables us to focus on the joint effects of policies devoted to internalizing the social externalities of fertility, education, and labor supply in old age.

The majority of the existing literature concentrates on the link between endogenous elderly labor supply and life expectancy without including fertility choice (see, e.g., Aísa et al. (2012), Cipriani (2014) and Nishimura et al. (2018)). The recent paper by Cipriani and Pascucci (2020) includes retirement policies in a model with endogenous fertility, but in their framework retirement is exogenous and thus they do not focus on the first-best policies. Cipriani and Fioroni (2021) develop a framework with both endogenous fertility and endogenous elderly labor supply with a view to comparing the impact of different retirement schemes, but they do not look at potential government policies in order to attain the first-best allocation. Hirazawa and Yakita (2017) develop a framework with endogenous life expectancy, retirement, fertility, and human capital accumulation but do not consider pension systems.

Michel and Pestieau (2013) and Miyazaki (2019) are among the few recent authors who have focused on policies aimed at reaching the first-best allocation in the presence of endogenous retirement, but they ignore fertility choices. Zhang and Zhang (2009) and Yew and Zhang (2013), on the other hand, concentrate on socially optimal government policies in a dynastic family model with human capital externalities and endogenous fertility, but they do not include endogenous retirement. Tamura and Cuberes (2020) use the A-efficiency criteria to study the gap between optimal fertility and investment in human capital. In particular, with respect to our approach, they suppose a sequence of myopic social planners who maximize the utility of those alive. Andersen and Bhattacharya (2017) and Bishnu et al. (2021) focus on efficient allocations in models with both human capital and pensions, but they abstract from endogenous fertility.

Finally, Chen and Miyazaki (2018) develop an overlapping generations model to examine the impact of PAYG pension systems and child allowances on fertility, the elderly labor supply, and welfare, but investment in education is not considered. The inclusion of human capital choices allows us to capture the interaction between social security on the one hand and the trade-off between the quality and quantity of children on the other hand. In particular, adding human capital to a framework where agents choose when to retire is particularly relevant because education has both a direct and an indirect effect via the choice of retirement age, on the rate of return of a PAYG system. Moreover, in accordance with Aísa et al. (2012) and Nishimura et al. (2018), we assume that human capital depreciates with age and this crucially affects retirement decisions and therefore also the optimal policy combinations. Overall, we show that agents choose a retirement period which is suboptimal and behind this result may lie three possible mechanisms. First, according to the existing literature, pension provisions reduce the marginal cost of leisure and therefore agents tend to retire early (Gruber and Wise (1998)). Second, in a PAYG pension system, elderly agents tend to choose a suboptimal labor supply because they do not consider the positive effect that their labor supply has on pension benefits. Finally, agents fail to consider that a higher labor supply, for a given aggregate saving and fertility rate, decreases the capital labor ratio. This can result in a higher than optimum retirement age. We conclude that governments can achieve the first-best allocation through a policy mix which includes education subsidization and a subsidy for elderly labor supply, whereas fertility should not necessarily always be subsidized and in fact, in some cases, a tax on children may be required in order to achieve the first-best solution.

In particular, we focus on two alternative scenarios: (i) full retirement, when agents retire at the beginning of old age; (ii) partial retirement, when agents work for part of the last period. The main result is that while in the first scenario the first-best outcome can be realized through a policy consisting of both a child benefit scheme and a subsidy for education investment, and in the second the introduction of a subsidy for elderly labor supply can remove the need to subsidize fertility if human capital decay is sufficiently low. In fact, a higher human capital productivity maintained in the second period increases fertility through a positive income effect and therefore a tax on children may be required in order to attain the first-best solution. Our focus on human capital decay is particularly important since preventing it is one of the main policy recommendations to promote the sustainability of a pension system (OECD (2019b)).

The structure of the paper is as follows: Section 2 presents the basic model; Section 3 compares the optimum allocation versus the market allocation; Section 4 provides a numerical example in order to study different policy combinations to achieve the first-best allocation and their welfare implications. The final section summarizes our results.

2. The model

Let us consider a small open economy populated by overlapping generations of people who live for three periods: childhood, adulthood, and old age. In the first period, agents are children and make no decisions; in adulthood, they work full-time, raise their offspring, and invest in the education of their children. In the third period, they face a retirement choice. When they retire, they receive a state-funded PAYG pension.

2.1 Production

Production occurs within a period according to a neoclassical, constant returns to scale technology. Assuming a Cobb–Douglas production function, output produced at time $t$ , $Y_t$ is given by:

(1) \begin{equation} Y_t=A K_t^{\psi } H_t^{1-\psi } \end{equation}

where $K_t$ and $H_t$ are physical capital and the aggregate quantity of efficiency units of labor employed in production in period $t$ , respectively, $A \gt 0$ is a technological parameter, and $\psi \in (0,1)$ .

The effective labor supply in each period $t$ is given by the number of effective labor units supplied by young workers, that is, $h_tN_t$ and the number of effective labor units from elderly workers at time $t$ , that is, $\lambda h_{t-1} l_t N_{t-1}$ where $h_t$ is the human capital level for each young worker at time $t$ , $l_t$ is the labor supply during the old age, and the parameter $\lambda \in (0,1)$ captures the fraction of youth productivity maintained in old age (see Aísa et al. (2012), Nishimura et al. (2018), and Hirazawa and Yakita (2017)). Thus, $\lambda$ close to $0$ implies a significant decline in human capital. Given $N_t=n_{t-1}N_{t-1}$ , the total amount of effective labor at time $t$ is given by:

(2) \begin{equation} H_t=N_t\!\left (h_t+\frac{\lambda h_{t-1} l_t}{n_{t-1}}\right ) \end{equation}

With perfectly mobile capital, the interest rate is equal to the world interest rate $r$ , which is assumed to be exogenous and constant over time. Thus, given a constant-returns-to-scale technology, both capital per unit of effective labor and the wage $w$ per unit of human capital are fixed and constant over time.

In accordance with existing literature, we assume that the level of human capital of children in period $t+1$ , is an increasing, strictly concave function of parental investment in education (or the share of income that parents devote to education). ¹ In particular, for the sake of simplicity, we specify the following reduced form for human capital production function (Rehme (2007)): ²

(3) \begin{equation} h_{t+1}=e_t^{\epsilon } \end{equation}

where $\epsilon \in (0,1)$ .

2.2 Households

Adults (born in period $t-1$ ) derive utility from consumption in adult age, that is, $c^y_t$ , the number of children $n_t$ , the human capital of their offspring $h_{t+1}$ , from consumption $c^o_{t+1}$ , and leisure time $(1-l_{t+1})$ after retirement. Thus, the utility function is given by:

(4) \begin{equation} U^t=\ln c_t^y+\theta \ln n_t h_{t+1}+\beta [\ln c^o_{t+1}+\delta\! \ln (1-l_{t+1})] \end{equation}

where $\beta \in (0,1)$ is the overall weight attached to utility in old age, $\delta \in (0,1)$ is the weight of retirement, and $\theta$ reflects the preferences given to children (quality as well as quantity). ³

In adulthood, agents apportion their wage between consumption $c^y_t$ , saving $s_t$ , raising their children $n_t$ , investing in their children’s education $e_t$ , and paying a tax $\tau$ in order to contribute to the PAYG pension scheme. Thus, the budget constraint in adulthood is given by:

(5) \begin{equation} c^y_t=wh_t(1-\tau -qn_t-e_tn_t)-s_t \end{equation}

where $q\gt 0$ is the cost of raising each child. In old age, agents consume their savings and if they work they pay a tax to finance the PAYG scheme, whereas they receive a pension benefit $b_{t+1}$ for the period during which they do not work. Thus, the budget constraint in old age is as follows:

(6) \begin{equation} c^o_{t+1}=Rs_t+\lambda w h_{t} l_{t+1}(1-\tau )+b_{t+1}(1-l_{t+1}) \end{equation}

where $R=1+r$ is the rate of return on savings.

The government has to observe a balanced budget and a PAYG social security scheme. Thus, the revenue from taxing both the young, that is, $\tau w h_{t+1}n_tN_t$ , and the elderly, that is, $\tau w\lambda h_t l_{t+1}N_t$ is used to finance retirement pensions. Thus, pension benefits are given by:

(7) \begin{equation} b_{t+1}=\frac{\tau w (h_{t+1} n_t+\lambda h_t l_{t+1})}{1-l_{t+1}} \end{equation}

DEFINITION 1. Given $w$ , $R$ , and an initial state $K_0, H_0, N_0$ , a competitive equilibrium in the economy with PAYG social security is a sequence of allocations $\{c^y_t, c^o_{t}, e_t, n_t, s_t, l_t, k_t\}^\infty _{t=0}$ such that (i) taking the social security tax rate as given, firms and households optimize and their choices satisfy the budget constraints (5) and (6) the technologies (1) and (2), (ii) the social security budget is balanced, and (iii) goods and factor markets clear.

Specifically, households maximization of the the utility function (4) subject to (3), (5), (6) and $l_t\geq 0$ taking the wage, the interest rate and the pension benefit as given, leads to the following first-order conditions:

(8) \begin{equation} c^o_{t+1}=\beta R c^y_t \end{equation}

(9) \begin{equation} \frac{\theta }{n_t}=\frac{w h_t (e_t+q)}{c^y_t} \end{equation}

(10) \begin{equation} \frac{w h_t n_t}{c^y_t}=\frac{\theta \epsilon }{e_t} \end{equation}

(11) \begin{equation} \frac{w\lambda h_t(1-\tau )-b_{t+1}}{c^o_{t+1}} \leq \frac{\delta }{1-l_{t+1}} (= \; \text{if} \; l_{t+1}\gt 0) \end{equation}

Equation (8) is the standard life cycle saving first-order condition. Equation (9) equalizes the marginal gain in utility of having a child to the marginal loss in utility due to the upbringing and education cost. Equation (10) balances the marginal loss in parental utility from investing in their children’s education against the marginal gain deriving from a higher future human capital. Finally in equation (11), agents choose to work in old age if the marginal utility of leisure in old age is sufficiently low.

After substituting $b_{t+1}$ from equation (7) optimal education, fertility, retirement, and consumption at an interior solution are given by:

(12) \begin{equation} e_t=\frac{q \epsilon }{1-\epsilon } \end{equation}

(13) \begin{equation} n_t=\frac{\theta (1-\epsilon )(1-\tau )(1+\lambda/R)}{q[1+\theta +\beta (1+\delta )]} \end{equation}

(14) \begin{equation} l_{t+1}=\frac{(1-\tau )\{Rqh_t[\lambda (1+\theta +\beta )-\beta R \delta ]-\tau \theta (\lambda +R)[w h_t q \epsilon/(1-\epsilon )]^\epsilon \}}{R\lambda q h_t[1+\theta +\beta (1+\delta )]} \end{equation}

(15) \begin{equation} c^y_t=\frac{w h_t(1-\tau )(R+\lambda )}{R[1+\theta +\beta (1+\delta )]} \end{equation}

Equation (13) shows that a lower depreciation of human capital in old age, that is, a higher value of $\lambda$ , positively affects fertility via a positive income effect (Mizuno and Yakita (2013)). In other words, a lower human capital decay increases lifetime labor income and therefore enables agents to increase consumption as well as the number of children when young. Moreover, we can observe that, in accordance with the quantity–quality trade-off literature as the return to the investment in education increases, that is, as $\epsilon$ increases for any $\epsilon \in (0,1)$ , the investment in education increases and fertility decreases. From equation (14), it is easy to note that, in accordance with Aísa et al. (2012) and Hirazawa and Yakita (2017), in the absence of pension benefits, agents choose to supply labor in old age if $\lambda$ is sufficiently high. On the other hand, the presence of a pension benefit leads to an ambiguous effect of $\lambda$ on $l_{t+1}$ . In fact if, on the one hand, a higher $\lambda$ is a stimulus to remain in the labor market, on the other hand, it increases pension benefits and therefore generates a greater incentive for retirement. Finally, a higher human capital level leads agents to retire later. In fact, if, on the one hand, agents with a higher human capital level earn a higher income in the first period of life, which could lead to an early retirement, they can also earn a higher income in old age, and this acts as an incentive to retire later (see Aísa et al. (2012)).

When the agents’ weight on the preference over leisure when old is sufficiently high, that is, $\delta \gt \underline{\delta }$ , with $\underline{\delta }=[Rq\lambda h_t(1+\theta +\beta )-(w h_t q \epsilon )^{\epsilon }(1-\epsilon )^{1-\epsilon }\tau (1+\lambda )]/R^2 \beta q h_t$ , then a corner solution for elderly labor supply arises, where $l_{t+1}=0$ and:

(16) \begin{equation} e_t=\frac{q \epsilon }{1-\epsilon } \end{equation}

(17) \begin{equation} n_t=\frac{(1-\epsilon )R\theta h_t(1-\tau )}{qRh_t(1+\beta +\theta )-\theta \tau (q \epsilon )^{\epsilon }(1-\epsilon )^{(1-\epsilon )}} \end{equation}

(18) \begin{equation} c^y_t=\frac{w h_t^2(1-\tau )qR}{qRh_t(1+\beta +\theta )-\theta \tau (q \epsilon )^{\epsilon }(1-\epsilon )^{(1-\epsilon )}} \end{equation}

2.3 The planning problem

In this section, we extend the social planner problem developed by van Groezen et al. (2003) by adding endogenous retirement. Thus, the social planner chooses consumption, education, fertility, and retirement age in order to maximize the utility of a dynastic family, subject to the resource constraint of the economy.

DEFINITION 2. The social welfare function is defined as the discounted sum of average lifetime utility of all current and future generations:

(19) \begin{equation} W_t=\sum _{i=t}^\infty \alpha ^{i-t}[\ln c^y_{i-1}+\theta \ln n_{i-1}h_{i}+\beta [\ln c^o_{i}+\delta \ln (1-l_{i})] ] \end{equation}

where $0\lt \alpha \lt 1$ is the social discount factor. ⁴

Assuming that capital does not depreciate, the total resources of the economy, comprising production and debt creation minus interest payments, are allocated to the consumption of the young and old, the cost of raising children $q$ , the expenditure for children’s education $e_t$ , and domestic investments:

(20) \begin{equation} c_t^y=Rk+w\!\left (h_t+\frac{\lambda h_{t-1}l_t}{n_{t-1}}\right )-kn_t+n_t d_{t+1}-d_t R-n_t w h_t(q+e_t)-\frac{c^o_t}{n_{t-1}} \end{equation}

where $k=K/N$ is the capital per young worker and $d_t$ is the per capita foreign debt of the country at time $t$ . ⁵

The first-order conditions of the social planning problem are therefore given by:

(21) \begin{equation} \frac{c^y_{t+1}}{c^y_{t}}=\frac{\alpha R}{n_t} \end{equation}

(22) \begin{equation} \frac{c^o_{t+1}}{c^y_{t}}=\beta R \end{equation}

(23) \begin{equation} \frac{c^y_t (\theta +\beta )}{n_t}=(q+e_t)wh_t+ k-d_{t+1}+\frac{\alpha \lambda w h_t l_{t+1}}{n^2_t} \end{equation}

(24) \begin{equation} \frac{\theta \epsilon c^y_t}{e_{t}}=n_t w h_t-\alpha w h'_{\!\!t+1}[1-(q+e_t)n_t]-\alpha ^2\frac{w \lambda h'_{\!\!t+1}l_{t+1} }{n_t} \end{equation}

(25) \begin{equation} \frac{w \lambda h_t}{c^o_{t+1}}\leq \frac{\delta }{1-l_{t+1}} (= \; \text{if} \; l_{t+1}\gt 0) \end{equation}

where $h'_{\!\!t+1}=\partial h_{t+1}/\partial e_t$ . Equations (21) and (22) show, respectively, the optimal intergenerational allocation, that is, the equality of the marginal utility of the young generation in two different periods, and the optimal intragenerational allocation, that is, the equal marginal utility of consumption of the young and old generations. Equation (23) states that the marginal benefit of having a child should be equal to the marginal loss in utility, including the wage, the time, and the education cost. Equation (24) equates the marginal cost of education with the marginal utility gain from higher human capital for children, which includes not only the conventional gains from the marginal product of human capital but also labor earnings in adulthood and old age for a given retirement period. Note that in old age, this effect is mitigated by the decay of human capital (Nishimura et al. (2018)). Equation (25) indicates that a corner solution for labor supply in old age arises if the marginal cost of leisure in old age is lower than its marginal utility.

For the solution to be a social optimum, we assume (for technical details see Appendix A):

ASSUMPTION 1. $\max \{\alpha _1,\tilde{\alpha _1}\}\lt \alpha \lt \tilde{\alpha _2}$ and $qR\lt \min \{\epsilon,1-\epsilon \}$

Thus, we can now characterize the social planner’s steady state:

PROPOSITION 1. If the preference for leisure time in old age is sufficiently low, that is, $\delta \leq \hat{\delta }$ , with $\hat{\delta }=\lambda [\alpha (1+\theta +\beta )(1+\alpha \epsilon )-\theta (1-\epsilon +\alpha \epsilon )]/\alpha \beta R (1-\epsilon +\alpha \epsilon -Rq)$ , then there exist an interior steady-state first-best optimum given by:

(26) \begin{equation} n^{**}=\alpha R \end{equation}

(27) \begin{equation} c^{y**}=\frac{\alpha w h^{**}\{(\lambda +R)[1-\epsilon (1-\alpha )]-R^2q\}}{R \{\epsilon \alpha ^2[1+\theta +\beta (1+\delta )]+\alpha [1+\theta (1-\epsilon )+\beta (1+\delta -\delta \epsilon )]-\theta (1-\epsilon )\}} \end{equation}

(28) \begin{equation} l^{**}=1- \frac{\beta \delta \alpha }{\lambda }\frac{(\lambda +R)[1-\epsilon (1-\alpha )]-R^2q}{\{\epsilon \alpha ^2[1+\theta +\beta (1+\delta )]+\alpha [1+\theta (1-\epsilon )+\beta (1+\delta -\delta \epsilon )]-\theta (1-\epsilon )\}} \end{equation}

(29) \begin{equation} e^{**}=\epsilon \frac{\alpha [(\lambda +R)(1+\theta +\beta )+R^2q(\beta \delta +\theta )]-R^2q\alpha ^2[1+\theta +\beta (1+\delta )]-R^2q\theta }{R^2 \{\epsilon \alpha ^2[1+\theta +\beta (1+\delta )]+\alpha [1+\theta (1-\epsilon )+\beta (1+\delta -\delta \epsilon )]-\theta (1-\epsilon )\}} \end{equation}

By contrast if $\delta \geq \hat{\delta }$ then a corner solution with full retirement arises:

(30) \begin{equation} n^{**}=\alpha R \end{equation}

(31) \begin{equation} c^{y**}=\frac{\alpha w h^{**}[1-qR-\epsilon (1-\alpha )]}{\epsilon \alpha ^2(1+\theta +\beta )+\alpha [1+\beta +\theta (1-\epsilon )]-\theta (1-\epsilon )} \end{equation}

(32) \begin{equation} e^{**}=\frac{\epsilon [\alpha (1+\theta +\beta +Rq\theta )-Rq\alpha ^2(1+\theta +\beta )-Rq\theta ]}{R[\epsilon \alpha ^2(1+\theta +\beta )+\alpha [1+\beta +\theta (1-\epsilon )]-\theta (1-\epsilon )]} \end{equation}

where $h^{**}={e^{**}}^{\epsilon }$ .

Proof. The proof follows immediately from the first-order condition of the social planning problem given by equations (21)–(25).

3. Optimum versus market allocation

Comparing equations (8)–(11) with equations (21)–(25), we can observe that while savings decisions of agents are consistent with a centralized economy, agents’ fertility, education, and labor supply choices differ from those of a central planner. In fact, as opposed to the central planner, individuals do not have the right incentives to choose the optimal fertility rate because they do not internalize the positive effect of each person’s fertility decisions on the future workforce and therefore on pension benefits. This is the familiar intergenerational transfer effect, which leads to a decentralized equilibrium with too few children.

In addition to this intergenerational transfer effect, the literature has also highlighted a counterbalancing effect called capital dilution, that is, parents do not take into account that a higher fertility rate, given the aggregate capital saved by the previous generation, lowers the capital per worker. In addition to these effects, the externalities associated with the “quality” of children and labor supply in old age have to be considered. In particular, in a framework with endogenous investment in education, the rate of return of a pay-as-you-go system depends not only on the fertility rate but also on human capital accumulation. However, as it is easy to note from equation (32) that the market solution differs from the social planner’s because, other things being equal, agents do not internalize this marginal gain from the investment in education on labor earnings in adulthood and old age. This leads to a decentralized equilibrium characterized by underinvestment in human capital. Finally, from equation (11), we can observe that the provision of social security decreases the relative price of leisure in old age leading to early retirement. This is because agents do not take into consideration the fact that a higher labor supply in old age, all else being equal, increases pension benefits, and therefore, from this point of view, the labor supply in old age is too low. On the other hand, agents also fail to consider that a higher labor supply in old age, for given aggregate savings and fertility, would decrease the capital labor ratio. However, in a small open economy, the capital labor ratio is fixed, and thus, if the labor supply in old age increases, savings and/or foreign debt should increase. This leads to a decentralized equilibrium with an excessive labor supply in old age. These results suggest that the government needs to redistribute resources across generations in order to achieve the first-best allocation in a decentralized economy.

3.1 Corner solution

Let us first analyze potential government policies to implement the first-best allocation in a decentralized economy in the case of a corner solution.

Government runs a balanced budget. For simplicity, we assume that it imposes two payroll taxes, $\tau$ and $\eta$ , the first to finance policies for the elderly and the second to finance policies for the children. The latter consists of a subsidy $s_N$ per child in order to finance a fraction of the child rearing costs $q$ and a subsidy $s_H$ in order to cover a share of the educational cost. Therefore, agent’s budget constraint in adulthood becomes (see Stauvermann and Kumar (2018)):

(33) \begin{equation} c^y_t= wh_t[1-\tau -\eta -q(1-s_N)n_t-e_t(1-s_H)n_t]-s_t \end{equation}

Assuming a balanced budget regime in each period, the following equation in per capita terms must hold

(34) \begin{equation} (s_N q+s_H e_t)n_t=\eta \end{equation}

From equations (4), (6), (7), (33), and (34), the optimal investment in education, fertility, and consumption at the steady state are as follows:

(35) \begin{equation} e^*=\frac{(1-s_N)q\epsilon }{(1-s_H)(1-\epsilon )} \end{equation}

(36) \begin{equation} n^*=\frac{(1-\tau )R(1-\epsilon )(1-s_H)\theta }{qR\left \{(1+\beta )(1-s_H)(1-s_N)+\theta [1-\epsilon s_N-s_H(1-\epsilon )]\right \}-\theta \tau (1-s_H)(1-\epsilon )} \end{equation}

and:

(37) \begin{equation} c^{y*}=\frac{wh^*(1-\tau )(1-s_N)(1-s_H)Rq}{\left \{(1+\beta )(1-s_H)(1-s_N)+\theta [1-\epsilon s_N-s_H(1-\epsilon )]\right \}-\theta \tau (1-s_H)(1-\epsilon )} \end{equation}

Note that as a result of the quantity–quality trade-off between the number of children and their level of education, the introduction of child benefit increases the number of children but negatively affects the optimal investment in education (Stauvermann and Kumar (2018)). In fact, if, on the one hand, the introduction of a child allowance reduces the direct cost of child rearing, on the other hand, it increases the relative cost of education, leading parents to decrease the investments in their children’s education. For the same reasons, the introduction of an educational subsidy leads to a decline in the number of children and increases the investment in human capital. This suggests that neither education subsidization nor child benefit can achieve the social optimum on their own. The following proposition characterizes the optimal policy combination which allows to realize the first-best solution in a market economy.

PROPOSITION 2. Assuming $\alpha \geq \alpha _0$ , the optimal government policies, such that the market allocation is the same as the socially optimal allocation, are $\tau =\tau _c^*$ , $s_N=s_{N_c}^*$ , and $s_H=s_{H_c}^*$ :

(38) \begin{equation} \tau _c^*=\dfrac{\alpha [(1+\beta )\epsilon +Rq(1+\beta +\theta )]-(1-\epsilon )\theta }{\alpha ^2\epsilon (1+\beta +\theta )+\alpha [1+\beta +\theta (1-\epsilon )]-\theta (1-\epsilon )} \end{equation}

(39) \begin{equation} s_{N_c}^*=\dfrac{Rq\alpha ^2\epsilon (1+\theta +\beta )+\alpha \{Rq[1+\beta +\theta (1-\epsilon )]-\theta \epsilon (1-\epsilon )\}-\theta (1-\epsilon )^2}{Rq\{\alpha ^2\epsilon (1+\theta +\beta )+\alpha [1+\beta +\theta (1-\epsilon )]-\theta (1-\epsilon )]\}} \end{equation}

and:

(40) \begin{equation} s_{H_c}^*=\dfrac{\alpha [1+\beta +Rq\theta +\theta (1-\epsilon )]-Rq\alpha ^2(1+\theta +\beta )-\theta (1-\epsilon )}{\alpha (1+\theta +\beta +Rq\theta )-Rq\alpha ^2(1+\theta +\beta )-Rq\theta } \end{equation}

where under Assumption 1 $s_{N_c}^*\lt 1$ and $s_{H_c}^*\lt 1$ .

Proof. The first-best solution given by equations (30), (31), and (32) coincides with the market solution given by equations (35), (36), and (37) if $\tau =\tau _c^*$ , $s_N=s_{N_c}^*$ , and $s_H=s_{H_c}^*$ .

LEMMA 1. For a given value of the social discount factor, that is, $\alpha ^0=(1-\epsilon )\theta/[(1+\beta )\epsilon +Rq(1+\beta +\theta )]$ , the optimal level of consumption in a market economy coincides with the first-best solution and therefore $\tau _c^*=0$ and $\eta ^*=0$ . However, the quantity–quality trade-off between the number of children and their level of education still holds, particularly without policy intervention $n^*\gt n^{**}$ and $e^*\lt e^{**}$ , and therefore a tax on children, that is, $s_{N_c}\lt 0$ , and an eduction subsidy $s_{H_c}\gt 0$ are needed to achieve the first-best solutions. ⁶ For all other values of $\alpha \gt \alpha _0$ , possible combinations of pension, that is, $\tau _c^{*}\gt 0$ , education subsidy, and tax on or a subsidy for children arise.

Proof. Under Assumption 1, from equations (39) and (40) $s_{N_c}^*\gt 0$ if $\alpha \gt \hat{\alpha _c}$ with $\hat{\alpha _c}\gt \alpha _0$ and $s_{H_c}^*\gt 0$ if $\alpha \gt \bar{\alpha _c}$ with $\bar{\alpha _c}\lt \alpha _0$ . See Appendix B for more technical details.

3.2 Interior solution

We now derive socially optimal government policies which allow us to replicate the first-best allocation in a competitive equilibrium when agents choose to supply labor in old age.

At the interior solution, education subsidization and child benefit are not enough to ensure that the elderly labor supply reaches the first-best level; thus, the government needs to introduce a subsidy on elderly labor supply, that is, $\tau ^o\gt 0$ . This implies that agents’ budget constraints in adulthood are given by equation (33) and, in old age, by:

(41) \begin{equation} c^o_{t+1}=Rs_t+w \lambda h_t l_{t+1}(1+\tau ^o)+b_{t+1}(1-l_{t+1}) \end{equation}

Under the assumption that the government uses a fraction $0\lt a\lt 1$ of social security tax revenues for pension benefits and a fraction $1-a$ for elderly labor supply subsidy, the internal allocation of government expenditure is, therefore, given by:

(42) \begin{equation} b_{t+1}=\frac{a \tau w n_t h_{t+1}}{1-l_{t+1}} \end{equation}

and:

(43) \begin{equation} \tau ^o=\frac{(1-a)\tau n_t h_{t+1}}{\lambda h_tl_{t+1}} \end{equation}

From equations (4), (33), (34), (41), (42), and (43), the optimal investment in education, fertility, and consumption are as follows:

(44) \begin{equation} e^*=\frac{(1-s_N)q\epsilon }{(1-s_H)(1-\epsilon )} \end{equation}

(45) \begin{equation} n^*=\frac{\theta (1-s_H)(1-\epsilon )[R(1-\tau )+\lambda (1+\tau ^o)]}{qR\{(1-s_N)(1-s_H)[1+\beta (1+\delta )]+\Psi \}} \end{equation}

(46) \begin{equation} c^{y*}=\frac{wh_t(1-s_N)(1-s_H)[R(1-\tau )+\lambda (1+\tau ^o)]}{R\{(1-s_N)(1-s_H)[1+\beta (1+\delta )]+\Psi \}} \end{equation}

(47) \begin{equation} l^*=\frac{(1+\tau ^o)\lambda \{(1-s_H)[(1+\beta )(1-s_N)qR-a\tau \theta (1-\epsilon )]+ qR\Psi \}-R(1-\tau )(1-s_H)[\delta \beta q(1-s_N)R+a\tau \theta (1-\epsilon )]}{R\lambda (1+\tau ^o)q\{(1-s_N)(1-s_H)[1+(1+\delta )\beta ]+\Psi \}} \end{equation}

where $\Psi =\theta [1-\epsilon s_N-(1-\epsilon )s_H]$ .

We therefore derive socially optimal government policies such that the market equilibrium allocation in Definition 1 coincides with that preferred by the social planner in Proposition 1.

PROPOSITION 3. Assuming $\alpha \geq \tilde{\alpha ^0}$ , in equilibrium, the socially optimal government policy is characterized by $\tau =\tau ^*$ , $a=a^*$ , $s_H=s_H^*$ , and $s_N=s_N^*$ . Human capital decay unambiguously increases $s_N^*$ and $a^*$ , while its effect on $s^*_H$ is ambiguous:

(48) \begin{equation} \tau ^*=\frac{\alpha \{(1+\beta )(\lambda +R)\epsilon +R^2q[(1+\delta )\beta +1+\theta ]\}-\theta (1-\epsilon )(\lambda +R)}{R T} \end{equation}

(49) \begin{equation} a^*=\frac{\alpha \beta \delta \{(\lambda +R)[1-\epsilon (1-\alpha )]-R^2 q\}}{\lambda T} \end{equation}

(50) \begin{equation} s_N^*=\frac{qR^2T-(1-\epsilon )\theta \{ (\lambda +R)[1-\epsilon (1-\alpha )]-R^2q\}}{qR^2T} \end{equation}

(51) \begin{equation} s_H^*=\frac{\alpha \{(\lambda +R)[1+\beta +\theta (1-\epsilon )]+R^2q(\beta \delta +\theta )\}-R^2q\alpha ^2[1+\theta +\beta (1+\delta )]-(\lambda +R)(1-\epsilon )\theta }{\alpha [(\lambda +R)(1+\theta +\beta )+R^2q(\beta \delta +\theta )]-R^2q\alpha ^2[1+\theta +\beta (1+\delta )]-R^2q\theta } \end{equation}

where Assumption 1 implies that $\alpha [R(1+\theta +\beta +Rq\theta )+\lambda (1+\theta +\beta )+R^2q\beta \delta (1-\alpha )]-R^2q\alpha ^2(1+\theta +\beta )\gt R^2q\theta$ , $T=\epsilon \alpha ^2[1+\theta +\beta (1+\delta )]+\alpha [1+\beta +\theta (1-\epsilon )(1+\delta )]-\theta (1-\epsilon )\gt 0$ , $s_{N}^*\lt 1$ and $s_{H}^*\lt 1$ .

Proof. The first-best solution given by equations (26)–(29) coincides with the market solution given by equations (44)–(47) if $\tau =\tau ^*$ , $a=a^*$ , $s_H=s_H^*$ , and $s_N=s_N^*$ .

LEMMA 2. Similarly to the corner solution, we can see that for a given value of the social discount factor, that is, $\alpha =\tilde{\alpha ^0}=\theta (1-\epsilon )(\lambda +R)/\{R^2 q[(1+\delta )\beta +1+\theta ] + \epsilon (1+\beta )(\lambda +R)\}$ , the optimal level of consumption and elderly labor supply in a market economy coincide with the first-best solution and therefore $\tau ^*=0$ , $\tau ^{o*}=0$ , and $\eta ^*=0$ . However, fertility and education differ from the socially optimal ones and therefore a tax on children, that is, $s_N\lt 0$ , together with an eduction subsidy, that is, $s_H\gt 0$ , are both required to obtain the first-best solutions. ⁷ For all other values of $\alpha \gt \tilde{\alpha ^0}$ , possible combinations of pension, that is, $\tau ^{*}\gt 0$ , education subsidy and tax on or a subsidy for children arise.

Proof. Under Assumption 1, from equations (39) and (40), $s_{N}^*\gt 0$ if $\alpha \gt \hat{\alpha }$ with $\hat{\alpha }\gt \alpha _0$ and $s_{H}^*\gt 0$ if $\alpha \gt \bar{\alpha }$ with $\bar{\alpha }\lt \alpha _0$ . See Appendix B for technical details.

Human capital decay is a key parameter in this model, and its variation crucially affects policy combinations at the interior solution. In fact, if, ceteris paribus, human capital decay falls, child benefits decrease. In particular, from equation (50), it is easy to note that child benefit becomes a tax when human capital decay is sufficiently low, that is, $\lambda \gt \lambda _{SN}$ . ⁸ The intuition behind this result is that high human capital productivity maintained in the second period of life increases fertility through the positive income effect, as can be seen from equation (13). Thus, a sufficiently low human capital decay leads to a decentralized equilibrium with too many children, and therefore a tax on children is required in order to reach the first-best solution. As for education, the central planner, differently from the decentralized economy, considers the effect of children’s education on their labor income. Hence, when there is less decay, since the marginal productivity of education increases for the elderly, optimal education should be higher. As can be seen in equation (35), this can be achieved by changes in child or education benefit (where $s_N\lt s_H$ ). Given that $s_N$ decreases, $s_H$ can, therefore, increase or decrease. Moreover, from equation (49), it is evident that that a lower human capital decay reduces the fraction of social security tax revenues devoted to pensions, that is, $\partial{a^*}/\partial{\lambda }\lt 0$ . In Section 4, we carry out a sensitivity analysis to study the overall effect of a change in human capital decay.

4. Numerical examples

This section provides a quantitative analysis of optimal policy combinations as discussed in the previous section for reasonable values of the parameters.

We set the parameter values by using either the parametrization of other quantitative studies or by matching the data of OECD countries in 2019 where available. In particular, we assume that one period lasts 30 years. The parameter $\psi$ , that is, the capital share in added value, is set at $0.3$ as usual. The annual interest rate is set at $1.2\%$ per year and yields $R=1.4$ over a generation (30 years). The productivity level $A$ is a scale parameter and equal to $1.5$ . Given $R$ , $\psi$ , and $A$ , the capital:labor ratio is $k=0.2$ , and this means that $w=0.6$ . As in Blackburn and Cipriani (2002), the annual discount factor is $0.99$ and therefore $\beta = (0.99)^{30}=0.74$ over a generation.

We set the child rearing cost $q$ at $0.095$ , in accordance with Strulik (2004). The initial social security tax rate is set at $0.15$ . In accordance with De La Croix and Licandro (2013), we set the elasticity of human capital in relation to education $\epsilon$ at $0.2$ and De la Croix (2012) we set $\theta =0.27$ .

According to Ferreira and de Abreu Pessôa (2007), the rate at which labor productivity falls until the worker dies is about $1.5\%$ ; thus, we set as a benchmark $\lambda =0.9$ . In accordance with Miyazaki (2019), we set, in the benchmark economy, the weight of leisure related to consumption in old age, $\delta$ , equal to $0.2$ . Given this baseline parameter set, we get $\alpha _1=0.1$ , $\tilde{\alpha _1}=0.017$ , and $\tilde{\alpha _2}=7.6$ . Thus, in order to satisfy Assumption 1, we choose $\alpha =0.8$ which is higher than both $\alpha ^0=0.35$ and $\tilde{\alpha ^0}=0.4$ . A value of $\alpha \gt \alpha ^0$ implies that $\underline{\delta } \lt \hat{\delta }$ . ⁹ This allow us to characterize three scenarios: (i) the benchmark economy of the competitive equilibrium, without family policies other than pension; (ii) an economy where the optimal policy mix in order to get the first best is characterized by an education subsidy and a tax/subsidy on fertility; (iii) an economy where the optimal policy combination in order to achieve the social optimum is characterized by an education subsidy, a tax/subsidy on fertility, and a subsidy on elderly labor supply.

These scenarios were chosen because they are reminiscent of some of the principal policies that have been put into place over the past few years by various governments with the aim of reinforcing the financial sustainability of their respective pension systems. In fact, over the past two decades, the majority of OECD countries have raised the retirement age as a common reform (see Cipriani and Fioroni (2022)). Moreover, reducing incentives to retire early and rewarding employment at an older age to increase retirement age have been put in place by various countries (OECD (2019b)). Some countries, such as Belgium, Canada, and Denmark, have recently introduced incentives to enable elderly workers to either work longer or sign up to a variety of flexible retirement schemes (OECD (2019a)) .

Finally, many OECD countries have introduced a number of different incentives to cover both maternity leave and childcare, including credits based systems, aimed at subsidizing periods of absence from the labor market (OECD (2019a)). In reality, the distinction between the policies we are analyzing is not very clear and we find, in many countries, the existence of more complex scenarios. Thus, the scope of our study is to shed some light on the potential welfare impact of recent pension reforms without going into a comprehensive analysis of those complex reforms.

Note that in Policy 2, the introduction of a subsidy for elderly labor increases the marginal cost of leisure, thus increasing decentralized fertility above the optimum and requiring a child tax. We can also note that the introduction of a subsidy for elderly labor supply enables the economy to reach a higher level of welfare. In particular, in order to give a measure of welfare change, we calculate the wealth equivalent (i.e., $WE$ ). Specifically, this is a measure of the percentage increase in full lifetime resources needed in the initial scenario, without the policy, to achieve the level of utility obtained after the introduction of the policy (Auerbach & Kotlikoff, 1987), Fehr et al. (2017)). Overall, we find that welfare gains from the benchmark economy to an economy characterized by child subsidies combined with a subsidy to elderly labor supply, measured in terms of wealth equivalent, amount to 4.26, whereas the sole introduction of child subsidies provides a welfare gain equal to 0.23 (see Table 1).

Table 1. Welfare comparisons

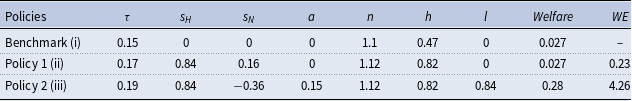
Note: WE is the wealth equivalent.

A key parameter in this model is the human capital decay; therefore, Table 2 presents a sensitivity analysis of socially optimal first-best policies and welfare variations measured in terms of wealth equivalent to variations in the degree of human capital decay. As discussed in the previous section, there is a positive relationship between human capital decay and child subsidy. In fact, a very high human capital decay makes the introduction of a child benefit necessary in order to achieve the first-best solution. In particular, with our parameters, set $\lambda _{SN}=0.33$ .

Table 2. Sensitivity analysis

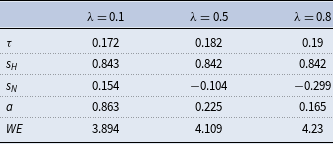
Finally, the reduction in human capital decay increases the welfare gain measured in terms of wealth equivalent derived from the introduction of flexible retirement. Thus, the variation of human capital decay crucially affects policy combinations and welfare. As emphasized by the OECD (OECD (2019b)), preventing human capital decay is one of the key policy recommendations to promote more and better jobs for older workers and therefore the sustainability of pension systems. In recent years, some countries have promoted programs to decrease the cost of training older workers compared to other employees and to boost the interest and motivation of the elderly to invest in their own skills. Australia and Korea, for example, offer targeted career advice and guidance services in order help the elderly understand the benefits of their investment in improving their skill. Moreover, many countries are setting up initiatives to recognize existing skills through validation and certification to encourage the participation of older workers in training (see OECD (2019b)).

5. Conclusion

Our objective in this paper is to contribute to the recent literature studying overlapping generations models of endogenous demographics and pensions by including human capital and child policies together with endogenous retirement. We argue that this is an important extension to the theoretical literature, since pension policies and child policies are clearly interlinked, as recognized by a well-established literature dating back several decades. It is also relevant from a policy perspective, since it gives rise to conclusions that contribute to the debate on pension reforms undertaken by many countries in the recent past. However, this does not imply that any of our policy results should be taken in isolation; in particular, we certainly do not claim that a tax on children should be conceived in isolation.

Using a standard overlapping generations model in an open economy framework with a pay-as-you-go pension system and full retirement, we demonstrate that decentralizing the optimum requires a subsidy on educational investment and tax on or a subsidy for children. It also requires a subsidy for the labor supply of the elderly workers in case of partial retirement. Human capital decay is a key parameter in this model, and we discuss its crucial role in affecting the optimal policy mix.

The model could be extended in several directions. One avenue could be the inclusion of endogenous growth in the model, which here could have human capital as its obvious engine. Together with this, one could consider the endogeneity of longevity and therefore the feedback from growth in longevity to optimal retirement and fertility. In the context of a fully endogenous aging population, a longer working life is even more important to preserve the stability of the pension system and to ensure acceptable replacement ratios. Finally, one could think of policies to retrain older workers in order to combat the decay of human capital. OECD countries vary quite substantially in their investments in the training of elderly workers. In our model, the parameter that captures the fraction of youth productivity preserved by the older workers is fixed, but this could be considered as affected by government policies.