Relative transmissibility of hand, foot and mouth disease from male to female individuals

Abstract

Hand, foot and mouth disease (HFMD) has spread widely and leads to high disease burden in many countries. However, relative transmissibility from male to female individuals remains unclear. HFMD surveillance database was built in Shenzhen City from 2013 to 2017. An intersex transmission susceptible–infectious–recovered model was developed to calculate the transmission relative rate among male individuals, among female individuals, from male to female and from female to male. Two indicators, ratio of transmission relative rate (R_β) and relative transmissibility index (RTI), were developed to assess the relative transmissibility of male vs. female. During the study period, 270 347 HFMD cases were reported in the city, among which 16 were death cases with a fatality of 0.0059%. Reported incidence of total cases, male cases and female cases was 0.0057 (range: 0.0036–0.0058), 0.0052 (range: 0.0032–0.0053) and 0.0044 (range: 0.0026–0.0047), respectively. The difference was statistically significant between male and female (t = 3.046, P = 0.002). R_β of male vs. female, female vs. female, from female to male vs. female and from male to female vs. female was 7.69, 1.00, 1.74 and 7.13, respectively. RTI of male vs. female, female vs. female, from female to male vs. female and from male to female vs. female was 3.08, 1.00, 1.88 and 1.43, respectively. Transmissibility of HFMD is different between male and female individuals. Male cases seem to be more transmissible than female.

Introduction

Hand, foot and mouth disease (HFMD) is an important infectious disease and leads to high disease burden in many countries [1–6]. There are over 20 types of enteroviruses leading to HFMD [1]. The main pathogens of the disease are Enterovirus 71 (EV71) and Coxsackievirus A16 (CV-A16). The complexity of the pathogens leads to difficulty in controlling the disease. Therefore, it is essential to understand the transmissibility of HFMD. Understanding the transmissibility of an infectious disease could help health department to forecast the attack rate and assess the effectiveness of countermeasures to contain the spread of the disease [7–12].

Several mathematical models have been developed to calculate the transmissibility of HFMD, and the results of these research studies showed that the transmissibility of HFMD has a wide-span range. The estimated basic reproduction number (R ₀) was 1.44 in Bangkok, Thailand, 2016 [2]. Results of a mathematical model study showed that the average R ₀ of three different strains of EV71 from Japan, Malaysia and Thailand were 37.35 ± 8.99, 8.37 ± 0.82 and 6.75 ± 0.16, respectively [13]. Another study showed that the median R ₀ of CV-A6, CV-A16 and EV-A71 in Singapore was estimated to be 5.04 (interquartile range (IQR) 3.57–5.16), 2.42 (IQR 1.85–3.36) and 3.50 (IQR 2.36–4.53), respectively [14]. Wang et al. [15] employed a susceptible–infectious–recovered (SIR) model to calculate the transmissibility of HFMD in 2008 and 2009 in China, and found that the effective reproductive number had a median of 1.4 (range: 1.4–1.6) in spring and stayed below 1.2 in other seasons. Takahashi et al. [16] found that the transmissibility of the disease was much higher from 2009 to 2013 in China. The R ₀ was 26.63 (IQR: 23.14–30.40) for Enterovirus 71 (EV71) and 27.13 (IQR: 23.15–31.34) for Coxsackievirus A16 (CV-A16) estimated by a time series SIR (TSIR) model [16]. Calculated the case-based data from 2009 to 2012 by the TSIR model, the median reproductive number of HFMD was 4.62 (IQR: 3.91–5.82) in Guangdong Province and 3.11 (IQR: 2.44–4.43) in Shenzhen City, respectively [17]. Undoubtedly, these research studies about the transmissibility of different pathogens in different areas have provided much epidemiological information for understanding and controlling HFMD.

However, significance difference in the incidence exists between male and female [18–20]. Wang et al. [15] found that the attack rate of male was higher than that of female in 2008 and 2009 in China. The significant gender differences reveals that the transmissibility of male might different to that of female. Unfortunately, the relative transmissibility from male to female individuals remains unclear. In this study, we first built case-based epidemiological data of reported HFMD cases from 2013 to 2017 in Shenzhen City, Guangdong Province, China. An intersex transmission SIR model was then developed according to the natural history and the intersex transmission mechanism of the disease to fit the epidemiological data. Finally we developed a relative transmissibility index (RTI) calculated by the model to assess the relative transmissibility of male vs. female.

Materials and methods

Data collection

A dataset of reported HFMD cases (clinically diagnosed cases and confirmed cases) and population information, collected from the Chinese Disease Control and Prevention Information System, was built in Shenzhen City from February 2013 to December 2017. The illness onset date and sex (male or female) of each case were collected. The population information included number of male and female individuals, birth rate and death rate of the population. The city which locates in the south China is a large city in Guangdong Province. It has a population of more than 12 million inhabitants and has a median birth rate of 18.40 per 1000 people (range: 17.48 per 1000 people to 19.94 per 1000 people) and median death rate of 6.72 per 1000 people (range: 6.63 per 1000 people to 9.72 per 1000 people) from 2013 to 2017.

The intersex transmission model

An intersex transmission SIR model was developed according to the natural history of HFMD and the mechanism of the transmission between male and female individuals (Fig. 1).

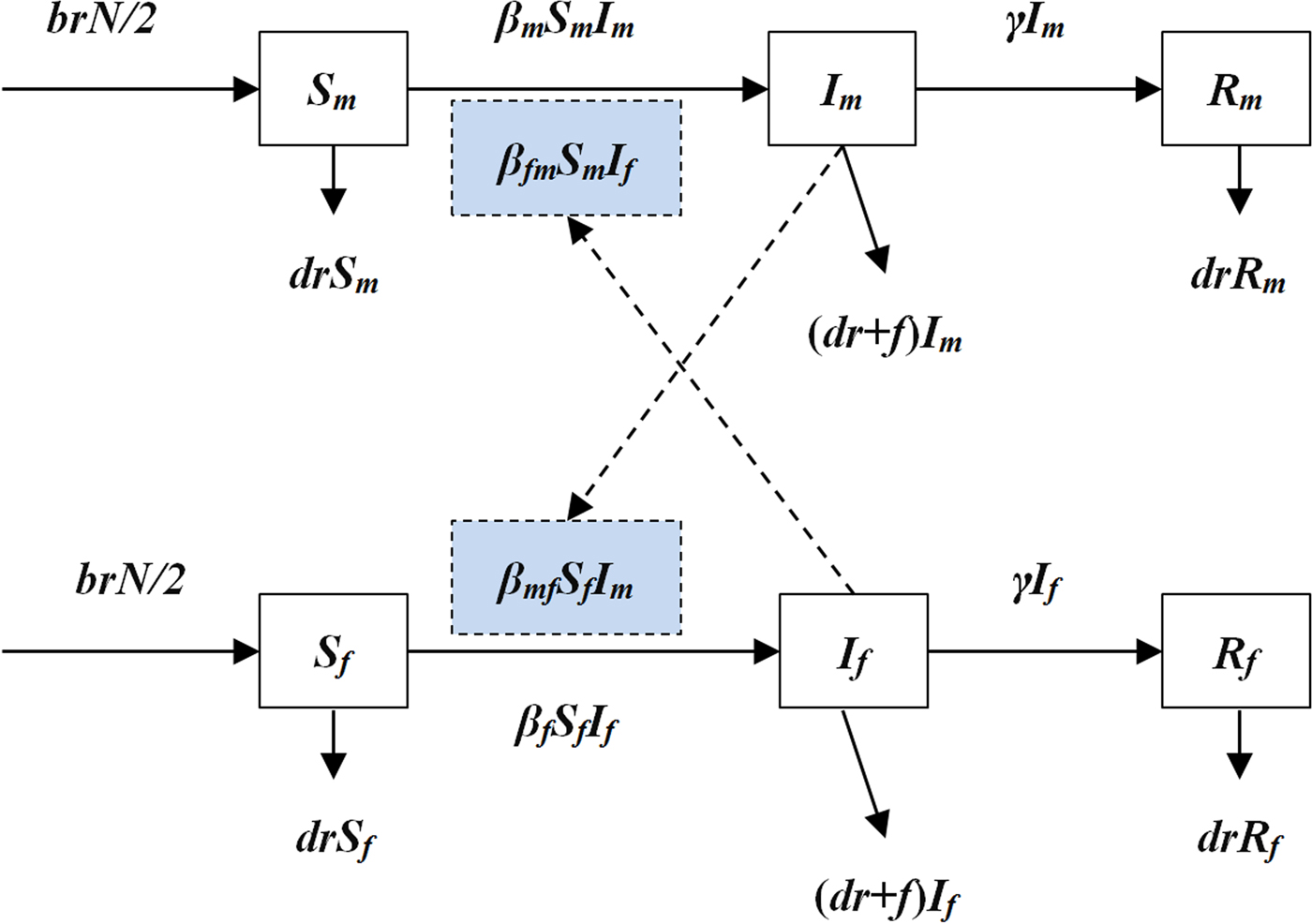
Fig. 1. The diagram of intersex transmission SIR model of HFMD.

In the model, we assumed that: (a) transmission relative rate among male and female individuals was β _m and β _f, respectively and (b) transmission relative rate from male to female was β _mf and from female to male was β _fm. Therefore, the transmission model was shown as follows:

$$\displaystyle{{{\rm d}S_{\rm m}} \over {{\rm d}t}} = \displaystyle{{brN} \over 2}-\beta _{\rm m}S_{\rm m}I_{\rm m}-\beta _{{\rm fm}}S_{\rm m}I_{\rm f}-drS_{\rm m}$$

$$\displaystyle{{{\rm d}I_{\rm m}} \over {{\rm d}t}} = \beta _{\rm m}S_{\rm m}I_{\rm m} + \beta _{{\rm fm}}S_{\rm m}I_{\rm f}-\lpar {\gamma + dr + f} \rpar I_{\rm m}$$

$$\displaystyle{{{\rm d}R_{\rm m}} \over {{\rm d}t}} = \gamma I_{\rm m}-drR_{\rm m}$$

$$\displaystyle{{{\rm d}S_{\rm f}} \over {{\rm d}t}} = \displaystyle{{brN} \over 2}-\beta _{\rm f}S_{\rm f}I_{\rm f}-\beta _{{\rm mf}}S_{\rm f}I_{\rm m}-drS_{\rm f}$$

$$\displaystyle{{{\rm d}I_{\rm f}} \over {{\rm d}t}} = \beta _{\rm f}S_{\rm f}I_{\rm f} + \beta _{{\rm mf}}S_{\rm f}I_{\rm m}-\lpar {\gamma + dr + f} \rpar I_{\rm f}$$

$$\displaystyle{{{\rm d}R_{\rm f}} \over {{\rm d}t}} = \gamma I_{\rm f}-drR_{\rm f}$$

$$N = S_{\rm m} + I_{\rm m} + R_{\rm m} + S_{\rm f} + I_{\rm f} + R_{\rm f}$$

In the above equations, S, I and R refer to susceptible individuals, infectious individuals and recovered individuals, respectively. The subscripts m and f refer to male and female. N refers to the number of the whole population. Parameters br, dr, f, β and γ refer to natural birth rate of the population, death rate of the population, fatality of HFMD, transmission relative rate and recovered relative rate, respectively.

Parameter estimation

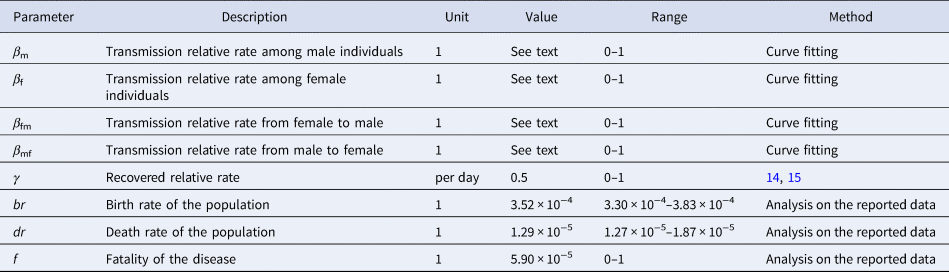
There were eight parameters (β _m, β _f, β _mf, β _fm, br, dr, f and γ) in the model (Table 1). Parameters br, dr and f were calculated from the collected data. According to the yearly values of br and dr, we calculated the weekly values of the two parameters. Therefore, the weekly value of br and dr was 0.000352 (range: 0.000330–0.000383) and 0.0000129 (range: 0.0000127–0.0000187), respectively. According to the published study [16, 17], the infectious period of HFMD was about 2 weeks, therefore γ = 0.5. The collected data of reported HFMD cases were employed to fit the SIR model to calculate β _m, β _f, β _mf and β _fm in each epidemic cycle.

Table 1. Parameter definitions and values

Indicators to assess the relative transmissibility of male vs. female

Two indicators, ratio of transmission relative rate (R _β) and RTI, were developed to assess the relative transmissibility of male vs. female. Let i = 1, 2, 3 and 4 refers to transmissibility among male individuals, among female individuals, from female to male and from male to female, respectively. The subscript j refers to the compared group, and was set as transmissibility among female individuals in this study. Therefore, four scenarios were simulated as M vs. F, F vs. F, FM vs. F and MF vs. F, where M, F, FM and MF refer to male, female, from female to male and from male to female, respectively. The equations to calculate R _β and RTI were shown as follows:

$$R_\beta = \displaystyle{{\beta _i} \over {\beta _j}}$$

$${\rm RT}{\rm I}_i = \displaystyle{{PR_i} \over {PR_j}}$$

$$PR_i = \displaystyle{{N_0-N_i} \over {N_0}} \times 100\%$$

In the above equations, PR _i, N ₀ and N _i refer to percentage of reduction under different intervention scenarios (β _m = 0, β _f = 0, β _fm = 0 and β _mf = 0), number of cases under the condition that no intervention was adopted and number of cases under the condition that four intervention scenarios (β _m = 0, β _f = 0, β _fm = 0 and β _mf = 0) were simulated, respectively.

Statistical analysis

Berkeley Madonna 8.3.18 (developed by Robert Macey and George Oster of the University of California at Berkeley. Copyright ©1993–2001 Robert I. Macey & George F. Oster) was employed to run the model and least root mean square was adopted to assess goodness of fit. SPSS 13.0 (IBM Corp., Armonk, NY, USA) was employed to run the t test between male and female and Kruskal–Wallis test among β _m, β _f, β _mf and β _fm.

Results

Epidemiological characteristics of reported HFMD cases

From 2013 to 2017, 270 347 HFMD cases (including 162 757 male cases and 107 590 female cases) were reported in Shenzhen City, among which 16 were death cases with a fatality of 0.0059%. Reported incidence of total cases, male cases and female cases increased yearly with a median value of 0.0057 (range: 0.0036–0.0058), 0.0052 (range: 0.0032–0.0053) and 0.0044 (range: 0.0026–0.0047), respectively (Fig. 2).

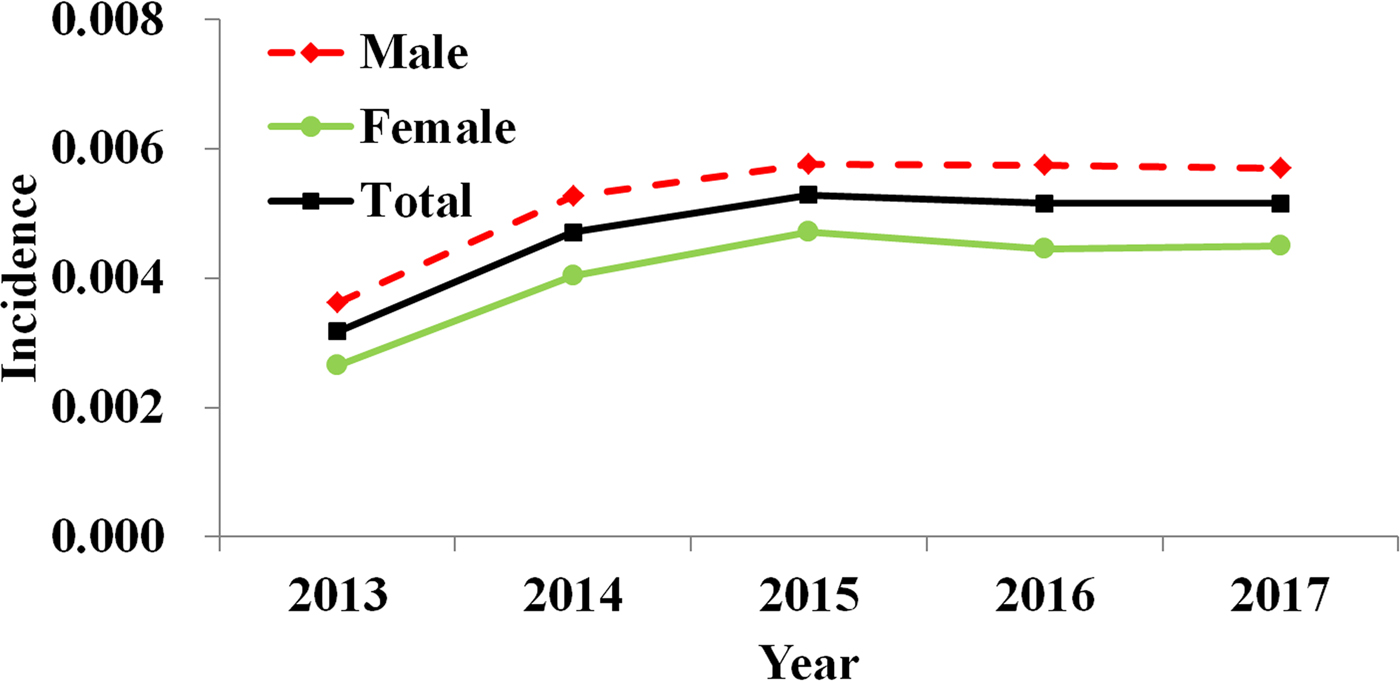
Fig. 2. Yearly reported incidence of HFMD in Shenzhen City, 2013 to 2017.

By analysing the weekly reported data, almost two epidemic cycles were observed at the turn of seasons from spring to summer and from summer to autumn in a year. These cycles were observed from both male and female cases. However, the reported incidence of male cases was slightly higher than female (Fig. 3). The difference of weekly incidence was statistically significant between male and female (t = 3.046, P = 0.002).

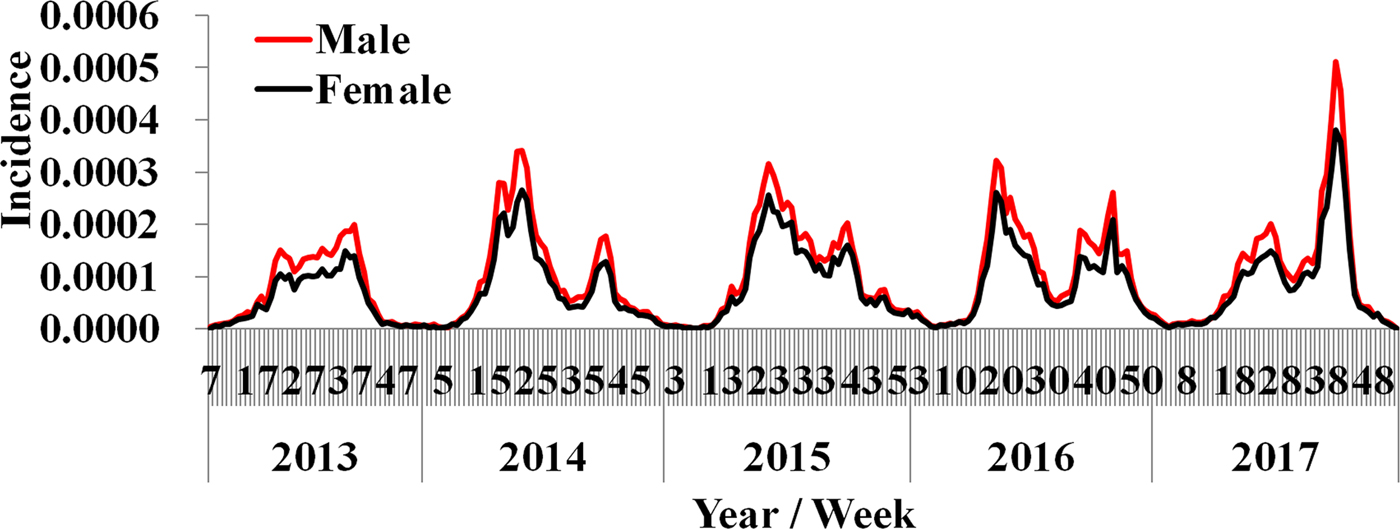
Fig. 3. Weekly reported incidence of HFMD in Shenzhen City from week 7, 2013 to week 53, 2017.

Curve fitting results

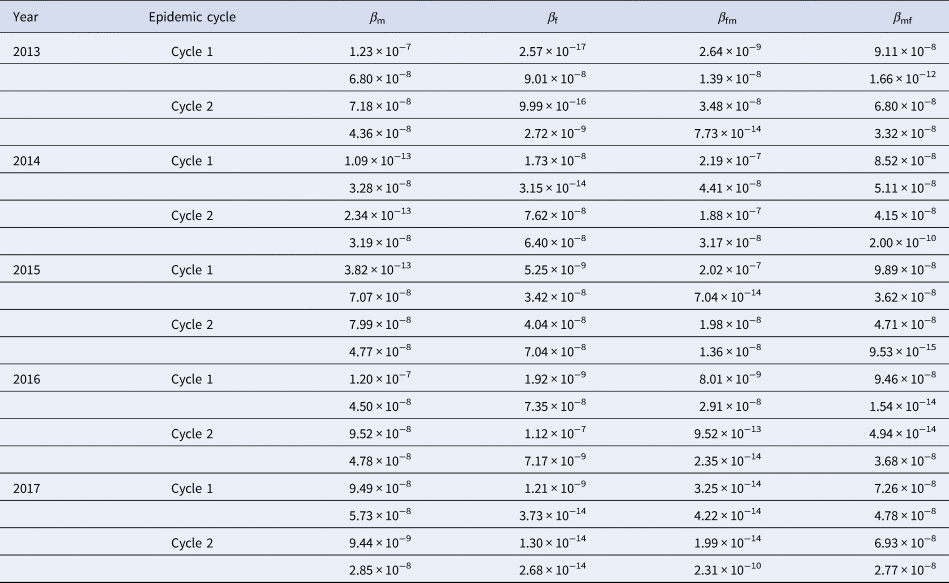
Results of curve fitting showed that the SIR model fitted the data well (Fig. 4). Four β values were calculated in a year, because there were two epidemic cycles in a year and ascending period and descending period of an epidemic cycle had different β values. All the values inner and between male and female individuals are shown in Table 2. The median value of β _m, β _f, β _mf and β _fm was 4.78 × 10⁻⁸ (range: 1.09 × 10⁻¹³–1.23 × 10⁻⁷), 6.21 × 10⁻⁹ (range: 2.57 × 10⁻¹⁷–1.12 × 10⁻⁷), 1.08 × 10⁻⁸ (range: 1.99 × 10⁻¹⁴–2.19 × 10⁻⁷) and 4.43 × 10⁻⁸ (range: 9.53 × 10⁻¹⁵–9.89 × 10⁻⁸), respectively. The results of Kruskal–Wallis test showed that the difference among β _m, β _f, β _mf and β _fm was statistically significant (χ ² = 7.938, P = 0.047). Therefore, R _β of M vs. F, F vs. F, FM vs. F and MF vs. F was 7.69, 1.00, 1.74 and 7.13, respectively.

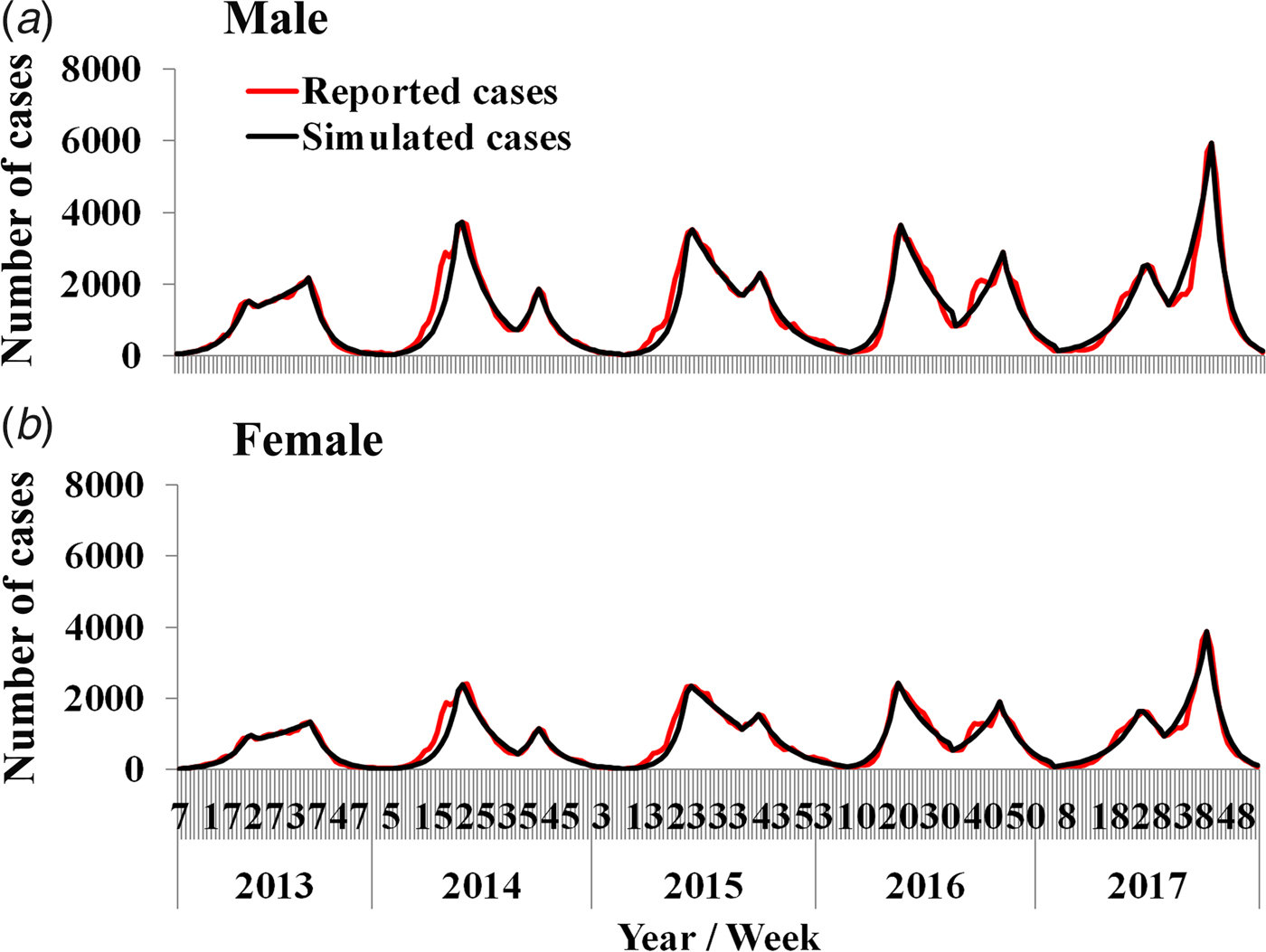
Fig. 4. Curve fitting results run by the intersex transmission SIR model to weekly reported HFMD cases.

Table 2. Transmission relative rate in epidemic cycle from 2013 to 2017 in Shenzhen City

Relative transmissibility

The simulation results showed that the 5-year-average number of cases was 54 026 among which 32 524 were male cases and 21 502 were female cases.

If we set β _m = 0, the 5-year-average value of total cases was reduced 54.27% [(54 026 − 24 706)/54 026 × 100%] and male and female cases was reduced 64.22% [(32 524 − 11 638)/32 524 × 100%] and 39.22% [(21 502 − 13 069)/21 502 × 100%], respectively. If we set β _f = 0, the 5-year-average value of total cases was reduced 27.31% [(54 026 − 39 269)/54 026 × 100%] and male and female cases was reduced 20.87% [(32 524 − 25 735)/32 524 × 100%] and 37.06% [(21 502 − 13 534)/21 502 × 100%], respectively. If we set β _fm = 0, the 5-year-average value of total cases was reduced 34.69% [(54 026 − 35 284)/54 026 × 100%] and male and female cases was reduced 39.24% [(32 524 − 19 760)/32 524 × 100%] and 27.80% [(21 502 − 15 524)/21 502 × 100%], respectively. If we set β _mf = 0, the 5-year-average value of total cases was reduced 42.94% [(54 026 − 30 830)/54 026 × 100%] and male and female cases was reduced 29.85% [(32 524 − 22 816)/32 524 × 100%] and 62.73% [(21 502 − 8014)/21 502 × 100%], respectively. Similar results were observed in 2013 and 2017, except in 2014–2016 (Fig. 5 and Table 3).

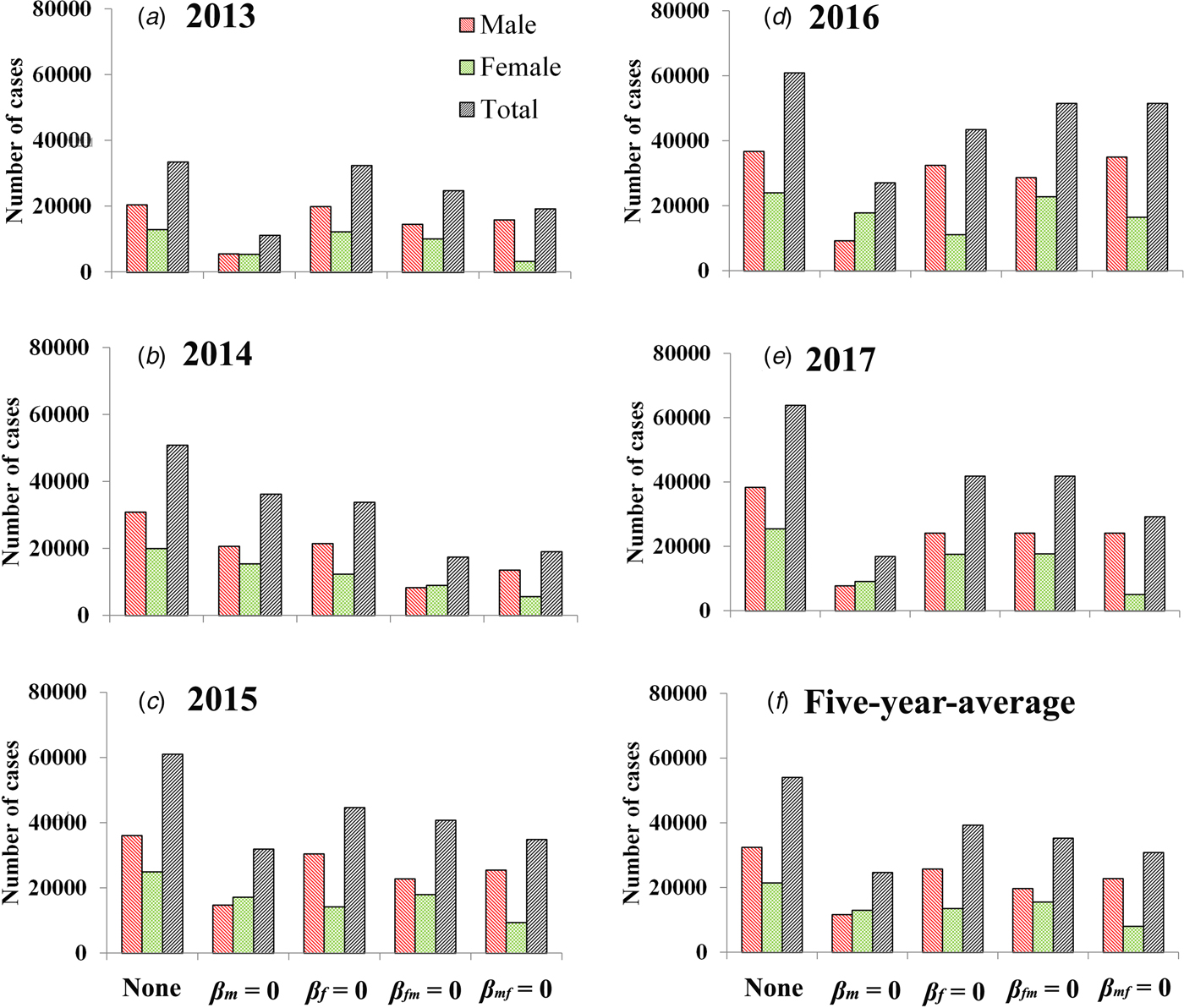
Fig. 5. Reduction of cases under the different conditions (none, β _m = 0, β _f = 0, β _fm = 0 and β _mf = 0). (A–E) Scenarios in 2013 to 2017; (F) results of 5-year-average value. None refers to no intervention implemented.

Table 3. PR (%) in the four scenarios (β _m = 0, β _f = 0, β _fm = 0 and β _mf = 0) from 2013 to 2017 in Shenzhen City

When we focus on the 5-year-average male cases, RTI of M vs. F, F vs. F, FM vs. F and MF vs. F was 3.08, 1.00, 1.88 and 1.43, respectively. When we focus on the 5-year-average female cases, RTI of M vs. F, F vs. F, FM vs. F and MF vs. F was 1.06, 1.00, 0.75 and 1.69, respectively. When we focus on the 5-year-average total cases, RTI of M vs. F, F vs. F, FM vs. F and MF vs. F was 1.99, 1.00, 1.27 and 1.57, respectively. Similar results were observed in 2013 and 2017, except in 2014–2016 (Table 4).

Table 4. RTI in the four scenarios (M vs. F, F vs. F, FM vs. F and MF vs. F) from 2013 to 2017 in Shenzhen City

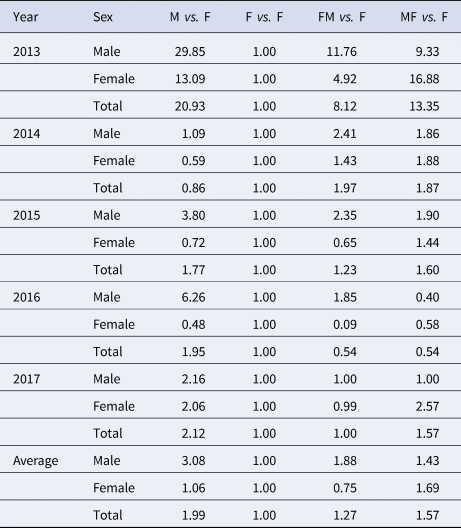
M, male; F, female; FM, from female to male; MF, from male to female.

Discussion

Significant difference of HFMD incidence between male and female is commonly observed by the descriptive epidemiology method [20–23]. We assumed that this phenomenon is attributed to the different transmissibility among male and female individuals. In this study, the HFMD incidence of male was slightly higher than that of female in Shenzhen City, although the difference value was lower than the published data [15]. To verify our hypothesis, we developed an intersex transmission SIR model to explore the difference first. Our simulation results showed that the value of the transmission relative rate among male, among female, from male to female and from female to male was different, and they have the following order: β _m > β _mf > β _fm > β _f. Therefore, the values of R _β have the following order: M vs. F > MF vs. F > FM vs. F > F vs. F.

Considering that β is a process parameter, it plays the role of transmission force behind the phenomenon. To make the outcomes more direct, we simulated several ‘knockout’ scenarios (β _m = 0, β _mf = 0, β _fm = 0 and β _f = 0) orderly. The results of the simulation showed that the values of RTI have the following order: M vs. F > MF vs. F > FM vs. F > F vs. F. This order is the same as that of R _β. These findings revealed that male individuals are more transmissible than female individuals. Therefore, the different transmissibility between male and female is the reason of the significance of gender distribution.

Published research showed that most HFMD cases have an age lower than 5 years especially lower than 3 years [15, 18, 19, 24]. A system review showed that being male is a risk factor for both mild and severe HFMD [25]. Their findings suggest that boys are more likely to develop symptoms, more involved in propagation of outbreaks or more likely to be brought for medical care than girls [25]. Our results show that the values of β among male and from male to female were higher than those among female and from female to male. To our knowledge, boy is more active than girl. The daily contact rate among boys, from boy to girl and between boy and environment is higher than that of girl. These differences might lead to the higher values of β among male and from male to female. However, the value of β might be affected by multifactor including behaviour of individuals and environment. More research might be needed to explore the multifactorial interaction.

Of note, there is a limitation that the skewed distribution of age was not considered in our study. The relative transmissibility might be different at different age groups. However, to explore the age-specific relative transmissibility, more complex model and age distribution data are needed in the future.

Conclusion

The HFMD incidence of male is higher than that of female. The transmissibility of HFMD is different between male and female individuals. Male cases seem to be more transmissible than female.