Published online by Cambridge University Press: 05 January 2023
Finite element methods developed for unfitted meshes have been widely applied to various interface problems. However, many of them resort to non-conforming spaces for approximation, which is a critical obstacle for the extension to 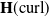 $\textbf{H}(\text{curl})$ equations. This essential issue stems from the underlying Sobolev space
$\textbf{H}(\text{curl})$ equations. This essential issue stems from the underlying Sobolev space  $\textbf{H}^s(\text{curl};\,\Omega)$, and even the widely used penalty methodology may not yield the optimal convergence rate. One promising approach to circumvent this issue is to use a conforming test function space, which motivates us to develop a Petrov–Galerkin immersed finite element (PG-IFE) method for
$\textbf{H}^s(\text{curl};\,\Omega)$, and even the widely used penalty methodology may not yield the optimal convergence rate. One promising approach to circumvent this issue is to use a conforming test function space, which motivates us to develop a Petrov–Galerkin immersed finite element (PG-IFE) method for 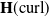 $\textbf{H}(\text{curl})$-elliptic interface problems. We establish the Nédélec-type IFE spaces and develop some important properties including their edge degrees of freedom, an exact sequence relating to the
$\textbf{H}(\text{curl})$-elliptic interface problems. We establish the Nédélec-type IFE spaces and develop some important properties including their edge degrees of freedom, an exact sequence relating to the  $H^1$ IFE space and optimal approximation capabilities. We analyse the inf-sup condition under certain assumptions and show the optimal convergence rate, which is also validated by numerical experiments.
$H^1$ IFE space and optimal approximation capabilities. We analyse the inf-sup condition under certain assumptions and show the optimal convergence rate, which is also validated by numerical experiments.