Let $\mathbf {D}$ be a bounded homogeneous domain in ${\mathbb {C}}^n$. In this note, we give a characterization of the Stein domains in $\mathbf {D}$ which are invariant under a maximal unipotent subgroup N of $Aut(\mathbf {D})$. We also exhibit an N-invariant potential of the Bergman metric of $\mathbf {D}$, expressed in a Lie theoretical fashion. These results extend the ones previously obtained by the authors in the symmetric case.

A Vaisman manifold is a special kind of locally conformally Kähler manifold, which is closely related to a Sasaki manifold. In this paper, we show a basic structure theorem of simply connected homogeneous Sasaki and Vaisman manifolds of unimodular Lie groups, up to holomorphic isometry. For the case of unimodular Lie groups, we obtain a complete classification of simply connected Sasaki and Vaisman unimodular Lie groups, up to modification.

Suppose $G$ is a connected complex Lie group and $H$ is a closed complex subgroup. Then there exists a closed complex subgroup $J$ of $G$ containing $H$ such that the fibration $\pi :G/H\to $$G/J$ is the holomorphic reduction of $G/H$i.e., $G/J$ is holomorphically separable and $\mathcal{O}(G/H)\cong $${{\pi }^{*}}\mathcal{O}(G/J)$. In this paper we prove that if $G/H$ is pseudoconvex, i.e., if $G/H$ admits a continuous plurisubharmonic exhaustion function, then $G/J$ is Stein and $J/H$ has no non-constant holomorphic functions.

We collect evidence in support of a conjecture of Griffiths, Green, and Kerr on the arithmetic of extension classes of limiting mixed Hodge structures arising from semistable degenerations over a number field. After briefly summarizing how a result of Iritani implies this conjecture for a collection of hypergeometric Calabi–Yau threefold examples studied by Doran and Morgan, the authors investigate a sequence of (non-hypergeometric) examples in dimensions $1\,\le \,d\,\le \,6$ arising from Katz's theory of the middle convolution. A crucial role is played by the Mumford-Tate group (which is ${{G}_{2}}$ ) of the family of 6-folds, and the theory of boundary components of Mumford–Tate domains.

If $X$ is a connected complex manifold with ${{d}_{X}}\,=\,2$ that admits a (connected) Lie group $G$ acting transitively as a group of holomorphic transformations, then the action extends to an action of the complexification $\widehat{G}$ of $G$ on $X$ except when either the unit disk in the complex plane or a strictly pseudoconcave homogeneous complex manifold is the base or fiber of some homogeneous fibration of $X$.

We show that the modulus of the Bergman kernel $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}B(z, \zeta )$ of a general homogeneous Siegel domain of type II is ‘almost constant’ uniformly with respect to $z$ when $\zeta $ varies inside a Bergman ball. The control is expressed in terms of the Bergman distance. This result was proved by A. Korányi for symmetric Siegel domains of type II. Subsequently, R. R. Coifman and R. Rochberg used it to establish an atomic decomposition theorem and an interpolation theorem by functions in Bergman spaces $A^p$ on these domains. The atomic decomposition theorem and the interpolation theorem are extended here to the general homogeneous case using the same tools. We further extend the range of exponents $p$ via functional analysis using recent estimates.

We show that the Bergman metric of a bounded homogeneous domain has a potential function whose gradient has a constant norm with respect to the Bergman metric, and further that this constant is independent of the choice of such a potential function.

we study some automorphic cohomology classes of degree one on the griffiths–schmid varieties attached to some unitary groups in three variables. using partial compactifications of those varieties, constructed by kato and usui, we define for such a cohomology class some analogues of fourier–shimura coefficients, which are cohomology classes on certain elliptic curves. we show that a large space of such automorphic classes can be generated by those with rational ‘coefficients’. more precisely, we consider those cohomology classes that come from picard modular forms, via some penrose-like transform studied in a previous article: we prove that the coefficients of the classes thus obtained can be computed from the coefficients of the picard form by a similar transform defined at the level of the elliptic curve.

Let Γ be a discrete cocompact subgroup of SL2(ℂ). We conjecture that the quotient manifold X = SL2(ℂ) / Γ contains infinitely many non-isogenous elliptic curves and prove this is indeed the case if Schanuel’s conjecture holds. We also prove it in the special case where Γ ∩ SL2(∝) is cocompact in SL2(ℝ).

Furthermore, we deduce some consequences for the geodesic length spectra of real hyperbolic 2- and 3-folds.

Define dx to be the codimension of the top nonvanishing homology group of the manifold X with coefficients in 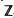2. We investigate homogeneous spaces X := G/H, where G is a connected complex Lie group and H is a closed complex subgroup for which dx = 1,2 and O(X) ≠ ℂ. There exists a fibration π: G/H → G/U such that G/U is holomorphically separable and π*(O(G/U)) = O(G/H), see [11]. We prove the following. If dx = 1, then F := U/H is compact and connected and Y :=G/U is an affine cone with its vertex removed. If dx = 2, then either F is connected with dF = 1 and Y is an affine cone with its vertex removed, or F is compact and connected and dy = 2, where Y is ℂ, the affine quadric Q2, ℙ2 — Q (with Q a quadric curve) or a homogeneous holomorphic 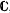* -bundle over an affine cone minus its vertex which is itself an algebraic principal bundle or which admits a two-to-one covering that is.

Given any homogeneous complex manifold X = G/H, there exists a natural coset map π :G/H → G/K satisfying π (X1) = π (x2) if and only if dx(x1 x2) = 0, where dx denotes the Kobayashi pseudometric on X. Its typical fiber Z : = K/H is a connected complex submanifold of X. Also G/K has a (7-invariant complex structure, provided K satisfies a certain technical assumption (see Theorem 3). If Z is compact as well, then G/K is biholomorphic to a homogeneous bounded domain.

A complex nilmanifold X is isomorphic to a product X ⋍ ℂp x N/┌, where N is a simply connected nilpotent complex Lie group and ┌ is a discrete subgroup of N not contained in a proper connected complex subgroup of N. The pair (N, ┌) is uniquely determined up to holomorphic group isomorphisms.