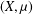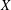We examine a class of ergodic transformations on a probability measure space $(X,{\it\mu})$ and show that they extend to representations of ${\mathcal{B}}(L^{2}(X,{\it\mu}))$ that are both implemented by a Cuntz family and ergodic. This class contains several known examples, which are unified in our work. During the analysis of the existence and uniqueness of this Cuntz family, we find several results of independent interest. Most notably, we prove a decomposition of $X$ for $N$-to-one local homeomorphisms that is connected to the orthonormal bases of certain Hilbert modules.

We determine the general solutions of the functional equation for ƒi: G → F (i = 1,2,3,4), where G is a 2-divisible group and F is a commutative field of characteristic different from 2. The motivation for studying this equation came from a result due to Dry gas [4] where he proved a Jordan and von Neumann type characterization theorem for quasi-inner products. Also, this equation is a generalization of the quadratic functional equation investigated by several authors in connection with inner product spaces and their generalizations. Special cases of this equation include the Cauchy equation, the Jensen equation, the Pexider equation and many more. Here, we determine the general solution of this equation without any regularity assumptions on ƒi.

Together with Vern Paulsen we were able to show that the elementary theory of tensor norms of Banach spaces carries over to operator spaces. We suggested that the Grothendieck tensor norm program, which was of course enormously important in the development of Banach space theory, be carried out for operator spaces. Some of this has been done by the authors mentioned above, and by Effros and Ruan. We give alternative developments of some of this work, and otherwise continue the tensor norm program.