In this study, we investigate the intial value problem (IVP) for a time-fractional fourth-order equation with nonlinear source terms. More specifically, we consider the time-fractional biharmonic with exponential nonlinearity and the time-fractional Cahn–Hilliard equation. By using the Fourier transform concept, the generalized formula for the mild solution as well as the smoothing effects of resolvent operators are proved. For the IVP associated with the first one, by using the Orlicz space with the function 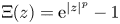$\Xi (z)={\textrm {e}}^{|z|^{p}}-1$ and some embeddings between it and the usual Lebesgue spaces, we prove that the solution is a global-in-time solution or it shall blow up in a finite time if the initial value is regular. In the case of singular initial data, the local-in-time/global-in-time existence and uniqueness are derived. Also, the regularity of the mild solution is investigated. For the IVP associated with the second one, some modifications to the generalized formula are made to deal with the nonlinear term. We also establish some important estimates for the derivatives of resolvent operators, they are the basis for using the Picard sequence to prove the local-in-time existence of the solution.

We consider a diffuse interface model of tumour growth proposed by A. Hawkins-Daruud et al. ((2013) J. Math. Biol.67 1457–1485). This model consists of the Cahn–Hilliard equation for the tumour cell fraction ϕ nonlinearly coupled with a reaction–diffusion equation for ψ, which represents the nutrient-rich extracellular water volume fraction. The coupling is expressed through a suitable proliferation function p(ϕ) multiplied by the differences of the chemical potentials for ϕ and ψ. The system is equipped with no-flux boundary conditions which give the conservation of the total mass, that is, the spatial average of ϕ + ψ. Here, we prove the existence of a weak solution to the associated Cauchy problem, provided that the potential F and p satisfy sufficiently general conditions. Then we show that the weak solution is unique and continuously depends on the initial data, provided that p satisfies slightly stronger growth restrictions. Also, we demonstrate the existence of a strong solution and that any weak solution regularizes in finite time. Finally, we prove the existence of the global attractor in a phase space characterized by an a priori bounded energy.