We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We show the existence of a measurable selector in Carpenter’s Theorem due to Kadison. This solves a problem posed by Jasper and the first author in an earlier work. As an application we obtain a characterization of all possible spectral functions of shift-invariant subspaces of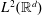 
$L^{2}(\mathbb{R}^{d})$ and Carpenter’s Theorem for type 
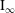$\text{I}_{\infty }$ von Neumann algebras.
