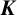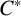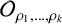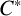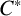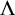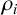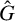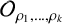We introduce certain 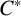 $C^*$
-algebras and k-graphs associated to k finite-dimensional unitary representations
$C^*$
-algebras and k-graphs associated to k finite-dimensional unitary representations  $\rho _1,\ldots ,\rho _k$
of a compact group G. We define a higher rank Doplicher-Roberts algebra
$\rho _1,\ldots ,\rho _k$
of a compact group G. We define a higher rank Doplicher-Roberts algebra 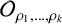 $\mathcal {O}_{\rho _1,\ldots ,\rho _k}$
, constructed from intertwiners of tensor powers of these representations. Under certain conditions, we show that this
$\mathcal {O}_{\rho _1,\ldots ,\rho _k}$
, constructed from intertwiners of tensor powers of these representations. Under certain conditions, we show that this 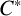 $C^*$
-algebra is isomorphic to a corner in the
$C^*$
-algebra is isomorphic to a corner in the 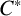 $C^*$
-algebra of a row-finite rank k graph
$C^*$
-algebra of a row-finite rank k graph 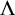 $\Lambda $
with no sources. For G finite and
$\Lambda $
with no sources. For G finite and 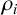 $\rho _i$
faithful of dimension at least two, this graph is irreducible, it has vertices
$\rho _i$
faithful of dimension at least two, this graph is irreducible, it has vertices 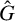 $\hat {G}$
and the edges are determined by k commuting matrices obtained from the character table of the group. We illustrate this with some examples when
$\hat {G}$
and the edges are determined by k commuting matrices obtained from the character table of the group. We illustrate this with some examples when 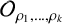 $\mathcal {O}_{\rho _1,\ldots ,\rho _k}$
is simple and purely infinite, and with some K-theory computations.
$\mathcal {O}_{\rho _1,\ldots ,\rho _k}$
is simple and purely infinite, and with some K-theory computations.
 $\boldsymbol {C}^{*}$
-ALGEBRAS FROM
$\boldsymbol {C}^{*}$
-ALGEBRAS FROM 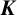 $\boldsymbol {K}$
GROUP REPRESENTATIONS
$\boldsymbol {K}$
GROUP REPRESENTATIONS