For a general autonomous planar polynomial differential system, it is difficult to find conditions that are easy to verify and which guarantee global asymptotic stability, weakening the Markus–Yamabe condition. In this paper, we provide three conditions that guarantee the global asymptotic stability for polynomial differential systems of the form 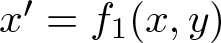 $x^{\prime}=f_1(x,y)$,
$x^{\prime}=f_1(x,y)$, 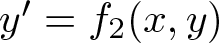 $y^{\prime}=f_2(x,y)$, where f1 has degree one, f2 has degree
$y^{\prime}=f_2(x,y)$, where f1 has degree one, f2 has degree  $n\ge 1$ and has degree one in the variable y. As a consequence, we provide sufficient conditions, weaker than the Markus–Yamabe conditions that guarantee the global asymptotic stability for any generalized Liénard polynomial differential system of the form
$n\ge 1$ and has degree one in the variable y. As a consequence, we provide sufficient conditions, weaker than the Markus–Yamabe conditions that guarantee the global asymptotic stability for any generalized Liénard polynomial differential system of the form 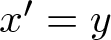 $x^{\prime}=y$,
$x^{\prime}=y$, 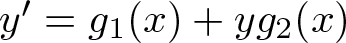 $y^{\prime}=g_1(x) +y g_2(x)$ with g1 and g2 polynomials of degrees n and m, respectively.
$y^{\prime}=g_1(x) +y g_2(x)$ with g1 and g2 polynomials of degrees n and m, respectively.
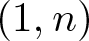 $(1,n)$
$(1,n)$