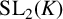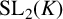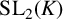2 results
BASIC NONARCHIMEDEAN JØRGENSEN THEORY
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 19 March 2024, pp. 1-15
-
- Article
-
- You have access
- HTML
- Export citation
-
We prove a nonarchimedean analogue of Jørgensen’s inequality, and use it to deduce several algebraic convergence results. As an application, we show that every dense subgroup of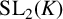
${\mathrm {SL}_2}(K)$, where K is a p-adic field, contains two elements that generate a dense subgroup of 
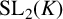${\mathrm {SL}_2}(K)$, which is a special case of a result by Breuillard and Gelander [‘On dense free subgroups of Lie groups’, J. Algebra 261(2) (2003), 448–467]. We also list several other related results, which are well known to experts, but not easy to locate in the literature; for example, we show that a nonelementary subgroup of 
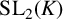${\mathrm {SL}_2}(K)$ over a nonarchimedean local field K is discrete if and only if each of its two-generator subgroups is discrete.
JØRGENSEN’S INEQUALITY FOR QUATERNIONIC HYPERBOLIC SPACE WITH ELLIPTIC ELEMENTS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 81 / Issue 1 / February 2010
- Published online by Cambridge University Press:
- 02 October 2009, pp. 121-131
- Print publication:
- February 2010
-
- Article
-
- You have access
- Export citation
