We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We consider the Cauchy problem
where N > 2, p > 1, and u0 is a bounded continuous non-negative function in RN. We study the case where u0(x) decays at the rate |x|−2/(p−1) as |x| → ∞, and investigate the convergence property of the global solutions to the forward self-similar solutions. We first give the precise description of the relationship between the spatial decay of initial data and the large time behaviour of solutions, and then we show the existence of solutions with a time decay rate slower than the one of self-similar solutions. We also show the existence of solutions that behave in a complicated manner.
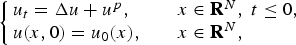$$\left\{ {\matrix{ {u_t = \Delta u + u^p,\quad } \hfill & {x\in {\bf R}^N,\;t \leq 0,} \hfill \cr {u(x,0) = u_0(x),\quad } \hfill & {x\in {\bf R}^N,} \hfill \cr } } \right.$$
