1 results
Random triangular groups at density
$1/3$
- Part of
-
- Journal:
- Compositio Mathematica / Volume 151 / Issue 1 / January 2015
- Published online by Cambridge University Press:
- 27 November 2014, pp. 167-178
- Print publication:
- January 2015
-
- Article
-
- You have access
- Export citation
-
Let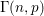
${\rm\Gamma}(n,p)$ denote the binomial model of a random triangular group. We show that there exist constants 
$c,C>0$ such that if 
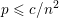$p\leqslant c/n^{2}$, then asymptotically almost surely (a.a.s.) 
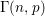${\rm\Gamma}(n,p)$ is free, and if 
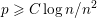$p\geqslant C\log n/n^{2}$, then a.a.s. 
${\rm\Gamma}(n,p)$ has Kazhdan’s property (T). Furthermore, we show that there exist constants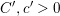 
$C^{\prime },c^{\prime }>0$ such that if 
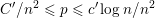$C^{\prime }/n^{2}\leqslant p\leqslant c^{\prime }\log n/n^{2}$, then a.a.s. 
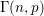${\rm\Gamma}(n,p)$ is neither free nor has Kazhdan’s property (T).
