Search

We study the asymptotic behaviour of the following nonlinear problem: $$\{\begin{array}{ll}-{\rm div}(a( Du_h))+\vert u_h\vert^{p-2}u_h =f \quad\hbox{in }\Omega_h, a( Du_h)\cdot\nu = 0 \quad\hbox{on }\partial\Omega_h, \end{array}.$$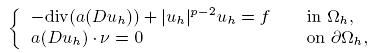

in a domain Ωh of $\mathbb{R}^n$ 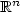whose boundary ∂Ωh contains an oscillating part with respect to hwhen h tends to ∞. The oscillating boundary is defined by a set of cylinders with axis 0xn that are h -1-periodically distributed. We prove that the limit problem in the domain corresponding tothe oscillating boundary identifieswith a diffusion operator with respect tox n coupled with an algebraic problemfor the limit fluxes.

The computation of glacier movements leads to a system of nonlinear partial differentialequations. The existence and uniqueness of a weak solution is established by using the calculus ofvariations. A discretization by the finite element method is done. Thesolution of the discrete problem is proved to be convergent to the exactsolution. A first simple numerical algorithm is proposed and its convergence numericallystudied.

Search Results

Refine search

Refine search

Actions for selected content:

2 results

Homogenization of a monotone problemin a domain with oscillating boundary

A strongly nonlinear problem arising in glaciology

Search Results

Refine search

Refine search

Actions for selected content:

Save Search

2 results

Homogenization of a monotone problemin a domain with oscillating boundary

A strongly nonlinear problem arising in glaciology