We formulate combinatorial principles that combine the square principle with various strong forms of the diamond principle, and prove that the strongest amongst them holds in L for every infinite cardinal.

As an application, we prove that the following two hold in L:

1. 1. For every infinite regular cardinal λ, there exists a special λ+-Aronszajn tree whose projection is almost Souslin;

2. 2. For every infinite cardinal λ, there exists a respecting λ+-Kurepa tree; Roughly speaking, this means that this λ+-Kurepa tree looks very much like the λ+-Souslin trees that Jensen constructed in L.

If M is a proper class inner model of ZFC and 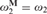$\omega _2^{\bf{M}} = \omega _2 $, then every sound mouse projecting to ω and not past 0¶ belongs to M. In fact, under the assumption that 0¶ does not belong to M, 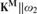${\bf{K}}^{\bf{M}} \parallel \omega _2 $ is universal for all countable mice in V.

Similarly, if M is a proper class inner model of ZFC, δ > ω1 is regular, (δ+)M = δ+ and in V there is no proper class inner model with a Woodin cardinal, then 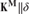${\bf{K}}^{\bf{M}} \parallel \delta $ is universal for all mice in V of cardinality less than δ.