In the study of the spectra of algebras of holomorphic functions on a Banach space E, the bidual E″ has a central role, and the spectrum is often shown to be locally homeomorphic to E″. In this paper we consider the problem of spectra of subalgebras invariant under the action of a group (functions f such that f ○ g = f). It is natural to attempt a characterization in terms of the space of orbits E″/~ obtained from E″ through the action of the group, so we pursue this approach here and introduce an analytic structure on the spectrum in some situations. In other situations we encounter some obstacles: in some cases, the lack of structure of E″/~ itself; in others, problems of weak continuity and non-approximability of functions in the algebra. We also define a convolution operation related to the spectrum.

It is known that all k-homogeneous orthogonally additive polynomials P over C(K) are of the form

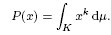
Thus, x ↦ xk factors all orthogonally additive polynomials through some linear form μ. We show that no such linearization is possible without homogeneity. However, we also show that every orthogonally additive holomorphic function of bounded type f over C(K) is of the form

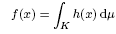
for some μ and holomorphic h : C (K) → L1(μ) of bounded type.

We give a characterization of composition operators between algebras of analytic functions on a Banach space. We show (under fairly general conditions) that they are precisely the multiplicative operators that are transposes of operators of between the preduals of the algebras. The special cases of $H^\infty(U)$ and $H_{\mathrm{b}}(U)$ are considered. In these cases, the composition operators are those which are pointwise-to-pointwise continuous and/or $\tau_0$-to-$\tau_0$ continuous (where $\tau_0$ is the compact-open topology). We obtain Banach–Stone-type theorems for these algebras.