The paper considers a modified storage process 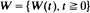 with state space [0,∞). Away from the origin, W behaves like an ordinary storage process with constant release rate and finite jump intensity A. In state 0, however, the jump intensity falls to
with state space [0,∞). Away from the origin, W behaves like an ordinary storage process with constant release rate and finite jump intensity A. In state 0, however, the jump intensity falls to  It is shown that W can be obtained by applying first a reflection mapping and then a random change of time scale to a compound Poisson process with drift. When these same two transformations are applied to Brownian motion, one obtains sticky (or slowly reflected) Brownian motion W∗ on [0,∞). Thus W∗ is the natural diffusion approximation for W, and it is shown that W converges in distribution to W∗ under appropriate conditions. The boundary behavior of W∗ is discussed, its infinitesimal generator is calculated and its stationary distribution (which has an atom at the origin) is computed.
It is shown that W can be obtained by applying first a reflection mapping and then a random change of time scale to a compound Poisson process with drift. When these same two transformations are applied to Brownian motion, one obtains sticky (or slowly reflected) Brownian motion W∗ on [0,∞). Thus W∗ is the natural diffusion approximation for W, and it is shown that W converges in distribution to W∗ under appropriate conditions. The boundary behavior of W∗ is discussed, its infinitesimal generator is calculated and its stationary distribution (which has an atom at the origin) is computed.

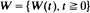 with state space [0,∞). Away from the origin,
with state space [0,∞). Away from the origin,  It is shown that
It is shown that 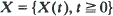 be a process with stationary, independent increments and no negative jumps. Let
be a process with stationary, independent increments and no negative jumps. Let 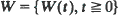 be this same process modified by a reflecting barrier at zero (a storage process). Assuming that –
be this same process modified by a reflecting barrier at zero (a storage process). Assuming that –  and denote by ψ(
and denote by ψ(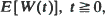 as a function of
as a function of 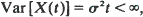 it follows that
it follows that 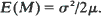 These surprisingly simple formulas were originally obtained by Zolotarev using analytical methods.
These surprisingly simple formulas were originally obtained by Zolotarev using analytical methods.