We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We completely characterize the validity of the inequality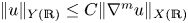 
$\| u \|_{Y(\mathbb R)} \leq C \| \nabla^{m} u \|_{X(\mathbb R)}$, where X and Y are rearrangement-invariant spaces, by reducing it to a considerably simpler one-dimensional inequality. Furthermore, we fully describe the optimal rearrangement-invariant space on either side of the inequality when the space on the other side is fixed. We also solve the same problem within the environment in which the competing spaces are Orlicz spaces. A variety of examples involving customary function spaces suitable for applications is also provided.
