We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We prove existence results of two solutions of the problem
where 
\[ \begin{cases} L(u)+u^{m-1}=\lambda u^{p-1} & \text{in}\ \Omega,\\ u>0 & \text{in}\ \Omega,\\ u=0 & \text{on}\ \partial \Omega, \end{cases} \]
$L(v)=-\textrm {div}(M(x)\nabla v)$ is a linear operator, 
$p\in (2,2^{*}]$ and 
$\lambda$ and 
$m$ sufficiently large. Then their asymptotical limit as 
$m\to +\infty$ is investigated showing different behaviours.
This paper deals with solutions of semilinear elliptic equations of the type
where Ω is a radially symmetric domain of the plane that can be bounded or unbounded. We consider solutions u that are invariant by rotations of a certain angle θ and which have a bound on their Morse index in spaces of functions invariant by these rotations. We can prove that or u is radial, or, else, there exists a direction 
\[ \left\{\begin{array}{@{}ll} -\Delta u = f(|x|, u) \qquad & \text{ in } \Omega, \\ u= 0 & \text{ on } \partial \Omega, \end{array} \right. \]
$e\in \mathcal {S}$ such that u is symmetric with respect to e and it is strictly monotone in the angular variable in a sector of angle θ/2. The result applies to least-energy and nodal least-energy solutions in spaces of functions invariant by rotations and produces multiplicity results.
In this paper we study the problem
where Ω = ℝ
N
or Ω = B
1, N ⩾ 3, p > 1 and 
. Using a suitable map we transform problem (1) into another one without the singularity 1/|x|2. Then we obtain some bifurcation results from the radial solutions corresponding to some explicit values of λ.
In this paper we consider the following semilinear elliptic equation
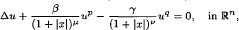
where n ≥ 3,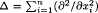 
and β ≥ 0, γ ≥ 0, q > p ≥ 1, μ and ν are real constants. We note that if γ = 0, β > 0 and ν ≥ 2, then the equation above is called the Matukuma-type equation. If β = 0, γ > 0 and ν > 2, then the complete classification of all possible positive solutions had been conducted by Cheng and Ni. If β > 0, γ > 0 and μ ≥ ν ≥ 2, then some results about the maximal solution and positive solution structures can be found in Chern. The purpose of this paper is to discuss and investigate the blow-up and positive entire solutions of the equation above for the μ ≥ 2 ≥ ν case.
We consider a control constrained optimal control problem governed by a semilinear elliptic equation with nonlocal interface conditions. These conditions occur during themodeling of diffuse-gray conductive-radiative heat transfer. After stating first-order necessary conditions, second-order sufficient conditions are derived that account for strongly active sets. These conditions ensure local optimality in an Ls -neighborhood of a reference function whereby the underlying analysis allows to use weaker norms than $L^\infty$ 
.
Let f be an odd function of a class C2 such that ƒ(1) = 0,ƒ'(0) < 0,ƒ'(1) > 0 and $x\mapsto f(x)/x$ 
increases on [0,1]. We approximate the positive solution of Δu + ƒ(u) = 0, on $\xR_{+}^{2}$ 
with homogeneous Dirichlet boundary conditions by thesolution of $-\Delta u_{L}+f(u_{L})=0,$ 
on ]0,L[2 with adequatenon-homogeneous Dirichlet conditions. We show that the error uL - utends to zero exponentially fast, in the uniform norm.
