1 results
An n-dimensional chemotaxis system with signal-dependent motility and generalized logistic source: global existence and asymptotic stabilization
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 151 / Issue 2 / April 2021
- Published online by Cambridge University Press:
- 29 May 2020, pp. 821-841
- Print publication:
- April 2021
-
- Article
- Export citation
-
This paper deals with the global existence for a class of Keller–Segel model with signal-dependent motility and general logistic term under homogeneous Neumann boundary conditions in a higher-dimensional smoothly bounded domain, which can be written as
It is shown that whenever ρ ∈ ℝ, μ > 0 and
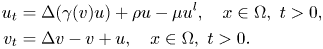$$\eqalign{& u_t = \Delta (\gamma (v)u) + \rho u-\mu u^l,\quad x\in \Omega ,\;t > 0, \cr & v_t = \Delta v-v + u,\quad x\in \Omega ,\;t > 0.} $$then the considered system possesses a global classical solution for all sufficiently smooth initial data. Furthermore, the solution converges to the equilibrium
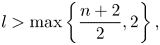$$l > \max \left\{ {\displaystyle{{n + 2} \over 2},2} \right\},$$as t → ∞ under some extra hypotheses, where ρ+ = max{ρ, 0}.
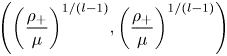$$\left( {{\left( {\displaystyle{{\rho _ + } \over \mu }} \right)}^{1/(l-1)},{\left( {\displaystyle{{\rho _ + } \over \mu }} \right)}^{1/(l-1)}} \right)$$
