1 results
SOME GLOBAL EXISTENCE RESULTS ON LOCALLY FINITE GRAPHS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 06 November 2023, pp. 1-17
-
- Article
-
- You have access
- HTML
- Export citation
-
Let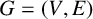
$G=(V, E)$ be a locally finite graph with the vertex set V and the edge set E, where both V and E are infinite sets. By dividing the graph G into a sequence of finite subgraphs, the existence of a sequence of local solutions to several equations involving the p-Laplacian and the poly-Laplacian systems is confirmed on each subgraph, and the global existence for each equation on graph G is derived by the convergence of these local solutions. Such results extend the recent work of Grigor’yan, Lin and Yang [J. Differential Equations, 261 (2016), 4924–4943; Rev. Mat. Complut., 35 (2022), 791–813]. The method in this paper also provides an idea for investigating similar problems on infinite graphs.
