1 results
Stable finiteness of twisted group rings and noisy linear cellular automata
- Part of
-
- Journal:
- Canadian Journal of Mathematics / Volume 76 / Issue 4 / August 2024
- Published online by Cambridge University Press:
- 22 May 2023, pp. 1089-1108
- Print publication:
- August 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
-
For linear nonuniform cellular automata (NUCA) which are local perturbations of linear CA over a group universe G and a finite-dimensional vector space alphabet V over an arbitrary field k, we investigate their Dedekind finiteness property, also known as the direct finiteness property, i.e., left or right invertibility implies invertibility. We say that the group G is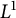
$L^1$-surjunctive, resp. finitely 
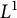$L^1$-surjunctive, if all such linear NUCA are automatically surjective whenever they are stably injective, resp. when in addition k is finite. In parallel, we introduce the ring 
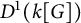$D^1(k[G])$ which is the Cartesian product 
$k[G] \times (k[G])[G]$ as an additive group but the multiplication is twisted in the second component. The ring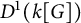 
$D^1(k[G])$ contains naturally the group ring 
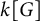$k[G]$ and we obtain a dynamical characterization of its stable finiteness for every field k in terms of the finite 
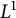$L^1$-surjunctivity of the group G, which holds, for example, when G is residually finite or initially subamenable. Our results extend known results in the case of CA.
