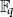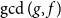1 results
Average-case complexity of the Euclidean algorithm with a fixed polynomial over a finite field
- Part of
-
- Journal:
- Combinatorics, Probability and Computing / Volume 31 / Issue 1 / January 2022
- Published online by Cambridge University Press:
- 06 July 2021, pp. 166-183
-
- Article
- Export citation
-
We analyse the behaviour of the Euclidean algorithm applied to pairs (g,f) of univariate nonconstant polynomials over a finite field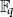
$\mathbb{F}_{q}$
of q elements when the highest degree polynomial g is fixed. Considering all the elements f of fixed degree, we establish asymptotically optimal bounds in terms of q for the number of elements f that are relatively prime with g and for the average degree of 
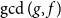$\gcd(g,f)$
. We also exhibit asymptotically optimal bounds for the average-case complexity of the Euclidean algorithm applied to pairs (g,f) as above.
