We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
In this work we study the Cauchy problem in Gevrey spaces for a generalized class of equations that contains the case $b=0$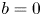
of the $b$
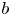-equation. For the generalized equation, we prove that it is locally well-posed for initial data in Gevrey spaces. Moreover, as we move to global well-posedness, we show that for a particular choice of the parameter in the equation the local solution is global analytic in both time and spatial variables.
