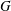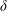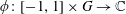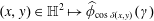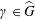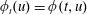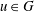1 results
Positive Definiteness on Products of Compact Two-point Homogeneous Spaces and Locally Compact Abelian Groups
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 63 / Issue 4 / December 2020
- Published online by Cambridge University Press:
- 29 October 2019, pp. 705-715
- Print publication:
- December 2020
-
- Article
- Export citation
-
In this paper, we consider the problem of characterizing positive definite functions on compact two-point homogeneous spaces cross locally compact abelian groups. For a locally compact abelian group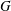
$G$ with dual group 
$\widehat{G}$, a compact two-point homogeneous space 
$\mathbb{H}$ with normalized geodesic distance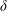 
$\unicode[STIX]{x1D6FF}$ and a profile function 
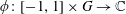$\unicode[STIX]{x1D719}:[-1,1]\times G\rightarrow \mathbb{C}$ satisfying certain continuity and integrability assumptions, we show that the positive definiteness of the kernel 
$((x,u),(y,v))\in (\mathbb{H}\times G)^{2}\mapsto \unicode[STIX]{x1D719}(\cos \unicode[STIX]{x1D6FF}(x,y),uv^{-1})$ is equivalent to the positive definiteness of the Fourier transformed kernels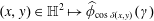 
$(x,y)\in \mathbb{H}^{2}\mapsto \widehat{\unicode[STIX]{x1D719}}_{\cos \unicode[STIX]{x1D6FF}(x,y)}(\unicode[STIX]{x1D6FE})$, 
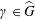$\unicode[STIX]{x1D6FE}\in \widehat{G}$, where 
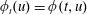$\unicode[STIX]{x1D719}_{t}(u)=\unicode[STIX]{x1D719}(t,u)$, 
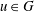$u\in G$. We also provide some results on the strict positive definiteness of the kernel.
