We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We study the symplectic geometry of derived intersections of Lagrangian morphisms. In particular, we show that for a functional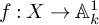 
$f : X \rightarrow \mathbb {A}_{k}^{1}$, the derived critical locus has a natural Lagrangian fibration 
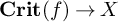$\textbf {Crit}(f) \rightarrow X$. In the case where f is nondegenerate and the strict critical locus is smooth, we show that the Lagrangian fibration on the derived critical locus is determined by the Hessian quadratic form.
