We consider a subshift of finite type on q symbols with a union of t cylinders based at words of identical length p as the hole. We explore the relationship between the escape rate into the hole and a rational function, 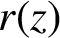$r(z)$, of correlations between forbidden words in the subshift with the hole. In particular, we prove that there exists a constant 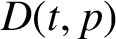$D(t,p)$ such that if 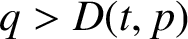$q>D(t,p)$, then the escape rate is faster into the hole when the value of the corresponding rational function 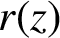$r(z)$ evaluated at $D(t,p)$ is larger. Further, we consider holes which are unions of cylinders based at words of identical length, having zero cross-correlations, and prove that the escape rate is faster into the hole with larger Poincaré recurrence time. Our results are more general than the existing ones known for maps conjugate to a full shift with a single cylinder as the hole.

We consider multimodal maps with holes and study the evolution of the open systems with respect to equilibrium states for both geometric and Hölder potentials. For small holes, we show that a large class of initial distributions share the same escape rate and converge to a unique absolutely continuous conditionally invariant measure; we also prove a variational principle connecting the escape rate to the pressure on the survivor set, with no conditions on the placement of the hole. Finally, introducing a weak condition on the centre of the hole, we prove scaling limits for the escape rate for holes centred at both periodic and non-periodic points, as the diameter of the hole goes to zero.

Although the whip is a common tool that has been used for thousands of years, there have been very few studies on its dynamic behavior. With the advance of modern technology, designing and building soft- body robot whips has become feasible. This paper presents a study on the modeling and experimental testing of a robot whip. The robot whip is modeled using a Pseudo-Rigid-Body Model (PRBM). The PRBM consists of a number of pseudo-rigid-links and pseudo-revolute-joints just like a multi-linkage pendulum. Because of its large number of degrees of freedom (DOF) and inherited underactuation, the robot whip exhibits prominent transient chaotic behavior. In particular, depending on the initial driving force, the chaos may start sooner or later, but will die down because of the gravity and air damping. The dynamic model is validated by experiments. It is interesting to note that with the same amount of force, the robot whip can generate a velocity more than 3 times and an acceleration up to 43 times faster than that of its rigid counterpart. This gives the robot whip some potential applications, such as whipping, wrapping and grabbing. This study also helps to develop other soft-body robots that involve nonlinear dynamics.