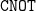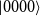Refine search
Actions for selected content:
1 results
Quantum circuits generating four-qubit maximally entangled states
-
- Journal:
- Mathematical Structures in Computer Science / Volume 32 / Issue 3 / March 2022
- Published online by Cambridge University Press:
- 28 September 2022, pp. 257-270
-
- Article
- Export citation