A bipartite graph 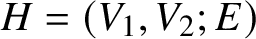$H = \left (V_1, V_2; E \right )$ with $\lvert V_1\rvert + \lvert V_2\rvert = n$ is semilinear if 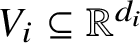$V_i \subseteq \mathbb {R}^{d_i}$ for some 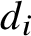$d_i$ and the edge relation E consists of the pairs of points 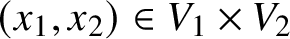$(x_1, x_2) \in V_1 \times V_2$ satisfying a fixed Boolean combination of s linear equalities and inequalities in 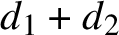$d_1 + d_2$ variables for some s. We show that for a fixed k, the number of edges in a 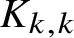$K_{k,k}$ -free semilinear H is almost linear in n, namely 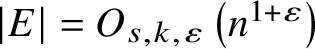$\lvert E\rvert = O_{s,k,\varepsilon }\left (n^{1+\varepsilon }\right )$ for any 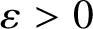$\varepsilon> 0$ ; and more generally, $\lvert E\rvert = O_{s,k,r,\varepsilon }\left (n^{r-1 + \varepsilon }\right )$ for a $K_{k, \dotsc ,k}$ -free semilinear r-partite r-uniform hypergraph.

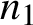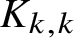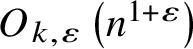As an application, we obtain the following incidence bound: given $n_1$ points and $n_2$ open boxes with axis-parallel sides in $\mathbb {R}^d$ such that their incidence graph is $K_{k,k}$ -free, there can be at most $O_{k,\varepsilon }\left (n^{1+\varepsilon }\right )$ incidences. The same bound holds if instead of boxes, one takes polytopes cut out by the translates of an arbitrary fixed finite set of half-spaces.

We also obtain matching upper and (superlinear) lower bounds in the case of dyadic boxes on the plane, and point out some connections to the model-theoretic trichotomy in o-minimal structures (showing that the failure of an almost-linear bound for some definable graph allows one to recover the field operations from that graph in a definable manner).