In this note, we compare the arrival and time stationary distributions of the number of customers in the GI/M/c/n and GI/M/c queueing systems as n tends to infinity. We show that earlier results established for GI/M/1/n and GI/M/1 remain true. Namely, it is proved that if the interarrival time c.d.f. H is non lattice with mean value λ−1 and if the traffic intensity 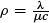 is strictly less than one, then the convergence rates in l1norm of the arrival and time stationary distributions of GI/M/c/n to the corresponding stationary distributions of GI/M/c are geometric and are characterized by ω, the unique solution in (0,1) of the equation z = ∫∞0 exp{-μc(1-z)t}dH(t).
is strictly less than one, then the convergence rates in l1norm of the arrival and time stationary distributions of GI/M/c/n to the corresponding stationary distributions of GI/M/c are geometric and are characterized by ω, the unique solution in (0,1) of the equation z = ∫∞0 exp{-μc(1-z)t}dH(t).